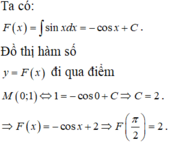Tính nguyên hàm của y=sin³x.cosx
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Tính đạo hàm của hàm số y = x.cosx
A. cosx – x.sinx
B. sinx + x.cosx
C. cosx+ x. sinx
D. cosx + sinx

Chọn A
Ta áp dụng đạo hàm của 1 tích :
y ' = ( x ) ' . c osx + x. (cosx)' =1.cosx + x. (- sinx ) = c osx- x.sin x

vì: sin bình phương x= 1-cos bình phương x
vậy y = (1-cos bình phương x) nhân cos c
=cos x nhân cos mũ ba x
đặt a= cos x (đk : -1<= a<= 1)
khi đó y =a-a^3
đạo hàm = -3a^2=1
theo bản biến thiên thì trên khoảng -1 đến 1 thì giá trị y đạt dược lớn nhất tại a=căn ba trên 3
a=căn 3 trên ba => cos x=căn ba trên ba => x=........

a: TXĐ: D=R
Với mọi x thuộc D thì -x cũng thuộc D
\(f\left(-x\right)=-x\cdot cos\left(-x\right)=-x\cdot cosx=-f\left(x\right)\)
=>f(x) lẻ
b: TXĐ: D=R
Với mọi x thuộc D thì -x cũng thuộc D
\(f\left(-x\right)=5\cdot sin^2\left(-x\right)+1=5\cdot sin^2x+1=f\left(x\right)\)
=>f(x) chẵn
c: TXĐ: D=R
Với mọi x thuộc D thì -x cũng thuộc D
\(f\left(-x\right)=sin\left(-x\right)\cdot cos\left(-x\right)=-sinx\cdot cosx=-f\left(x\right)\)
=>f(x) lẻ

Đáp án B
Do y = x cos x nên y ' = cos x − x sin x ⇒ y ' ' = − sin x − sin x − x cos x = − 2 sin x − x cos x
Như thế 2 cos x − y ' = 2 x sin x , x y ' ' + y = − 2 x sin x
Vậy 2 cos x − y ' + x y ' ' + y = 0

tham khảo:
a)\(y'=xsin2x+sin^2x\)
\(y'=sin^2x+xsin2x\)
b)\(y'=-2sin2x+2cosx\\ y'=2\left(cosx-sin2x\right)\)
c)\(y=sin3x-3sinx\)
\(y'=3cos3x-3cosx\)
d)\(y'=\dfrac{1}{cos^2x}-\dfrac{1}{sin^2x}\)
\(y'=\dfrac{sin^2x-cos^2x}{sin^2x.cos^2x}\)


Cách này hơi dài chút, nhưng nếu nghĩ ra cách hay hơn mình sẽ đề xuất nhe!
\(=\int\sin^5x.\left(2\sin x\cos x\right)^3.2xdx=16\int x.\sin^8x\cos^3xdx\)
\(\left\{{}\begin{matrix}u=x\\dv=\sin^8x.\cos^3xdx\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}du=dx\\v=\int\sin^8x.\cos^3xdx\end{matrix}\right.\)
\(I_1=\int\sin^8x\cos^3xdx=\int\sin^8x.\cos^2x.\cos xdx=\int\sin^8x.\left(1-\sin^2x\right)\cos xdx\)
\(t=\sin x\Rightarrow dt=\cos xdx\Rightarrow\int\sin^8x\left(1-\sin^2x\right)\cos xdx=\int(t^8-t^{10})dt=\dfrac{1}{9}t^9-\dfrac{1}{11}t^{11}=\dfrac{1}{9}\sin^9x-\dfrac{1}{11}\sin^{11}x\)
\(\Rightarrow\left\{{}\begin{matrix}du=dx\\v=\dfrac{1}{9}\sin^9x-\dfrac{1}{11}\sin^{11}x\end{matrix}\right.\)
\(\Rightarrow\dfrac{I}{16}=x.\left(\dfrac{1}{9}\sin^9x-11\sin^{11}x\right)-\int\left(\dfrac{1}{9}\sin^9x-\dfrac{1}{11}\sin^{11}x\right)dx\)
\(I_2=\int\left(\dfrac{1}{9}\sin^9x-\dfrac{1}{11}\sin^{11}x\right)dx=\dfrac{1}{9}\int\sin^9xdx-\dfrac{1}{11}\int\sin^{11}xdx\)
À thế này là xong rồi còn gì :) Bạn tự làm nốt nhé