Tìm GTLN,GTNN của hàm số : \(y=2sin^2x+cos^22x\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đề là \(y=2sin^2x+cos^22x\) hả bạn? Và tìm GTNN, GTLN hay tìm tập giá trị?

\(y=1-cos2x+2sin2x+6=2sin2x-cos2x+7\)
\(y=\sqrt{5}\left(\dfrac{2}{\sqrt{5}}sin2x-\dfrac{1}{\sqrt{5}}cos2x\right)+7\)
Đặt \(\dfrac{2}{\sqrt{5}}=cosa\) với \(a\in\left(0;\dfrac{\pi}{2}\right)\)
\(y=\sqrt{5}sin\left(2x-a\right)+7\)
\(\Rightarrow-\sqrt{5}+7\le y\le\sqrt{5}+7\)

y=(sin2x-3)^2-6
-1<=sin2x<=1
=>-4<=sin2x-3<=-2
=>4<=(sin2x-3)^2<=16
=>-2<=y<=10
y min khi sin2x-3=-2
=>sin 2x=1
=>2x=pi/2+k2pi
=>x=pi/4+kpi
y max khi sin 2x-3=-4
=>sin 2x=-1
=>2x=-pi/2+k2pi
=>x=-pi/4+kpi

ta có \(y=2sin^4x+\left(1-2sin^2x\right)^2\)=\(2sin^4x+4sin^4x-4sinx^2+1=6sin^4x-4sin^2x+1\)
đặt \(t=sin^2x,0\le t\le1\)
ta đc \(y=6t^2-4t+1\)
ta tính y'=12t-4
giải pt y'=0 suy ra t=1/3
ta có bảng biến thiên
x y' y 0 1/3 1 0 - + 1 3 1/3
từ bảng bt ta suy ra hàm số đạt giá trị nhỏ nhất \(y=\frac{1}{3}\) khi \(t=\frac{1}{3}\Rightarrow sin^2x=\frac{1}{3}\)
hàm số đạt giá trị lớn nhất y=3 khi \(t=1\Rightarrow sin^2x=1\)

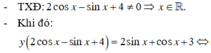
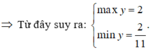
\(y=2\left(\frac{1}{2}-\frac{1}{2}cos2x\right)+cos^22x=cos^22x-cos2x+1\)
\(=\left(cos2x-\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}\)
\(\Rightarrow y_{min}=\frac{3}{4}\) khi \(cos2x=\frac{1}{2}\)
\(y=cos^22x-2cos2x+cos2x-2+3\)
\(y=\left(cos2x-2\right)\left(cos2x+1\right)+3\)
Do \(-1\le cos2x\le1\Rightarrow\left\{{}\begin{matrix}cos2x-2< 0\\cos2x+1\ge0\end{matrix}\right.\) \(\Rightarrow\left(cos2x-2\right)\left(cos2x+1\right)\le0\)
\(\Rightarrow y\le3\Rightarrow y_{max}=3\) khi \(cos2x=-1\)