Lg2x - logx.log2(4x)+2log2x=0 . Tổng nghiệm là bao nhiêu
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Những câu hỏi liên quan
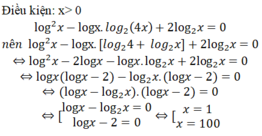

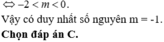
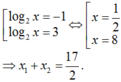


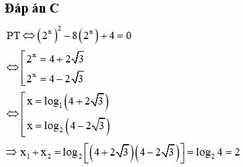
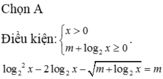
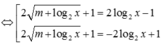
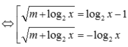
Đề như vậy hả bạn?
\(log^2x-logx.log_2\left(4x\right)+2log_2x=0\)
\(\Leftrightarrow log^2x-logx.\left(log_24+log_2x\right)+2log_2x=0\)
\(\Leftrightarrow log^2x-logx.\left(2+log_2x\right)+2log_2x=0\)
\(\Leftrightarrow log^2x-2logx-logx.log_2x+2log_2x=0\)
\(\Leftrightarrow logx\left(logx-2\right)-log_2x\left(logx-2\right)=0\)
\(\Leftrightarrow\left(logx-2\right)\left(logx-log_2x\right)=0\) \(\Rightarrow\left[{}\begin{matrix}logx=2\Rightarrow x=100\\logx-log_2x=0\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow logx-\dfrac{logx}{log2}=0\Rightarrow logx\left(1-\dfrac{1}{log2}\right)=0\Rightarrow logx=0\Rightarrow x=1\)
Vậy tổng các nghiệm là \(100+1=101\)