cho 3 số x,y,z không âm thỏa mãn x3+y3+z3=3. Tìm GTLN của A=3(xy+yz+zx)-xyz
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
SD
1
CV
24 tháng 10 2018
ap dung bdt co si ta co:
\(xy+yz+xz>=3\sqrt[3]{\left(xyz\right)^2}\)
=>\(100>3\sqrt[3]{x^2y^2z^2}\)
=>\(\frac{100}{3}>=\sqrt[3]{\left(xyz\right)^2}\)
=>\(\sqrt{\frac{100^3}{3^3}}>=xyz\)
=>\(\frac{1000}{3\sqrt{3}}>=xyz\)
=>\(Amax=\frac{1000}{3\sqrt{3}}\)
xay ra dau bang khi va chi khi x=y=z\(\frac{10}{\sqrt{3}}\)
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 8 2021
\(P=\sqrt{y}\left(\sqrt{x}+2\sqrt{z}\right)+3\sqrt{zx}=\left(6-\sqrt{x}-\sqrt{z}\right)\left(\sqrt{x}+2\sqrt{z}\right)+3\sqrt{zx}\)
\(P=-x+6\sqrt{x}-2z+12z=-\left(\sqrt{x}-3\right)^2-2\left(\sqrt{z}-3\right)^2+27\le27\)
\(P_{max}=27\) khi \(\left(x;y;z\right)=\left(9;0;9\right)\)
DT
0
PT
1
23 tháng 11 2016
với mọi x, y, z ta có:
(x-y)^2 +(y-z)^2+ (z-x)^2>=0
<=>2x^2 +2y^2 + 2z^2 - 2xy -2yz - 2xz >=0
<=>x^2 + y^2 +z^2 - xy -yz -zx >=0
<=>(x+y+z)^2 >= 3(x+y+z)
<=>[(x+y+z)^2]/3 >= xy+yz+ zx
=>xy +yz + zx <=3
dấu = xảy ra khi x=y=z =1
Khi đó P=1.1+1.1+1.1=3
CM
12 tháng 10 2017
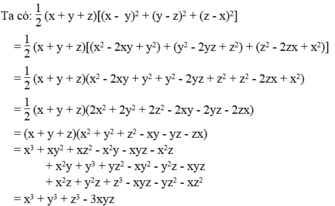
Vế trái bằng vế phải nên đẳng thức được chứng minh.
Nếu x ≥ 0, y ≥ 0, z ≥ 0 thì:
x + y + z ≥ 0
x - y 2 + y - z 2 + z - x 2 ≥ 0
Suy ra:
x 3 + y 3 + z 3 - 3 x y z ≥ 0 ⇔ x 3 + y 3 + z 3 ≥ 3 x y z
Hay: x 3 + y 3 + z 3 3 ≥ x y z
7 tháng 10 2021
\(P=\dfrac{\sqrt{x}}{\sqrt{xy}+\sqrt{x}+3}+\dfrac{\sqrt{y}}{\sqrt{yz}+\sqrt{y}+1}+\dfrac{3\sqrt{z}}{\sqrt{zx}+3\sqrt{x}+3}\)
\(=\dfrac{\sqrt{x}}{\sqrt{xy}+\sqrt{x}+\sqrt{xyz}}+\dfrac{\sqrt{y}}{\sqrt{yz}+\sqrt{y}+1}+\dfrac{\sqrt{xyz}\sqrt{z}}{\sqrt{zx}+\sqrt{xyz}\sqrt{z}+\sqrt{xyz}}\)
\(=\dfrac{1}{\sqrt{y}+1+\sqrt{yz}}+\dfrac{\sqrt{y}}{\sqrt{yz}+\sqrt{y}+1}+\dfrac{\sqrt{yz}}{1+\sqrt{yz}+\sqrt{y}}\)
\(=\dfrac{1+\sqrt{y}+\sqrt{yz}}{1+\sqrt{y}+\sqrt{yz}}=1\)
\(\Rightarrow\sqrt{10P-1}=\sqrt{10.1-1}=\sqrt{9}=3\)
TP
1
29 tháng 7 2020
Bài làm:
Ta có: \(x+y+z=8\Leftrightarrow\left(x+y+z\right)^2=64\)
\(\Leftrightarrow x^2+y^2+z^2+2\left(xy+yz+zx\right)=64\)
Mà \(\hept{\begin{cases}x^2+y^2\ge2xy\\y^2+z^2\ge2yz\\z^2+x^2\ge2zx\end{cases}}\)\(\Rightarrow2\left(x^2+y^2+z^2\right)\ge2\left(xy+yz+zx\right)\)
\(\Rightarrow x^2+y^2+z^2\ge xy+yz+zx\)
Thay vào ta có: \(64\ge3\left(xy+yz+zx\right)\)
\(\Leftrightarrow xy+yz+zx\le\frac{64}{3}\)
Dấu "=" xảy ra khi: \(x=y=z=\frac{8}{3}\)
Vậy Max(B) = 64/3 khi x = y = z = 8/3