giúp mik vs ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




e: \(E=\dfrac{x^2-9-x^2+4-x^2+9}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{x+2}{x+3}\)
a: \(A=\dfrac{4x^2+x^2-2x+1+x^2+2x+1}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{6x^2+2}{\left(x-1\right)\left(x+1\right)}\)


27. unforgetable
câu 31 đổi thành ...if I can give him his guide....
câu 32 thiếu on trong turned on và đổi thành has been turned on vì câu gốc là HTHT
câu 33 rút gọn mệnh đề ok
câu 34 hơi phân vân nhưng mình thấy đúng

Câu 3:
a: Áp dụng tính chất của dãy tỉ số bằng nhau, ta được:
\(\dfrac{x}{3}=\dfrac{y}{2}=\dfrac{x+y}{3+2}=\dfrac{90}{5}=18\)
Do đó: x=54; y=36

c. \(\left|\dfrac{8}{4}-\left|x-\dfrac{1}{4}\right|\right|-\dfrac{1}{2}=\dfrac{3}{4}\)
\(\Rightarrow\left[{}\begin{matrix}\left|\dfrac{8}{4}-x+\dfrac{1}{4}\right|-\dfrac{1}{2}=\dfrac{3}{4}\\\left|\dfrac{8}{4}+x-\dfrac{1}{4}\right|-\dfrac{1}{2}=\dfrac{3}{4}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}\left|\dfrac{9}{4}-x\right|-\dfrac{1}{2}=\dfrac{3}{4}\\\left|\dfrac{7}{4}+x\right|-\dfrac{1}{2}=\dfrac{3}{4}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\left[{}\begin{matrix}\dfrac{9}{4}-x-\dfrac{1}{2}=\dfrac{3}{4}\\x=\dfrac{9}{4}-\dfrac{1}{2}=\dfrac{3}{4}\end{matrix}\right.\\\left[{}\begin{matrix}\dfrac{7}{4}+x-\dfrac{1}{2}=\dfrac{3}{4}\\-\dfrac{7}{4}-x-\dfrac{1}{2}=\dfrac{3}{4}\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}\left[{}\begin{matrix}x=1\\x=\dfrac{7}{2}\end{matrix}\right.\\\left[{}\begin{matrix}x=-\dfrac{1}{2}\\x=-3\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{7}{2}\\x=-3\end{matrix}\right.\)
Ở nơi x=9/4-1/2 là x-9/4-1/2 nha
a. -1,5 + 2x = 2,5
<=> 2x = 2,5 + 1,5
<=> 2x = 4
<=> x = 2
b. \(\dfrac{3}{2}\left(x+5\right)-\dfrac{1}{2}=\dfrac{4}{3}\)
<=> \(\dfrac{3}{2}x+\dfrac{15}{2}-\dfrac{1}{2}=\dfrac{4}{3}\)
<=> \(\dfrac{9x}{6}+\dfrac{45}{6}-\dfrac{3}{6}=\dfrac{8}{6}\)
<=> 9x + 45 - 3 = 8
<=> 9x = 8 + 3 - 45
<=> 9x = -34
<=> x = \(\dfrac{-34}{9}\)

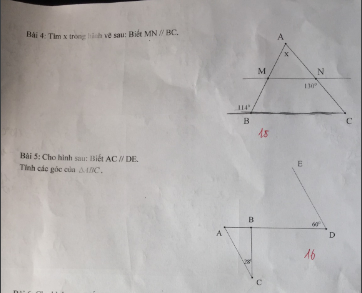 giúp mik với ạ mik cần gấp. GIẢI CỤ THỂ GIÚP MIK vs ạ
giúp mik với ạ mik cần gấp. GIẢI CỤ THỂ GIÚP MIK vs ạ

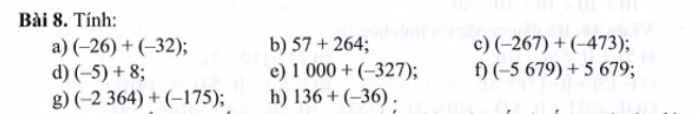






Trả lời:
Bài 1:
a, \(4x^2-10x+7\)
\(=4x^2-10x+\frac{25}{2}+\frac{3}{4}\)
\(=\left(4x^2-10x+\frac{25}{2}\right)+\frac{3}{4}\)
\(=\left[\left(2x\right)^2-2.2x.\frac{5}{2}+\left(\frac{5}{2}\right)^2\right]+\frac{3}{4}\)
\(=\left(2x-\frac{5}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}>0\forall x\)
Vậy đpcm
b, \(2x-4x^2-1\)
\(=-\left(4x^2-2x+1\right)\)
\(=-\left[4x^2-2x+\frac{1}{4}+\frac{3}{4}\right]\)
\(=-\left[\left(2x\right)^2-2.2x.\frac{1}{2}+\left(\frac{1}{2}\right)^2+\frac{3}{4}\right]\)
\(=-\left[\left(2x-\frac{1}{2}\right)^2+\frac{3}{4}\right]\)
\(=-\left(2x-\frac{1}{2}\right)^2-\frac{3}{4}\le-\frac{3}{4}< 0\forall x\)
Vậy đpcm
c, \(4x^2+6x+13\)
\(=4x^2+6x+\frac{9}{4}+\frac{43}{4}\)
\(=\left(4x^2+6x+\frac{9}{4}\right)+\frac{43}{4}\)
\(=\left[\left(2x\right)^2+2.2x.\frac{3}{2}+\left(\frac{3}{2}\right)^2\right]+\frac{43}{4}\)
\(=\left(2x+\frac{3}{2}\right)^2+\frac{43}{4}\ge\frac{43}{4}\forall x\)
Dấu "=" xảy ra khi \(2x+\frac{3}{2}=0\Leftrightarrow x=-\frac{3}{4}\)
Vậy GTNN của bt bằng 43/4 khi x = - 3/4
d, \(-8x-x^2+3\)
\(=-\left(x^2+8x-3\right)\)
\(=-\left(x^2+8x+16-19\right)\)
\(=-\left[\left(x+4\right)^2-19\right]\)
\(=-\left(x+4\right)^2+19\le19\forall x\)
Dấu "=" xảy ra khi x + 4 = 0 <=> x = - 4
Vậy GTLN của bt bằng 19 khi x = - 4
Trả lời:
Bài 2:
a, \(3x^3-18x^2+27x=0\)
\(\Leftrightarrow3x\left(x^2-6x+9\right)=0\)
\(\Leftrightarrow3x\left(x-3\right)^2=0\)
\(\Leftrightarrow\orbr{\begin{cases}3x=0\\\left(x-3\right)^2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=3\end{cases}}}\)
Vậy x = 0; x = 3 là nghiệm của pt.
b, \(6x\left(x-3\right)=x^3-9x\)
\(\Leftrightarrow6x\left(x-3\right)-\left(x^3-9x\right)=0\)
\(\Leftrightarrow6x\left(x-3\right)-x\left(x^2-9\right)=0\)
\(\Leftrightarrow6x\left(x-3\right)-x\left(x-3\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left[6x-x\left(x+3\right)\right]=0\)
\(\Leftrightarrow\left(x-3\right)\left(6x-x^2-3x\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(-x^2+3x\right)=0\)
\(\Leftrightarrow x\left(x-3\right)\left(3-x\right)=0\)
\(\Leftrightarrow-x\left(x-3\right)\left(x-3\right)=0\)
\(\Leftrightarrow-x\left(x-3\right)^2=0\)
\(\Leftrightarrow\orbr{\begin{cases}-x=0\\\left(x-3\right)^2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=3\end{cases}}}\)
Vậy x = 0; x = 3 là nghiệm của pt.
c, \(4x^3-6x^2+2x=0\)
\(\Leftrightarrow4x\left(x^2-\frac{3}{2}x+\frac{1}{2}\right)=0\)
\(\Leftrightarrow4x\left(x^2-2.x.\frac{3}{4}+\frac{9}{16}-\frac{1}{16}\right)=0\)
\(\Leftrightarrow4x\left[\left(x-\frac{3}{4}\right)^2-\frac{1}{16}\right]=0\)
\(\Leftrightarrow\orbr{\begin{cases}4x=0\\\left(x-\frac{3}{4}\right)^2-\frac{1}{16}=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\\left(x-\frac{3}{4}\right)^2=\frac{1}{16}\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=0\\x=1;x=\frac{1}{2}\end{cases}}}\)
Vậy x = 0; x = 1; x = 1/2 là nghiệm của pt.