Giải phương trình:x3+\(\dfrac{\text{x^3}}{\text{ }\left(x-1\right)}\)+\(\dfrac{\text{3x^2}}{\text{x-1}}\)=2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


ĐKXĐ: ...
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3}{x+y}+6x-3y=6\\\dfrac{3}{x+y}+2x-4y=1\end{matrix}\right.\)
\(\Rightarrow4x+y=5\Rightarrow y=5-4x\)
Thế vào phương trình đầu:
\(\dfrac{1}{x+5-4x}+2x-\left(5-4x\right)=2\)
\(\Leftrightarrow\dfrac{1}{5-3x}+6x-7=0\)
\(\Leftrightarrow\left(6x-7\right)\left(5-3x\right)+1=0\)
\(\Leftrightarrow...\)

a: \(\left\{{}\begin{matrix}3x-2y=1\\2x+4y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}6x-4y=2\\2x+4y=3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}8x=5\\3x-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{5}{8}\\2y=3x-1=\dfrac{15}{8}-1=\dfrac{7}{8}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{5}{8}\\y=\dfrac{7}{16}\end{matrix}\right.\)
b: \(\left\{{}\begin{matrix}4x-3y=1\\-x+2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x-3y=1\\-4x+8y=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=1\\x=-1+2y=-1+2=1\end{matrix}\right.\)
c: \(\left\{{}\begin{matrix}\dfrac{2}{3}x+\dfrac{4}{3}y=1\\\dfrac{1}{2}x-\dfrac{3}{4}y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x+4y=3\\2x-3y=8\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{41}{14}\\y=-\dfrac{5}{7}\end{matrix}\right.\)

câu c) mang tính mua vui hay gì hả bn
mếu thật thì x=0,x=số nào cx đc(câu trả lời này mang tính mua vui thôi nhé)

\(\dfrac{x-2}{x+1}-\dfrac{3}{x+2}>0.\left(x\ne-1;-2\right).\\ \Leftrightarrow\dfrac{x^2-4-3x-3}{\left(x+1\right)\left(x+2\right)}>0.\\ \Leftrightarrow\dfrac{x^2-3x-7}{\left(x+1\right)\left(x+2\right)}>0.\)
Đặt \(f\left(x\right)=\dfrac{x^2-3x-7}{\left(x+1\right)\left(x+2\right)}>0.\)
Ta có: \(x^2-3x-7=0.\Rightarrow\left[{}\begin{matrix}x=\dfrac{3+\sqrt{37}}{2}.\\x=\dfrac{3-\sqrt{37}}{2}.\end{matrix}\right.\)
\(x+1=0.\Leftrightarrow x=-1.\\ x+2=0.\Leftrightarrow x=-2.\)
Bảng xét dấu:
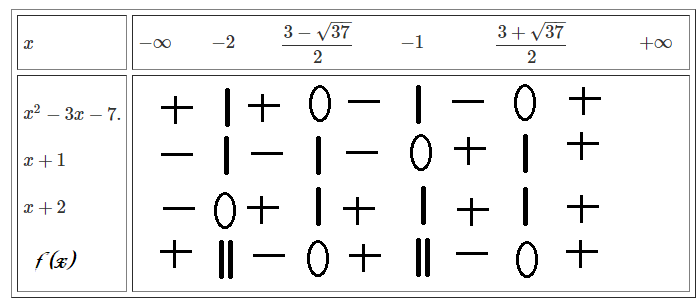
\(\Rightarrow f\left(x\right)>0\Leftrightarrow x\in\left(-\infty-2\right)\cup\left(\dfrac{3-\sqrt{37}}{2};-1\right)\cup\left(\dfrac{3+\sqrt{37}}{2};+\infty\right).\)
\(\sqrt{x^2-3x+2}\ge3.\\ \Leftrightarrow x^2-3x+2\ge9.\\ \Leftrightarrow x^2-3x-7\ge0.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{3-\sqrt{37}}{2}.\\x=\dfrac{3+\sqrt{37}}{2}.\end{matrix}\right.\)
Đặt \(f\left(x\right)=x^2-3x-7.\)
\(f\left(x\right)=x^2-3x-7.\)
\(\Rightarrow f\left(x\right)\ge0\Leftrightarrow x\in(-\infty;\dfrac{3-\sqrt{37}}{2}]\cup[\dfrac{3+\sqrt{37}}{2};+\infty).\)
\(\Rightarrow\sqrt{x^2-3x+2}\ge3\Leftrightarrow x\in(-\infty;\dfrac{3-\sqrt{37}}{2}]\cup[\dfrac{3+\sqrt{37}}{2};+\infty).\)

b) (4√x + 4)/(x + 2√x + 5) ≥ 1
⇔ (4√x + 4)/(x + 2√x + 5) - 1 ≤ 0
Do x ≥ 0 ⇒ x + 2√x + 5 > 0
⇒ (4√x + 4)/(x + 2√x + 5) - 1 ≤ 0
⇔ (4√x + 4) - (x + 2√x + 5) ≤ 0
⇔ 4√x + 4 - x - 2√x - 5 ≤ 0
⇔ -x + 2√x - 1 ≤ 0
⇔ -(x - 2√x + 1) ≤ 0
⇔ -(√x - 1)² ≤ 0 (luôn đúng)
Vậy (4√x + 4)/(x + 2√x + 5) ≤ 1 với mọi x ≥ 0
a: \(P=\dfrac{x+8\sqrt{x}+8-x-4\sqrt{x}-4}{\sqrt{x}\left(\sqrt{x}+2\right)}:\dfrac{x+\sqrt{x}+3+\sqrt{x}+2}{\sqrt{x}\left(\sqrt{x}+2\right)}\)
\(=\dfrac{4\left(\sqrt{x}+1\right)}{x+2\sqrt{x}+5}\)
b: 4(căn x+1)>=4
x+2căn x+5>=5
=>P<=4/5<1

\(\dfrac{-x^2-3x+18}{\left(x-2\right)\left(x+2\right)}< 0\)
\(\Leftrightarrow\dfrac{x^2+3x-18}{\left(x-2\right)\left(x+2\right)}>0\)

Theo BXD, ta có: f(x)>0
=>\(x\in\left(-\infty;-6\right)\cup\left(-2;2\right)\cup\left(3;+\infty\right)\)