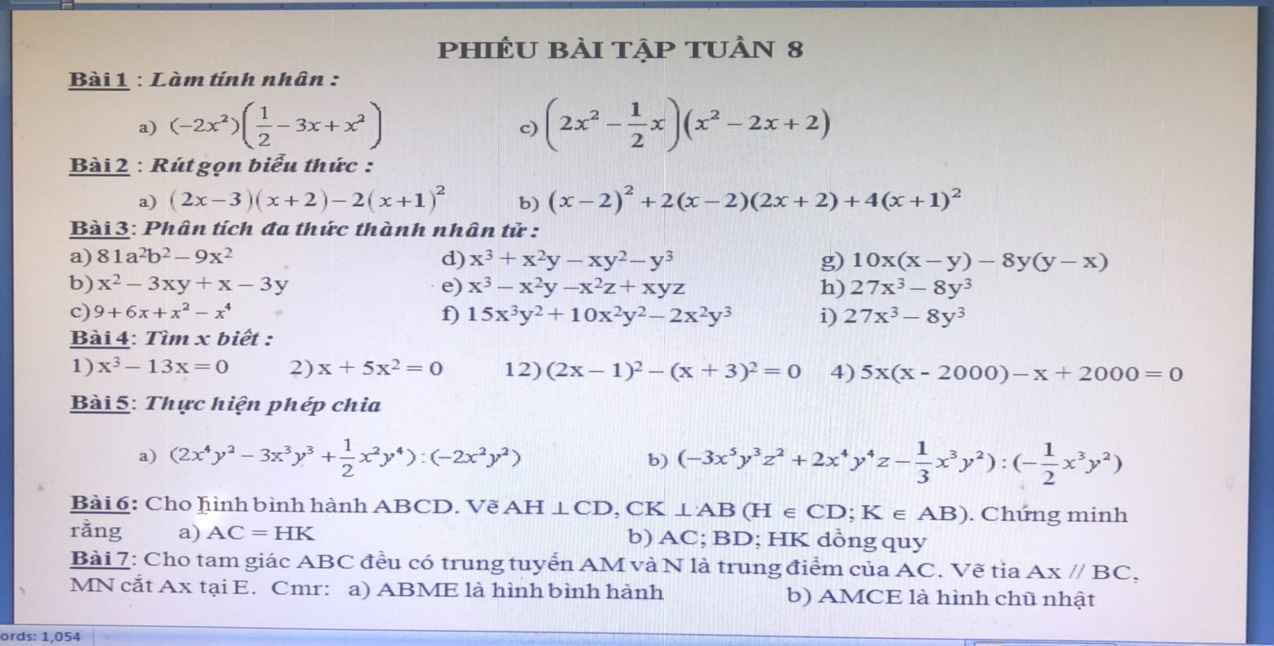
Làm hộ mình 3 bài cr ơn ạ:)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



1) Vì x=25 thỏa mãn ĐKXĐ nên Thay x=25 vào biểu thức \(A=\dfrac{\sqrt{x}-2}{x+1}\), ta được:
\(A=\dfrac{\sqrt{25}-2}{25+1}=\dfrac{5-2}{25+1}=\dfrac{3}{26}\)
Vậy: Khi x=25 thì \(A=\dfrac{3}{26}\)
2) Ta có: \(B=\dfrac{\sqrt{x}-3}{\sqrt{x}+1}+\dfrac{2x+8\sqrt{x}-6}{x-\sqrt{x}-2}\)
\(=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}+\dfrac{2x+8\sqrt{x}-6}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x-5\sqrt{x}+6+2x+8\sqrt{x}-6}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3x+3\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3\sqrt{x}}{\sqrt{x}-2}\)

a: Tỉ số là 3/2
b: Tỉ số phần trăm là;
40/(30+40+20+20+5)=34,78%
ngày mai mình thi học kì, đây là bài luyện tập, các bạn làm hộ để mình check bài với ạ. cảm ơn nhiều 

\(a,ĐK:x\ne0;x\ne5\\ B=\dfrac{x^2-25+2x^2-12x-x^2+8x+25}{2x\left(x-5\right)}=\dfrac{2x\left(x-2\right)}{2x\left(x-5\right)}=\dfrac{x-2}{x-5}\\ b,x=3\Leftrightarrow A=\dfrac{3+6}{5-3}=\dfrac{9}{2}\\ c,\text{Câu a}\\ d,E=B-A=\dfrac{x-2}{x-5}+\dfrac{x+6}{x-5}=\dfrac{2x+4}{x-5}=\dfrac{2\left(x-5\right)+14}{x-5}=2+\dfrac{14}{x-5}\in Z\\ \Leftrightarrow x-5\inƯ\left(14\right)=\left\{-14;-7;-2;-1;1;2;7;14\right\}\\ \Leftrightarrow x\in\left\{-9;-2;3;4;6;7;12;19\right\}\)


a) Phương trình hoành độ giao điểm là:
\(x^2=\left(m+2\right)x-2m\)
\(\Leftrightarrow x^2-\left(m+2\right)x+2m=0\)
\(\Delta=\left(m+2\right)^2-8m=m^2+4m+4-8m=m^2-4m+4=\left(m-2\right)^2\)
Để (d) và (P) cắt nhau tại hai điểm phân biệt thì \(\Delta>0\)
\(\Leftrightarrow\left(m-2\right)^2>0\)
mà \(\left(m-2\right)^2\ge0\)
nên \(m-2\ne0\)
hay \(m\ne2\)
Vậy: Để (d) và (P) cắt nhau tại hai điểm phân biệt thì \(m\ne2\)

\(2,\\ a,=2x^2+4x-3x-6-2x^2-4x-2=-3x-8\\ b,=\left[x-2+2\left(x+1\right)\right]^2=\left(x-2+2x+2\right)^2=9x^2\)




 làm bài hộ mình voiwas ạ, mik cảm ơn trước! ngày sinh là 20/2/2008
làm bài hộ mình voiwas ạ, mik cảm ơn trước! ngày sinh là 20/2/2008