cho biểu thức ;
M=5^0+5^1+5^2+5^3+5^4+5^5+...+5^2009
Chứng tỏ rằng;(4M+1).2^2010 là số chính phương
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
CM
13 tháng 8 2019
Thay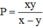 
vào biểu thức ta được:
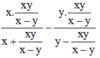
Ta có:
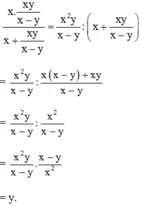
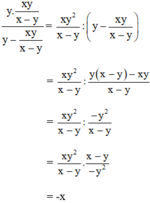
Vậy giá trị biểu thức bằng y – (-x) = x + y.
CM
21 tháng 7 2019
Ta có: 375+28=28+375
Vậy biểu thức có giá trị bằng với biểu thức 375+28 là 28+375.
Đáp án B
CM
30 tháng 7 2018
Khi nhân một số với một tổng, ta có thể nhân số đó với từng số hạng của tổng, rồi cộng các kết quả với nhau.
Do đó ta có: 45×(5+4)=45×5+45×4.
Vậy biểu thức có kết quả bằng với biểu thức 45×(5+4) là 45×5+45×4
Đáp án D
CM
6 tháng 12 2018
Khi đổi chỗ các thừa số trong một tích thì tích đó không thay đổi.
Do đó ta có: 38756×9=9×38756
Vậy biểu thức có giá trị bằng với biểu thức 38756×9 là 9×38756.
Đáp án D
.
18 tháng 1 2022
a,ĐKXĐ:\(\left\{{}\begin{matrix}x-3\ne0\\x+3\ne0\\x^2-9\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne3\\x\ne-3\\x\ne\pm3\end{matrix}\right.\Leftrightarrow x\ne\pm3\)
b, \(M=\dfrac{x+3}{x-3}-\dfrac{x-3}{x+3}-\dfrac{36}{x^2-9}\)
\(\Rightarrow M=\dfrac{\left(x+3\right)^2-\left(x-3\right)^2-36}{\left(x-3\right)\left(x+3\right)}\)
\(\Rightarrow M=\dfrac{x^2+6x+9-x^2+6x-9-36}{\left(x-3\right)\left(x+3\right)}\)
\(\Rightarrow M=\dfrac{12x-36}{\left(x-3\right)\left(x+3\right)}\)
\(\Rightarrow M=\dfrac{12\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}\)
\(\Rightarrow M=\dfrac{12}{x+3}\)
c, Thay x=0 vào M ta có: \(M=\dfrac{12}{x+3}=\dfrac{12}{0+3}=\dfrac{12}{3}=4\)
18 tháng 1 2022
a: ĐKXĐ: \(x\notin\left\{3;-3\right\}\)
b: \(M=\dfrac{x^2+6x+9-x^2+6x-9-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12x-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12}{x+3}\)
c: Thay x=0 vào M, ta được:
\(M=\dfrac{12}{0+3}=\dfrac{12}{3}=4\)
18 tháng 1 2022
a: ĐKXĐ: \(x\notin\left\{3;-3\right\}\)
b: \(M=\dfrac{x^2+6x+9-x^2+6x-9-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12x-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12}{x+3}\)
c: Thay x=0 vào M, ta được: M=12/3=4
18 tháng 1 2022
a: ĐKXĐ: \(x\notin\left\{3;-3\right\}\)
b: \(M=\dfrac{x^2+6x+9-x^2+6x-9-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12x-36}{\left(x-3\right)\left(x+3\right)}=\dfrac{12}{x+3}\)
c: Thay x=0 vào M, ta được:
M=12/3=4
22 tháng 1
a: Để \(\dfrac{3x-2}{4}\) không nhỏ hơn \(\dfrac{3x+3}{6}\) thì \(\dfrac{3x-2}{4}>=\dfrac{3x+3}{6}\)
=>\(\dfrac{6\left(3x-2\right)}{24}>=\dfrac{4\left(3x+3\right)}{24}\)
=>18x-12>=12x+12
=>6x>=24
=>x>=4
b: Để \(\left(x+1\right)^2\) nhỏ hơn \(\left(x-1\right)^2\) thì \(\left(x+1\right)^2< \left(x-1\right)^2\)
=>\(x^2+2x+1< x^2-2x+1\)
=>4x<0
=>x<0
c: Để \(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}\) không lớn hơn \(\dfrac{x^2}{7}-\dfrac{2x-3}{5}\) thì
\(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}< =\dfrac{x^2}{7}-\dfrac{2x-3}{5}\)
=>\(\dfrac{2x-3+5x\left(x-2\right)}{35}< =\dfrac{5x^2-7\cdot\left(2x-3\right)}{35}\)
=>\(2x-3+5x^2-10x< =5x^2-14x+21\)
=>-8x-3<=-14x+21
=>6x<=24
=>x<=4
6 tháng 5 2021
>_ là lớn hơn hoặc bằng nha do bị lỗi chính tả
_< là bé hơn hoặc bằng
A,
2-5x >_ 3(2-x)
⇔ 2-5x >_ 6-3x
⇔ -5x+3x >_ 6-2
⇔ -2x >_ 3
⇔ x _< \(\dfrac{-3}{2}\)
Tập nghiệm { x / x _< \(\dfrac{-3}{2}\)}
B,
-4x + 3 _< 5x - 7
⇔ -4x - 5x _< -7 - 3
⇔ -9x _< -10
⇔ x >_ \(\dfrac{10}{9}\)
Tập nghiệm { x / x >_ \(\dfrac{10}{9}\) }