
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) \(N=-1-x-x^2=-\left(x^2+x+\dfrac{1}{4}\right)-\dfrac{3}{4}=-\left(x+\dfrac{1}{2}\right)^2-\dfrac{3}{4}\le-\dfrac{3}{4}\)
\(maxN=-\dfrac{3}{4}\Leftrightarrow x=-\dfrac{1}{2}\)
b) \(B=3x^2+4x-13=3\left(x^2+\dfrac{4}{3}x+\dfrac{4}{9}\right)-\dfrac{35}{3}=3\left(x+\dfrac{2}{3}\right)^2-\dfrac{35}{3}\ge-\dfrac{35}{3}\)
\(minB=-\dfrac{35}{3}\Leftrightarrow x=-\dfrac{2}{3}\)
a: Ta có: \(N=-x^2-x-1\)
\(=-\left(x^2+x+1\right)\)
\(=-\left(x^2+2\cdot x\cdot\dfrac{1}{2}+\dfrac{1}{4}+\dfrac{3}{4}\right)\)
\(=-\left(x+\dfrac{1}{2}\right)^2-\dfrac{3}{4}\le-\dfrac{3}{4}\forall x\)
Dấu '=' xảy ra khi \(x=-\dfrac{1}{2}\)
b: ta có: \(B=3x^2+4x-13\)
\(=3\left(x^2+\dfrac{4}{3}x-\dfrac{13}{3}\right)\)
\(=3\left(x^2+2\cdot x\cdot\dfrac{2}{3}+\dfrac{4}{9}-\dfrac{43}{9}\right)\)
\(=3\left(x+\dfrac{2}{3}\right)^2-\dfrac{43}{3}\ge-\dfrac{43}{3}\forall x\)
Dấu '=' xảy ra khi \(x=-\dfrac{2}{3}\)


em ko biết em chỉ tim thui
có ai làm cho cisuuuuuuuuuuuuuuuuu ko

A = -4 - x2 + 6x = -(x2 - 6x + 9) + 5 = -(x - 3)2 + 5 \(\le\)5 \(\forall\) x
Dấu "=" xảy ra <=> x - 3 = 0 <=> x = 3
Vậy MaxA = 5 khi x = 3
F = (x - 1)(x - 3) + 11 = x2 - 4x + 3 + 11 = (x2 - 4x + 4) + 10 = (x - 2)2 + 10 \(\ge\)10 \(\forall\)x
Dấu "=" xảy ra <=> x - 2 = 0 <=> x = 2
Vậy MinF = 10 khi x = 2
B = 3x2 - 5x + 7 = 3(x2 - 5/3x + 25/36) + 59/12 = 3(x - 5/3)2 + 59/12 \(\ge\)59/12 \(\forall\)x
Dấu "=" xảy ra <=> x - 5/3 = 0 <=> x = 5/3
Vậy MinB = 59/12 khi x = 5/3
G = (x - 3)2 + (x - 2)2 = x2 - 6x + 9 + x2 - 4x + 4 = 2x2 - 10x + 13 = 2(x2 - 5x + 25/4) + 1/2 = 2(x - 5/2)2 + 1/2 \(\ge\)1/2 \(\forall\)x
Dấu "=" xảy ra <=> x - 5/2 = 0 <=> x = 5/2
Vậy MinG = 1/2 khi x = 5/2

B=-3x2-5y2+2x+7y-23
\(=-3x^2-5y^2+2x-7y-\frac{1}{3}-\frac{49}{20}-\frac{1213}{60}\)
\(=-3x^2+2x-\frac{1}{3}-5y^2+7y-\frac{49}{20}-\frac{1213}{60}\)
\(=-3\left(x^2-2\cdot\frac{1}{3}\cdot x+\frac{1}{3}^2\right)-5\left(y^2-2\cdot\frac{7}{10}\cdot y+y^2\right)-\frac{1213}{60}\)
\(=-3\left(x-\frac{1}{3}\right)^2-5\left(y-\frac{7}{10}\right)^2-\frac{1213}{60}\le0-\frac{1213}{60}\)
\(\Rightarrow B\le-\frac{1213}{60}\)
Dấu = khi x=1/3; y=7/10
Vậy .....

\(a,\Rightarrow x^3+9x^2+27x+27-9x^3-6x^2-x+8x^3+1-3x^2=54\\ \Rightarrow26x=26\Rightarrow x=1\\ b,\Rightarrow x^3-9x^2+27x-27-x^3+27+6x^2+12x+6+3x^2=-33\\ \Rightarrow39x=-39\Rightarrow x=-1\)

Bài 1:
\(a,=6x^2+6x\\ b,=15x^3-10x^2+5x\\ c,=6x^3+12x^2\\ d,=15x^4+20x^3-5x^2\\ e,=2x^2+3x-2x-3=2x^2+x-3\\ f,=3x^2-5x+6x-10=3x^2+x-10\)
Bài 2:
\(a,\Leftrightarrow3x^2+3x-3x^2=6\\ \Leftrightarrow3x=6\Leftrightarrow x=2\\ b,\Leftrightarrow6x^2+3x-6x^2+9x-2x-3=10\\ \Leftrightarrow10x=13\Leftrightarrow x=\dfrac{13}{10}\)

a: Ta có: \(\left(x^2+2\right)\left(x-4\right)-\left(x+2\right)^3=-16\)
\(\Leftrightarrow x^3-4x^2+2x-8-x^3-6x^2-12x-8=-16\)
\(\Leftrightarrow-10x^2-10x=0\)
\(\Leftrightarrow-10x\left(x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)
c: Ta có: \(x^3+3x^2+3x+28=0\)
\(\Leftrightarrow\left(x+1\right)^3=-27\)
\(\Leftrightarrow x+1=-3\)
hay x=-4

\(A=-3x^2+6x-7=-3\left(x^2-2x+1-1\right)-7\)
\(=-3\left(x-1\right)^2-4\le-4\)Dấu ''='' xảy ra khi x = 1
\(B=-2x^2+5x+1=-2\left(x^2-\dfrac{5}{2}x\right)+1\)
\(=-2\left(x^2-2.\dfrac{5}{4}x+\dfrac{25}{16}-\dfrac{25}{16}\right)+1\)
\(=-2\left(x-\dfrac{5}{4}\right)^2+\dfrac{33}{8}\le\dfrac{33}{8}\)Dấu ''='' xảy ra khi x = 5/4
C;D chỉ có GTNN thôi bạn nhé \(C=2x^2-8x+13=2\left(x^2-4x+4-4\right)+13\)
\(=2\left(x-2\right)^2+5\ge5\)Dấu ''='' xảy ra khi x = 2
\(D=x^2-3x+5=x^2-2.\dfrac{3}{2}x+\dfrac{9}{4}-\dfrac{9}{4}+5\)
\(=\left(x-\dfrac{3}{2}\right)^2+\dfrac{11}{4}\ge\dfrac{11}{4}\)Dấu ''='' xảy ra khi x = 3/2
d: Ta có: \(D=x^2-3x+5\)
\(=x^2-2\cdot x\cdot\dfrac{3}{2}+\dfrac{9}{4}+\dfrac{11}{4}\)
\(=\left(x-\dfrac{3}{2}\right)^2+\dfrac{11}{4}\ge\dfrac{11}{4}\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{3}{2}\)
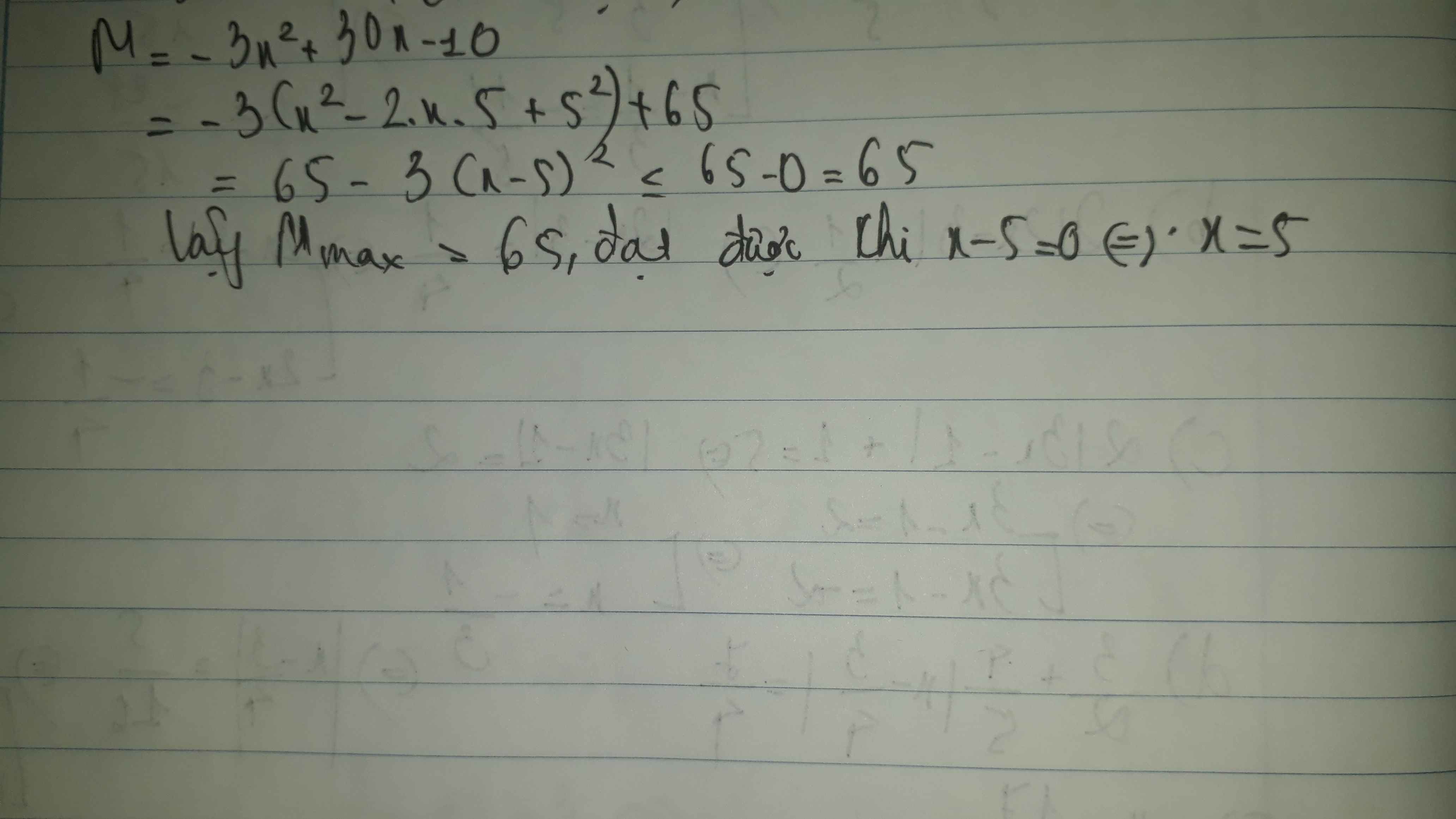
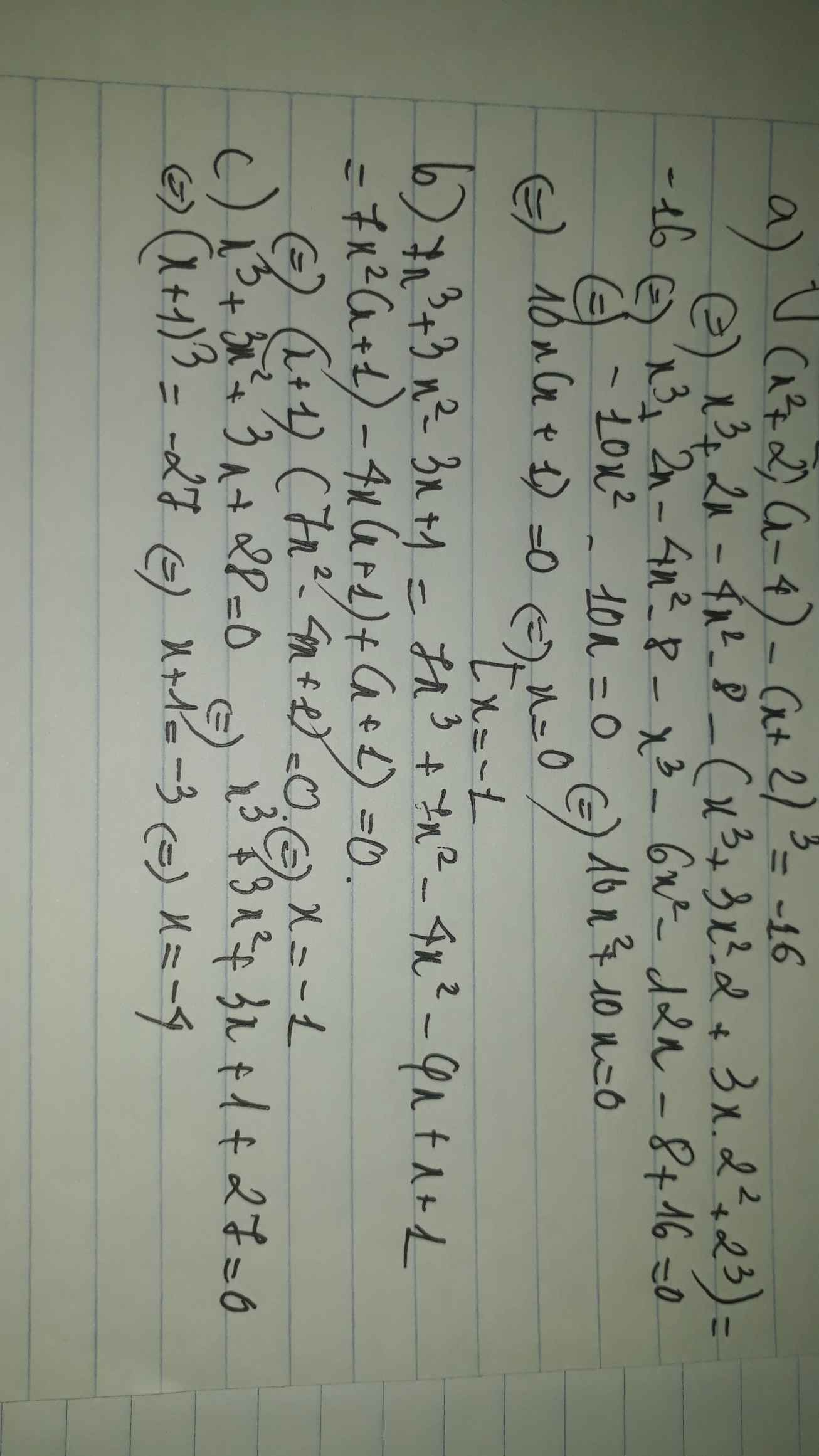
Đặt \(A=-3x^2+x-\frac{2}{3}=-3\left(x^2-\frac{x}{3}+\frac{1}{36}\right)-\frac{7}{12}=-3\left(x-\frac{1}{6}\right)^2-\frac{7}{12}\le-\frac{7}{12}\)
Vậy GTLN của A là \(-\frac{7}{12}\) khi \(x=\frac{1}{6}\)
Học tốt nhé em :)