FY
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
17 tháng 12 2019
Đáp án C
Phương trình hoành độ giao điểm là:
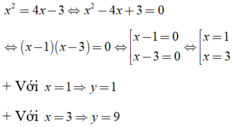
Do đó tọa độ giao điểm là (1; 1), (3; 9)
28 tháng 11 2023
a:
b: phương trình hoành độ giao điểm là:
4x+2=2x-2
=>4x-2x=-2-2
=>2x=-4
=>x=-2
Thay x=-2 vào y=4x+2, ta được:
\(y=4\cdot\left(-2\right)+2=-8+2=-6\)
Vậy: M(-2;-6)
c: Tọa độ A là:
\(\left\{{}\begin{matrix}y=0\\4x+2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\4x=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Tọa độ B là:
\(\left\{{}\begin{matrix}y=0\\2x-2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\2x=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\x=1\end{matrix}\right.\)
Vậy: B(1;0); A(-1/2;0)
d: M(-2;-6); B(1;0); A(-1/2;0)
\(MA=\sqrt{\left(-\dfrac{1}{2}+2\right)^2+\left(0-6\right)^2}=\dfrac{3\sqrt{17}}{2}\)
\(MB=\sqrt{\left(1+2\right)^2+\left(0+6\right)^2}=3\sqrt{5}\)
\(AB=\sqrt{\left(-\dfrac{1}{2}-1\right)^2+\left(0-0\right)^2}=\dfrac{3}{2}\)
Chu vi tam giác MAB là:
\(C_{MAB}=MA+MB+AB=\dfrac{3}{2}+3\sqrt{5}+\dfrac{3\sqrt{17}}{2}\)
Xét ΔMAB có \(cosAMB=\dfrac{MA^2+MB^2-AB^2}{2\cdot MA\cdot MB}=\dfrac{9}{\sqrt{85}}\)
=>\(sinAMB=\sqrt{1-\left(\dfrac{9}{\sqrt{85}}\right)^2}=\dfrac{2}{\sqrt{85}}\)
Diện tích tam giác MAB là:
\(S_{AMB}=\dfrac{1}{2}\cdot MA\cdot MB\cdot sinAMB=\dfrac{1}{2}\cdot\dfrac{3\sqrt{17}}{2}\cdot3\sqrt{5}\cdot\dfrac{2}{\sqrt{85}}\)
\(=\dfrac{9}{2}\)
25 tháng 11 2023
a: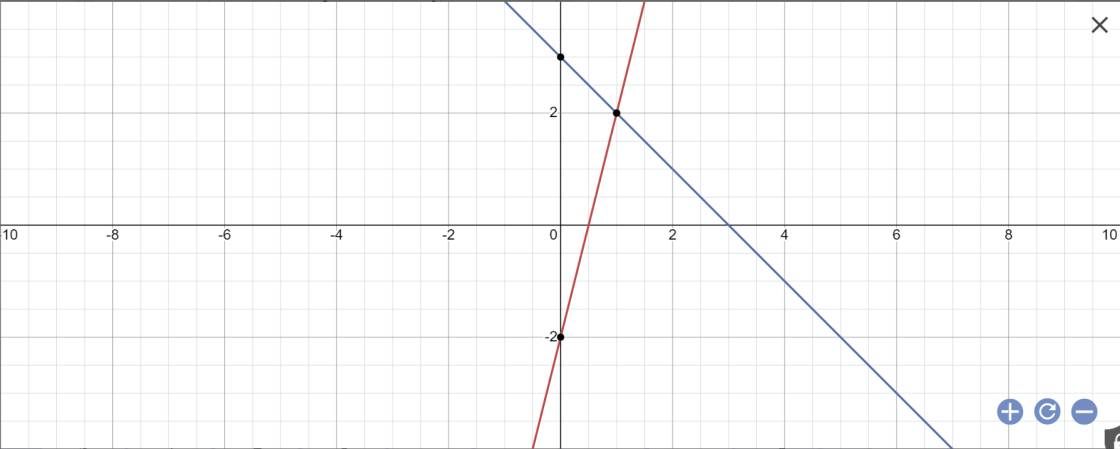 
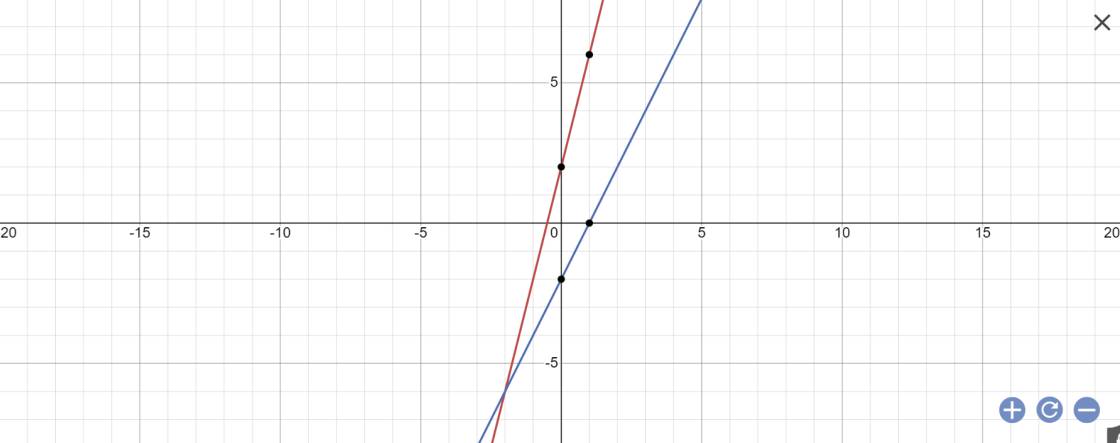
b: Phương trình hoành độ giao điểm là:
4x-2=-x+3
=>4x+x=3+2
=>5x=5
=>x=1
Thay x=1 vào y=-x+3, ta được:
\(y=-1+3=2\)
Vậy: M(1;2)
c: Gọi \(\alpha;\beta\) lần lượt là góc tạo bởi (d1),(d2) với trục Ox
(d1): y=4x-2
=>\(tan\alpha=4\)
=>\(\alpha=76^0\)
(d2): y=-x+3
=>\(tan\beta=-1\)
=>\(\beta=135^0\)
d: Thay y=6 vào (d1), ta được:
4x-2=6
=>4x=8
=>x=2
=>A(2;6)
Thay x=6/2=3 vào (d2), ta được:
\(y=-3+3=0\)
vậy: B(3;0)
Vì (d):y=ax+b đi qua A(2;6) và B(3;0) nên ta có hệ phương trình:
\(\left\{{}\begin{matrix}2a+b=6\\3a+b=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2a+b-3a-b=6-0\\3a+b=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-a=6\\b=-3a\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}a=-6\\b=-3\cdot\left(-6\right)=18\end{matrix}\right.\)
Vậy: (d): y=-6x+18
e: A(2;6); B(3;0); M(1;2)
\(AM=\sqrt{\left(1-2\right)^2+\left(2-6\right)^2}=\sqrt{17}\)
\(BM=\sqrt{\left(1-3\right)^2+\left(2-0\right)^2}=2\sqrt{2}\)
\(AB=\sqrt{\left(3-2\right)^2+\left(0-6\right)^2}=\sqrt{37}\)
Chu vi tam giác AMB là:
\(C_{AMB}=\sqrt{17}+2\sqrt{2}+\sqrt{37}\)
Xét ΔAMB có
\(cosAMB=\dfrac{MA^2+MB^2-AB^2}{2\cdot MA\cdot MB}=\dfrac{17+8-37}{2\cdot2\sqrt{2}\cdot\sqrt{17}}=\dfrac{-3}{\sqrt{34}}\)
=>\(\widehat{AMB}\simeq121^0\) và \(sinAMB=\sqrt{1-\left(-\dfrac{3}{\sqrt{34}}\right)^2}=\dfrac{5}{\sqrt{34}}\)
Xét ΔAMB có
\(\dfrac{AB}{sinAMB}=\dfrac{AM}{sinABM}=\dfrac{BM}{sinBAM}\)
=>\(\dfrac{\sqrt{17}}{sinABM}=\dfrac{2\sqrt{2}}{sinBAM}=\sqrt{37}:\dfrac{5}{\sqrt{34}}\)
=>\(sinABM\simeq0,58;\widehat{BAM}\simeq0,4\)
=>\(\widehat{ABM}\simeq35^0;\widehat{BAM}\simeq24^0\)
17 tháng 8 2021
a: Phương trình hoành độ giao điểm là:
3x-4=4x-6
\(\Leftrightarrow3x-4x=-6+4\)
\(\Leftrightarrow-x=-2\)
hay x=2
Thay x=2 vào \(\left(d1\right)\), ta được:
\(y=3\cdot2-4=2\)
b: Thay y=0 vào \(\left(d1\right)\), ta được:
\(3x-4=0\)
hay \(x=\dfrac{4}{3}\)
Thay x=0 vào \(\left(d1\right)\), ta được:
\(y=3\cdot0-4=-4\)
Vậy: \(A\left(\dfrac{4}{3};0\right);B\left(0;-4\right)\)
AH
Akai Haruma
Giáo viên
18 tháng 12 2021
Lời giải:
a.
Đồ thị xanh lá: $y=2x+1$
Đồ thị xanh dương: $y=x-3$
b.
PT hoành độ giao điểm:
$y=2x+1=x-3$
$\Leftrightarrow x=-4$
$y=x-3=(-4)-3=-7$
Vậy tọa độ điểm $M$ là $(-4;-7)$
NM
Nguyễn Minh Quang
Giáo viên
29 tháng 8 2021
Xét d cắt với Ox khi đó \(y=0\Rightarrow-4x+3=0\Leftrightarrow x=\frac{3}{4}\) Vậy giao với Ox tại điểm \(\left(\frac{3}{4};0\right)\)
d cắt với Oy khi đó : \(x=0\Rightarrow y=-4.0+3=3\) vậy giao với Oy tại điểm \(\left(0,3\right)\)
11 tháng 3 2022
làm bài này đâu nhất thiết phải dùng cách nào đâu bạn, vận dụng cách khoa học nhất là đc rồi nhé
a, bạn tự vẽ
b, Theo bài ra ta có hệ
\(\left\{{}\begin{matrix}2x^2+4x+2=0\\y=2x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x^2+4x+2=0\\y=2x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(x+1\right)^2=0\\y=2x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=2\end{matrix}\right.\)
Vậy (P) cắt (d) tại A(-1;2)
Phương trình hoành độ giao điểm của hai đồ thị :
\(4x^2=4x+3\Leftrightarrow4x^2-4x=3\)
\(\Leftrightarrow4x^2-4x+1=3+1\)
\(\Leftrightarrow\left(2x-1\right)^2=4\Leftrightarrow|2x-1|=2\)
\(\Leftrightarrow\orbr{\begin{cases}2x-1=2\\2x-1=-2\end{cases}\Leftrightarrow\orbr{\begin{cases}x=\frac{3}{2}\\x=-\frac{1}{2}\end{cases}}}\)
Vậy tọa độ giao điểm là :\(\left(\frac{3}{2};9\right)\) và \(\left(-\frac{1}{2};1\right)\)