Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: 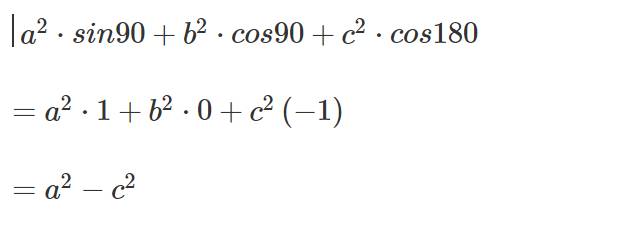
b: \(B=3-sin^290^0+2\cdot cos^260^0-3\cdot tan^245^0\)
\(=3-1+2\cdot\left(\dfrac{1}{2}\right)^2-3\cdot1^2\)
\(=2-3+2\cdot\dfrac{1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\)
c: \(C=sin^245^0-2\cdot sin^250^0+3\cdot cos^245^0-2\cdot sin^240^0+4\cdot tan55\cdot tan35\)
\(=\left(\dfrac{\sqrt{2}}{2}\right)^2+3\cdot\left(\dfrac{\sqrt{2}}{2}\right)^2-2\cdot\left(sin^250^0+sin^240^0\right)+4\)
\(=\dfrac{1}{2}+3\cdot\dfrac{1}{2}-2+4\)
\(=2-2+4=4\)

a.Ta có : \(x\in\left(\pi;\dfrac{3}{2}\pi\right)\Rightarrow cosx< 0\)
\(cosx=-\sqrt{1-sin^2x}=-\sqrt{1-0,8^2}=-0,6\)
\(tanx=\dfrac{4}{3};cotx=\dfrac{3}{4}\)
b. cos 2x = \(cos^2x-sin^2x=0,6^2-0,8^2=-0,28\)
\(P=2.cos2x=-0,56\)
\(Q=tan\left(2x+\dfrac{\pi}{3}\right)=\dfrac{tan2x+tan\dfrac{\pi}{3}}{1-tan2x.tan\dfrac{\pi}{3}}=\dfrac{tan2x+\sqrt{3}}{1-tan2x.\sqrt{3}}\)
tan 2x = \(\dfrac{2tanx}{1-tan^2x}=\dfrac{\dfrac{2.4}{3}}{1-\left(\dfrac{4}{3}\right)^2}=\dfrac{-24}{7}\)
\(Q=\dfrac{-\dfrac{24}{7}+\sqrt{3}}{1+\dfrac{24}{7}.\sqrt{3}}\) \(=\dfrac{-24+7\sqrt{3}}{7+24\sqrt{3}}\)

Ta có: \(\sin {70^o} = \cos {20^o};\;\cos {110^o} = - \cos {70^o} = - \sin {20^o}\)
\(\begin{array}{l} \Rightarrow A = {(\sin {20^o} + \cos {20^o})^2} + {(\cos {20^o} - \sin {20^o})^2}\\ = ({\sin ^2}{20^o} + {\cos ^2}{20^o} + 2\sin {20^o}\cos {20^o}) + ({\cos ^2}{20^o} + {\sin ^2}{20^o} - 2\sin {20^o}\cos {20^o})\\ = 2({\sin ^2}{20^o} + {\cos ^2}{20^o})\\ = 2\end{array}\)
Ta có: \(\tan {110^o} = - \tan {70^o} = - \cot {20^o};\;\cot {110^o} = - \cot {70^o} = - \tan {20^o}.\)
\( \Rightarrow B = \tan {20^o} + \cot {20^o} + ( - \cot {20^o}) + ( - \tan {20^o}) = 0\)

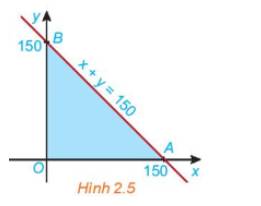
a) Thay tọa độ điểm O, A, B vào F(x;y) ta được:
F(0;0)=2.0+3.0=0
F(150;0)=2.150+3.0=300
F(0;150)=2.0+3.150=450.
b) Lấy một điểm bất kì trong miền tam giác OAB.
Vì miền OAB là miền nghiệm của hệ bất phương trình \(\left\{ \begin{array}{l}x \ge 0\\y \ge 0\\x + y \le 150\end{array} \right.\) nên mọi điểm (x;y) thuộc miền OAB thỏa mãn \(x \ge 0\).
Vì miền OAB là miền nghiệm của hệ bất phương trình \(\left\{ \begin{array}{l}x \ge 0\\y \ge 0\\x + y \le 150\end{array} \right.\) nên mọi điểm (x;y) thuộc miền OAB thỏa mãn \(y \ge 0\).
Vậy \(x \ge 0\) và \(y \ge 0\).
=> \(F\left( {x;y} \right) = 2x + 3y \ge 2.0 + 3.0 = 0\)
Vậy giá trị nhỏ nhất của F(x;y) trên miền OAB là 0.
c) Vì miền OAB là miền nghiệm của hệ bất phương trình \(\left\{ \begin{array}{l}x \ge 0\\y \ge 0\\x + y \le 150\end{array} \right.\) nên mọi điểm (x;y) thuộc miền OAB thỏa mãn \(x + y \le 150\)
Như vậy với mỗi điểm trong miền tam giác OAB thì đều có tổng \(x + y \le 150\)
Quan sát miền OAB ta thấy điểm B(0;150) là điểm có tung độ lớn nhất nên mọi điểm (x;y) thuộc miền OAB đều có \(y \le 150\).
Vậy ta có: \(F\left( {x;y} \right) = 2x + 3y\)\( = 2.\left( {x + y} \right) + y\)\( \le 2.150 + 150 = 450\)
Dấu “=” xảy ra khi x+y=150 và y=150. Hay x=0, y=150.
Giá trị lớn nhất trên miền OAB là 450 tại điểm B.

\(\sin\alpha=-\dfrac{1}{2}\)
\(\cos\alpha=\dfrac{\sqrt{3}}{2}\)
\(\tan\alpha=-\dfrac{\sqrt{3}}{3}\)
\(\cot\alpha=-\sqrt{3}\)

\(tana-cota=2\sqrt{3}\Rightarrow\left(tana-cota\right)^2=12\)
\(\Rightarrow\left(tana+cota\right)^2-4=12\Rightarrow\left(tana+cota\right)^2=16\)
\(\Rightarrow P=4\)
\(sinx+cosx=\dfrac{1}{5}\Rightarrow\left(sinx+cosx\right)^2=\dfrac{1}{25}\)
\(\Rightarrow1+2sinx.cosx=\dfrac{1}{25}\Rightarrow sinx.cosx=-\dfrac{12}{25}\)
\(P=\dfrac{sinx}{cosx}+\dfrac{cosx}{sinx}=\dfrac{sin^2x+cos^2x}{sinx.cosx}=\dfrac{1}{sinx.cosx}=\dfrac{1}{-\dfrac{12}{25}}=-\dfrac{25}{12}\)

Chọn B.
Ta có
C = ( tan50 . tan 850 ) .( tan 150 tan 750 ) ...tan 450
= ( tan50 .cot 50 ) .( tan 150 cot 150 ) ..tan 450 = 1
( do với 2 góc phụ nhau thì tan góc này bằng cot góc kia)

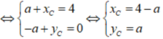
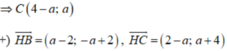
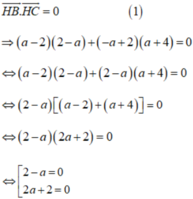
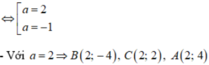
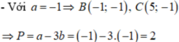
a/
\(tana+tanb=\frac{sina}{cosa}+\frac{sinb}{cosb}=\frac{sinacosb+cosa.sinb}{cosa.cosb}=\frac{sin\left(a+b\right)}{cosa.cosb}\)
\(C=tan80\left(tan20+tan140\right)+tan20.tan120\)
\(C=tan80.\frac{sin160}{cos20.cos140}+\frac{sin20.sin140}{cos20.cos140}\)
\(C=\frac{sin80}{cos80}.\frac{2.sin80.cos80}{\frac{1}{2}\left(cos160+cos120\right)}+\frac{-\frac{1}{2}\left(cos160-cos120\right)}{\frac{1}{2}\left(cos160+cos120\right)}\)
\(C=\frac{4sin^280}{cos160+cos120}-\frac{cos160-cos120}{cos160+cos120}\)
\(C=\frac{2\left(1-cos160\right)-cos160+cos120}{cos160+cos120}=\frac{2+cos120-3cos160}{cos120+cos160}\)
\(C=\frac{2-\frac{1}{2}-3cos160}{-\frac{1}{2}+cos160}=\frac{3-6cos160}{2cos160-1}=-3\)
b/
\(cos^275-sin^275=cos150=-\frac{\sqrt{3}}{2}\)