K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
24 tháng 9 2021
b: Ta có: \(\left(x-2\right)^3-x^2\left(x-6\right)=4\)
\(\Leftrightarrow x^3-6x^2+12x-8-x^3+6x^2=4\)
\(\Leftrightarrow12x=12\)
hay x=2
d: Ta có: \(3\left(x-1\right)^2-3x\left(x-5\right)=1\)
\(\Leftrightarrow3x^2-6x+3-3x^2+15x=1\)
\(\Leftrightarrow9x=-2\)
hay \(x=-\dfrac{2}{9}\)
CM
24 tháng 9 2018
a) Rút gọn được VT = 9x + 7. Từ đó tìm được x = 1.
b) Rút gọn được VT = 2x + 8. Từ đó tìm được x = 7 2 .
3 tháng 8 2021
(x-1)2-1+x2-(1-x)(x+3)=0
⇒x2-2x+1-1+x2-x(1-x)+3(1-x)=0
⇒x2-2x+1-1+x2-x+x2+3-3x=0
⇒3x2-6x+3=0
⇒3(x2-2x+1)=0
⇒x2-2x+1=0
⇒(x-1)2=0
⇒x-1=0
⇒x=1
AH
Akai Haruma
Giáo viên
3 tháng 8 2021
Lời giải:
$(x-1)^2-1+x^2-(1-x)(x+3)=0$
$\Leftrightarrow (x^2-2x+1)-1+x^2-(3-x^2-2x)=0$
$\Leftrightarrow x^2-2x+1-1+x^2-3+x^2+2x=0$
$\Leftrightarrow 3x^2-3=0$
$\Leftrightarrow x^2-1=0$
$\Leftrightarrow (x-1)(x+1)=0$
$\Leftrightarrow x=1$ hoặc $x=-1$
Bài 1.
23 tháng 9 2021
c: Ta có: \(\left(x+3\right)^3-x\left(3x+1\right)^2+\left(2x+1\right)\left(4x^2-2x+1\right)=28\)
\(\Leftrightarrow x^3+9x^2+27x+27-9x^3-6x^2-x+8x^3+1=28\)
\(\Leftrightarrow3x^2+26x=0\)
\(\Leftrightarrow x\left(3x+26\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{26}{3}\end{matrix}\right.\)
23 tháng 9 2021
\(a,\Leftrightarrow x^2+8x+16-x^3-12x^2=16\\ \Leftrightarrow x^3+11x^2-8x=0\\ \Leftrightarrow x\left(x^2+11x-8\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x^2+11x-8=0\left(1\right)\end{matrix}\right.\\ \Delta\left(1\right)=121+32=153\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-11-3\sqrt{17}}{2}\\x=\dfrac{-11+3\sqrt{17}}{2}\end{matrix}\right.\\ S=\left\{0;\dfrac{-11-3\sqrt{17}}{2};\dfrac{-11+3\sqrt{17}}{2}\right\}\)
\(c,\Leftrightarrow x^3+9x^2+27x+27-9x^3-6x^2-x+8x^3+1=28\\ \Leftrightarrow3x^2+26x=0\\ \Leftrightarrow x\left(3x+26\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{26}{3}\end{matrix}\right.\\ d,\Leftrightarrow x^3-6x^2+12x-8-x^3-125-6x^2=11\\ \Leftrightarrow-12x^2+12x-144=0\\ \Leftrightarrow x^2-x+12=0\Leftrightarrow\left[{}\begin{matrix}x=4\\x=3\end{matrix}\right.\)
NM
3
H9
HT.Phong (9A5)
CTVHS
16 tháng 11 2023
1. a) \(7x^2\left(2x^3+3x^5\right)=7x^2\cdot2x^3+7x^2\cdot3x^5=14x^5+21x^7\)
b) \(\left(x^3-x^2+x-1\right):\left(x-1\right)=\dfrac{x^3-x^2+x-1}{x-1}\)
\(=\dfrac{x^2\left(x-1\right)+\left(x-1\right)}{x-1}=\dfrac{\left(x-1\right)\left(x^2+1\right)}{x-1}=x^2+1\)
16 tháng 11 2023
2: \(x^2-8x+7=0\)
=>\(x^2-x-7x+7=0\)
=>\(x\left(x-1\right)-7\left(x-1\right)=0\)
=>\(\left(x-1\right)\left(x-7\right)=0\)
=>\(\left[{}\begin{matrix}x-1=0\\x-7=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=7\end{matrix}\right.\)
1:
a: \(7x^2\left(2x^3+3x^5\right)=7x^2\cdot2x^3+7x^2\cdot3x^5=21x^7+14x^5\)
b: \(\dfrac{x^3-x^2+x-1}{x-1}=\dfrac{x^2\left(x-1\right)+\left(x-1\right)}{\left(x-1\right)}\)
\(=x^2+1\)
LC
3
MA
16 tháng 8 2021
a, (x+2)2+(x-3)2=2x(x+7)
x.2+2.2+x.2+(-3).2-2x=8
2x+4+2x-6-2x=8
(2x+2x)+(4-6)=8
4x-2=8
4x=8+2
4x=10
X=10:4
X=5/2
3 tháng 9 2021
\(\left(x-1\right)^3+3\left(x+1\right)^2=\left(x+2\right)\left(x^2-2x+4\right)\)
\(\Leftrightarrow x^3-3x^2+3x-1+3x^2+6x+1=x^3+8\)
\(\Leftrightarrow x^3+9x=x^3+8\)
hay \(x=\dfrac{8}{9}\)
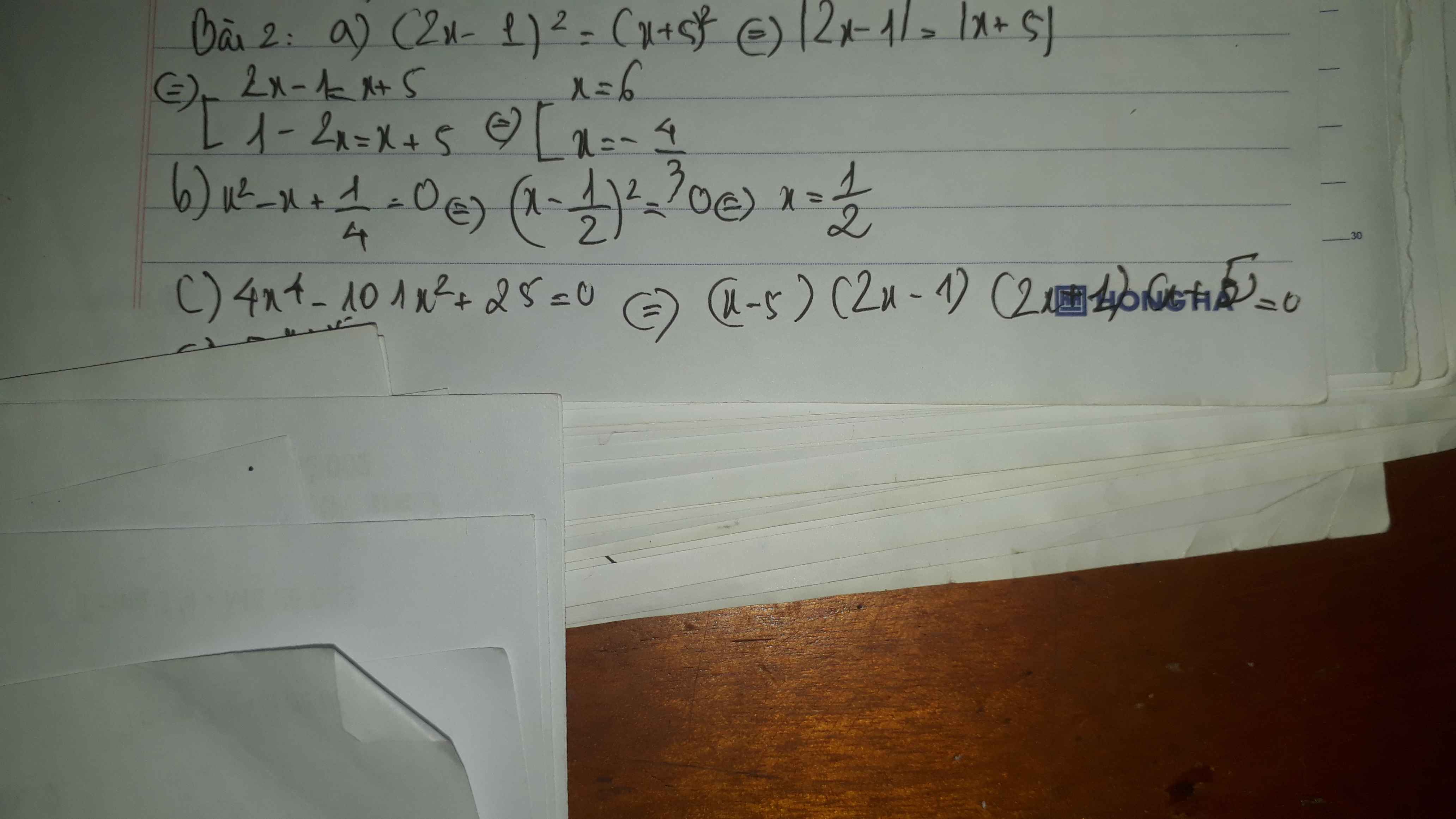
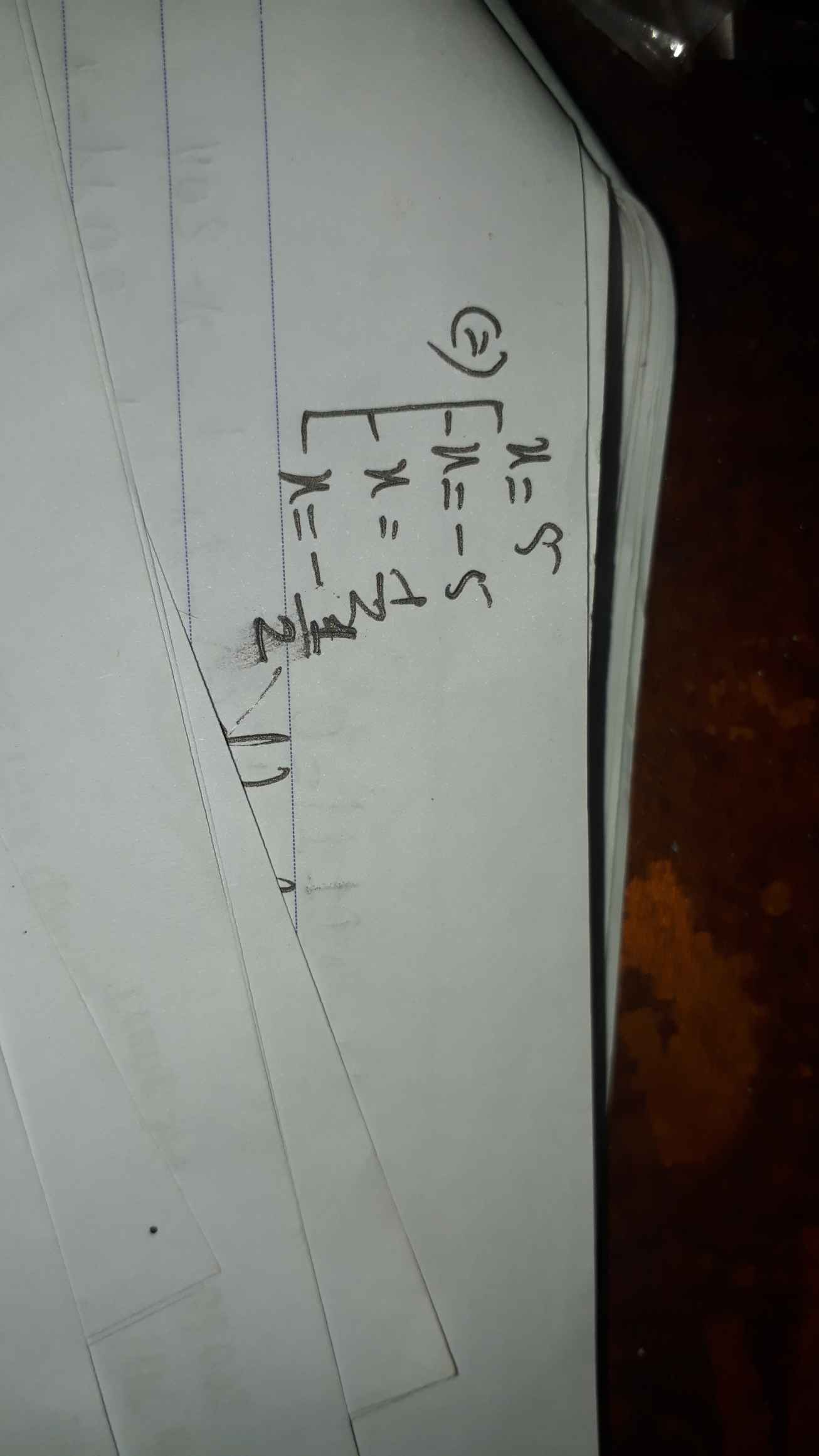
Lời giải:
ĐKXĐ: $x\neq -1$.
PT $\Leftrightarrow (x-\frac{x}{x+1})^2+\frac{2x^2}{x+1}=1$
$\Leftrightarrow (\frac{x^2}{x+1})^2+\frac{2x^2}{x+1}=1$
Đặt $\frac{x^2}{x+1}=a$ thì PT trở thành:
$a^2+2a=1$
$\Leftrightarrow (a+1)^2=2$
$\Leftrightarrow a+1=\pm \sqrt{2}$
$\Leftrightarrow a=\pm \sqrt{2}-1$
$\Leftrightarrow \frac{x^2}{x+1}=\pm \sqrt{2}-1$
$\Rightarrow x^2=(\sqrt{2}-1)(x+1)$ hoặc $x^2=(-\sqrt{2}-1)(x+1)$
Nếu $x^2=(\sqrt{2}-1)(x+1)$
$\Leftrightarrow x^2-(\sqrt{2}-1)x-(\sqrt{2}-1)=0$
$\Rightarrow x=\frac{1}{2}(-1+\sqrt{2}\pm \sqrt{2\sqrt{2}-1})$
Nếu $x^2=-(\sqrt{2}-1)(x+1)$
$\Leftrightarrow x^2+(\sqrt{2}-1)x+(\sqrt{2}-1)=0$
Dễ thấy:
$\Delta=(\sqrt{2}-1)^2-4(\sqrt{2}-1)<0$ nên pt này vô nghiệm.