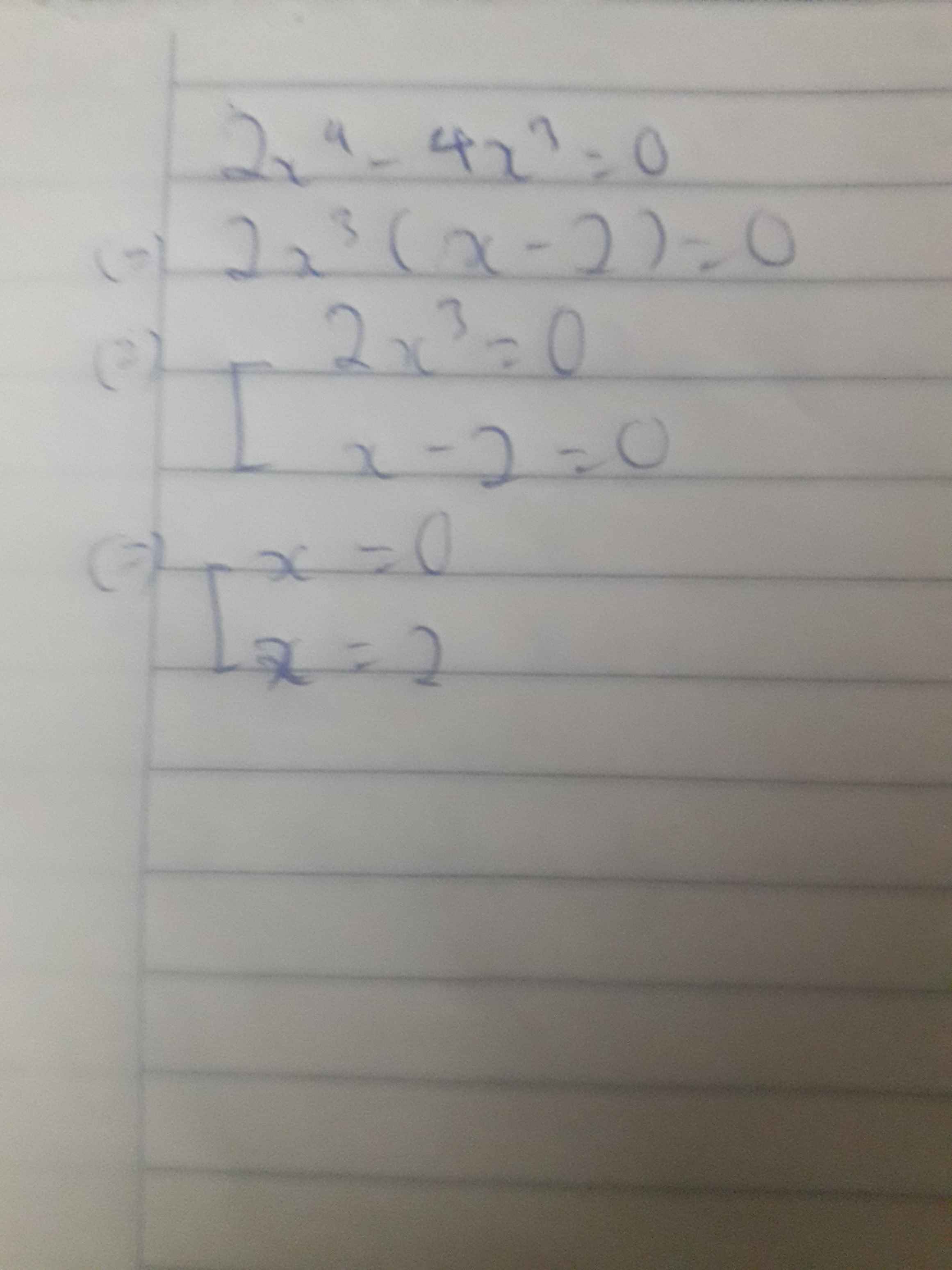K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LM
2
PN
20 tháng 5 2021
\(x^2-3x-4=0\)
\(< =>x^2+x-4x-4=0\)
\(< =>x\left(x+1\right)-4\left(x+1\right)=0\)
\(< =>\left(x-4\right)\left(x+1\right)=0\)
\(< =>\orbr{\begin{cases}x=4\\x=-1\end{cases}}\)
PN
20 tháng 5 2021
\(2x^3-x^2-2x+1=0\)
\(< =>x^2\left(2x-1\right)-\left(2x-1\right)=0\)
\(< =>\left(x^2-1\right)\left(2x-1\right)=0\)
\(< =>\left(x-1\right)\left(x+1\right)\left(2x+1\right)=0\)
\(< =>\hept{\begin{cases}x=1\\x=-1\\x=-\frac{1}{2}\end{cases}}\)
HP
1
NV
Nguyễn Việt Lâm
Giáo viên
17 tháng 4 2022
a.
\(P-\left(5x^4-xyz\right)=xy+2x^4-6xyz+654\)
\(\Rightarrow P=5x^4-xyz+xy+2x^4-6xyz+654\)
\(\Rightarrow P=7x^4-7xyz+xy+654\)
b.
\(x^2-2x=0\)
\(\Leftrightarrow x\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
20 tháng 2 2022
\(\Leftrightarrow2x^3\left(x-2\right)=0\)
=>x=0 hoặc x=2
A
1
10 tháng 5 2022
Giả sử đa thức \(=0\)
\(\rightarrow x\left(2x-4\right)-3\left(4-2x\right)=0\)
\(2x^2-4x-12+6x=0\)
\(2x^2+2x-12=0\)
\(x^2+x-6=0\)
\(x^2+3x-2x-6=0\)
\(x\left(x+3\right)-2\left(x+3\right)=0\)
\(\left(x+3\right)\left(x-2\right)=0\)
\(\rightarrow\left[{}\begin{matrix}x+3=0\\x-2=0\end{matrix}\right.\) \(\rightarrow\left[{}\begin{matrix}x=-3\\x=2\end{matrix}\right.\)
Vậy \(x=\left\{-3;2\right\}\) là nghiệm của đa thức
AD
10 tháng 5 2022
x(2x - 4) - 3(4 - 2x) = 0
\(\Leftrightarrow\) x(2x - 4) + 3(2x - 4) = 0
\(\Leftrightarrow\) (2x - 4)(x + 3) = 0
\(\Leftrightarrow\left[{}\begin{matrix}2x-4=0\\x+3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=-3\end{matrix}\right.\)
10 tháng 5 2018
Đa thức 2x^4+3x+1 vô nghiệm vì:
2x^4>(=)0
3x>(=)0
1>0
=>2x^4+3x+1>0