LD
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
15 tháng 9 2016
T/C của gttđ là >= 0 nên
a) GTNN = -4
b) GTLN = 2
c) GTNN = 2
8 tháng 9 2019
\(\left|x+1,5\right|\ge0\forall x\)
Dấu " = " xảy ra khi
| x + 1,5 | = 0
x = -1,5
Vậy MinA = 0 <=> x = -1,5
b)
\(\left|x-2\right|\ge0\forall x\Rightarrow\left|x-2\right|-\frac{9}{10}\ge\frac{9}{10}\forall x\)
Dấu " = " xảy ra khi
| x - 2 | = 0
x = 2
Vậy MinA = \(\frac{9}{10}\)<=> x = 2
8 tháng 9 2019
\(-\left|2x-1\right|\le0\forall x\)
Dấu " = " xảy ra khi :
- | 2x - 1 | = 0
=> x = \(\frac{1}{2}\)
Vậy MaxA = 0 <=> x = \(\frac{1}{2}\)
b)
\(-\left|5x-3\right|\le0\forall x\Rightarrow4-\left|5x-3\right|\le4\)
Dấu " = " xảy ra khi :
- | 5x - 3 | = 0
=> x = \(\frac{3}{5}\)
Vậy MaxB = 4 <=> x = \(\frac{3}{5}\)
Study well
NM
4
16 tháng 7 2021
Với mọi x ta có :
\(\left|x+5\right|\ge0\)
\(\Leftrightarrow\left|x+5\right|+5\ge0\)
\(\Leftrightarrow A\ge5\)
Dấu "=" xảy ra \(\Leftrightarrow x=-5\)
Vậy..
13 tháng 3 2022
\(A=\left(x-1\right)^2+1.\\ \left(x-1\right)^2\ge0\forall x\in R.\\ 1>0.\\ \Rightarrow\left(x-1\right)^2+1\ge1\forall x\in R.\\ \Rightarrow A\ge1.\\ \Rightarrow A_{min}=1.\)
\(B=x^2+x^4-\dfrac{1}{2}.\\ x^2+x^4\ge0\forall x\in R.\\ \Leftrightarrow x^2+x^4-\dfrac{1}{2}\ge\dfrac{-1}{2}\forall x\in R.\\ \Rightarrow B\ge\dfrac{-1}{2}.\\ \Rightarrow B_{min}=\dfrac{-1}{2}.\)
\(D=\dfrac{2}{\left(x-1\right)^2}+1.\\ \left(x-1\right)^2\ge0\forall x\in R.\\ \Leftrightarrow\dfrac{2}{\left(x-1\right)^2}\ge0.\\ \Leftrightarrow\dfrac{2}{\left(x-1\right)^2}+1\ge1\forall x\in R.\\ \Rightarrow D\ge1.\\ \Rightarrow D_{min}=1.\)
NT
1
27 tháng 5 2021
Ta có \(\left|x-1\right|\ge0\)
\(\Rightarrow\left|x-1\right|+2012\ge2012\)
Dấu "=" xảy ra \(\Leftrightarrow x=1\)
Vậy Min = 2012 \(\Leftrightarrow x=1\)
NN
2
18 tháng 12 2019
\(A = | x + 2014 | + | x + 2015| + 2015\)
\(A = | x + 2014 | + | x + 2015 | + 2015 \)\(\ge\)
\(2015\)
\(Dấu " = " xảy \) \(ra\) \(\Leftrightarrow\)\(x + 2014 = 0 hoặc x + 2015= 0\)
\(\Leftrightarrow\)\(x = - 2014 hoặc x = - 2015\)
\(Min A = 2015\) \(\Leftrightarrow\)\(x = - 2014 hoặc x = - 2015\)
18 tháng 12 2019
\(A=\left|x+2014\right|+\left|x+2015\right|+2015\)
\(=\left|x+2014\right|+\left|-x-2015\right|+2015\)
Ta có: \(\left|x+2014\right|+\left|-x-2015\right|\ge\left|x+2014-x-2015\right|=1\)
\(\Rightarrow\left|x+2014\right|+\left|-x-2015\right|+2015\ge2016\)
Dấu"="xảy ra \(\Leftrightarrow\left(x+2014\right)\left(-x-2015\right)\ge0\)
\(\Leftrightarrow\hept{\begin{cases}x+2014\ge0\\-x-2015\ge0\end{cases}}\)hoặc \(\hept{\begin{cases}x+2014< 0\\-x-2015< 0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x\ge-2014\\x\le-2015\end{cases}}\)hoặc \(\hept{\begin{cases}x< -2014\\x>-2015\end{cases}\left(loai\right)}\)
\(\Leftrightarrow-2014\le x\le-2015\)
Vậy \(A_{min}=2016\)\(\Leftrightarrow-2014\le x\le-2015\)
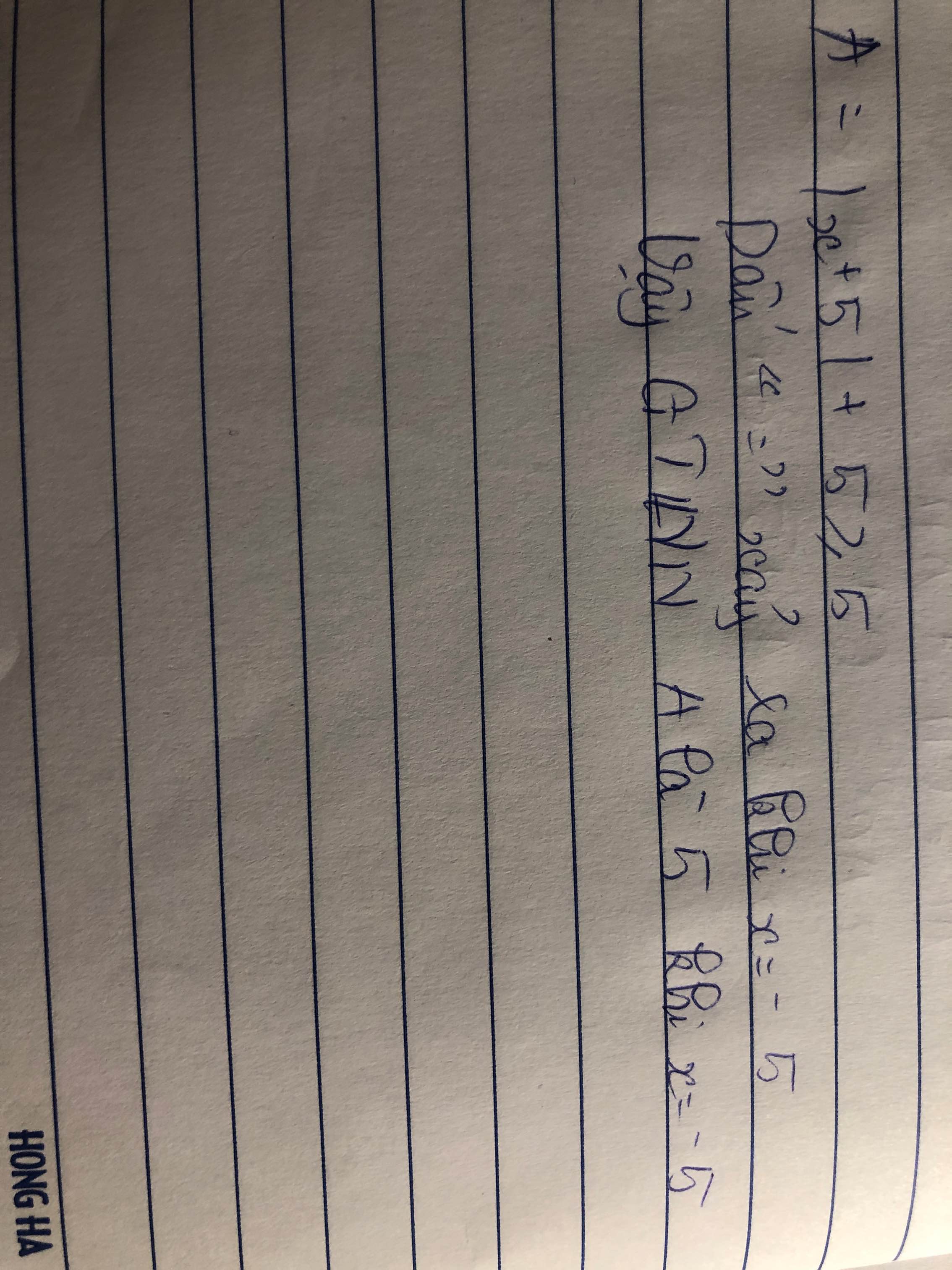
\(A=\left|x-102\right|+\left|2-x\right|\ge\left|x-102+2-x\right|=100\)
Dấu "=" xảy ra <=> \(\left(x-102\right)\left(2-x\right)\ge0\)
Xét 2 trường hợp:
\(\hept{\begin{cases}x-102\ge0\\2-x\ge0\end{cases}}\) Hoặc \(\hept{\begin{cases}x-102\le0\\2-x\le0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x\ge102\\x\le2\end{cases}}\) (Loại) Hoặc \(\hept{\begin{cases}x\le102\\x\ge2\end{cases}}\) (Nhận)
\(\Leftrightarrow2\le x\le102\)
Vậy AMin = 100 khi và chỉ khi \(2\le x\le102\)