K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
MN
1
25 tháng 9 2021
\(a,=-\left(x^2-2x+1\right)-3=-\left(x-1\right)^2-3\le-3\)
Dấu \("="\Leftrightarrow x=1\)
\(b,=-\left(x^2+4x+4\right)+4=-\left(x+2\right)^2+4\le4\)
Dấu \("="\Leftrightarrow x=-2\)
\(c,=-\left(9x^2-24x+16\right)-2=-\left(3x-4\right)^2-2\le-2\)
Dấu \("="\Leftrightarrow x=\dfrac{4}{3}\)
\(d,=-\left(x^2-4x+4\right)+3=-\left(x-2\right)^2+3\le3\)
Dấu \("="\Leftrightarrow x=2\)
MN
2
25 tháng 9 2021
a) x2 +x +1 = x2 + x + 1/4 + 3/4 =(x+1/2)2 + 3/4
=> GTNN a) =3/4 khi x=-1/2
b) 4x2 +4x -5 = 4x2 + 4x +1 -6 = (2x+1)2-6
=> GTNN b) = -6 khi x=-1/2
c) (x-3)(x+5) +4 = x2+2x -11 = x2+2x +1-12=(x+1)2-12
GTNN c) =12 khi x=-1
d) x2-4x+y2-8y+6=x2-4x+4+y2-8y+16-14=(x-2)2+(y-4)2-14
GTNN d) =-14 khi x=2 , y=4
25 tháng 9 2021
\(a,=\left(x^2+x+\dfrac{1}{4}\right)+\dfrac{3}{4}=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}\)
Dấu \("="\Leftrightarrow x=-\dfrac{1}{2}\)
\(b,=\left(4x^2+4x+1\right)-6=\left(2x+1\right)^2-6\ge-6\)
Dấu \("="\Leftrightarrow x=-\dfrac{1}{2}\)
\(c,=x^2+2x-15+4=\left(x+1\right)^2-12\ge-12\)
Dấu \("="\Leftrightarrow x=-1\)
\(d,=\left(x^2-4x+4\right)+\left(y^2-8y+16\right)-14=\left(x-2\right)^2+\left(y-4\right)^2-14\ge-14\)
Dấu \("="\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=4\end{matrix}\right.\)
NT
1
8 tháng 1 2021
Ta có: \(A=-2x^2-5x+3\)
\(=-2\left(x^2+\dfrac{5}{2}x-\dfrac{3}{2}\right)\)
\(=-2\left(x^2+2\cdot x\cdot\dfrac{5}{4}+\dfrac{25}{16}-\dfrac{49}{16}\right)\)
\(=-2\left(x+\dfrac{5}{4}\right)^2+\dfrac{49}{8}\)
Ta có: \(\left(x+\dfrac{5}{4}\right)^2\ge0\forall x\)
\(\Rightarrow-2\left(x+\dfrac{5}{4}\right)^2\le0\forall x\)
\(\Rightarrow-2\left(x+\dfrac{5}{4}\right)^2+\dfrac{49}{8}\le\dfrac{49}{8}\forall x\)
Dấu '=' xảy ra khi \(x+\dfrac{5}{4}=0\)
hay \(x=-\dfrac{5}{4}\)
Vậy: Giá trị lớn nhất của biểu thức \(A=-2x^2-5x+3\) là \(\dfrac{49}{8}\) khi \(x=-\dfrac{5}{4}\)
13 tháng 8 2023
=-3x^2+12x-12+12
=-3(x^2-4x+4)+12
==-3(x-2)^2+12<=12
Dấu = xảy ra khi x=2
27 tháng 12 2021
Bài 1:
\(A=x^2+6x+9+x^2-10x+25\)
\(=2x^2+4x+34\)
\(=2\left(x^2+2x+17\right)\)
\(=2\left(x+1\right)^2+32>=32\forall x\)
Dấu '=' xảy ra khi x=-1
1T
2
KS
27 tháng 12 2021
x + 3y = 10 <=> x = 10 - 3y thay vào D ta được:
D = (10 - 3y)2 + y2 = 100 - 60y + 9y2 + y2
D = 10y2 - 60y + 100 = 10(y2 - 6y + 10)
D = 10(y2 -2y3 + 9 + 1) = 10[(y - 3)2 + 1]
D = 10(y - 3)2 + 10 \(\ge\)10
Dấu "=" xảy ra khi: y - 3 = 0 <=> y = 3
=> x = 10 - 3y = 10 - 3.3= 1
Vậy gtnn D = 10 khi x = 1, y = 3
H2
1
19 tháng 4 2022
-Câu cuối thôi nha bạn :v
\(B=-5x^2-4x-\dfrac{19}{5}=-5\left(x^2+\dfrac{4}{5}x+\dfrac{19}{25}\right)=-5\left(x^2+2.\dfrac{2}{5}x+\dfrac{4}{25}+\dfrac{15}{25}\right)=-5\left(x+\dfrac{2}{5}\right)^2-\dfrac{15}{5}\le-3\)\(B_{max}=-3\Leftrightarrow x=\dfrac{-2}{5}\)
.
LN
1
LM
1
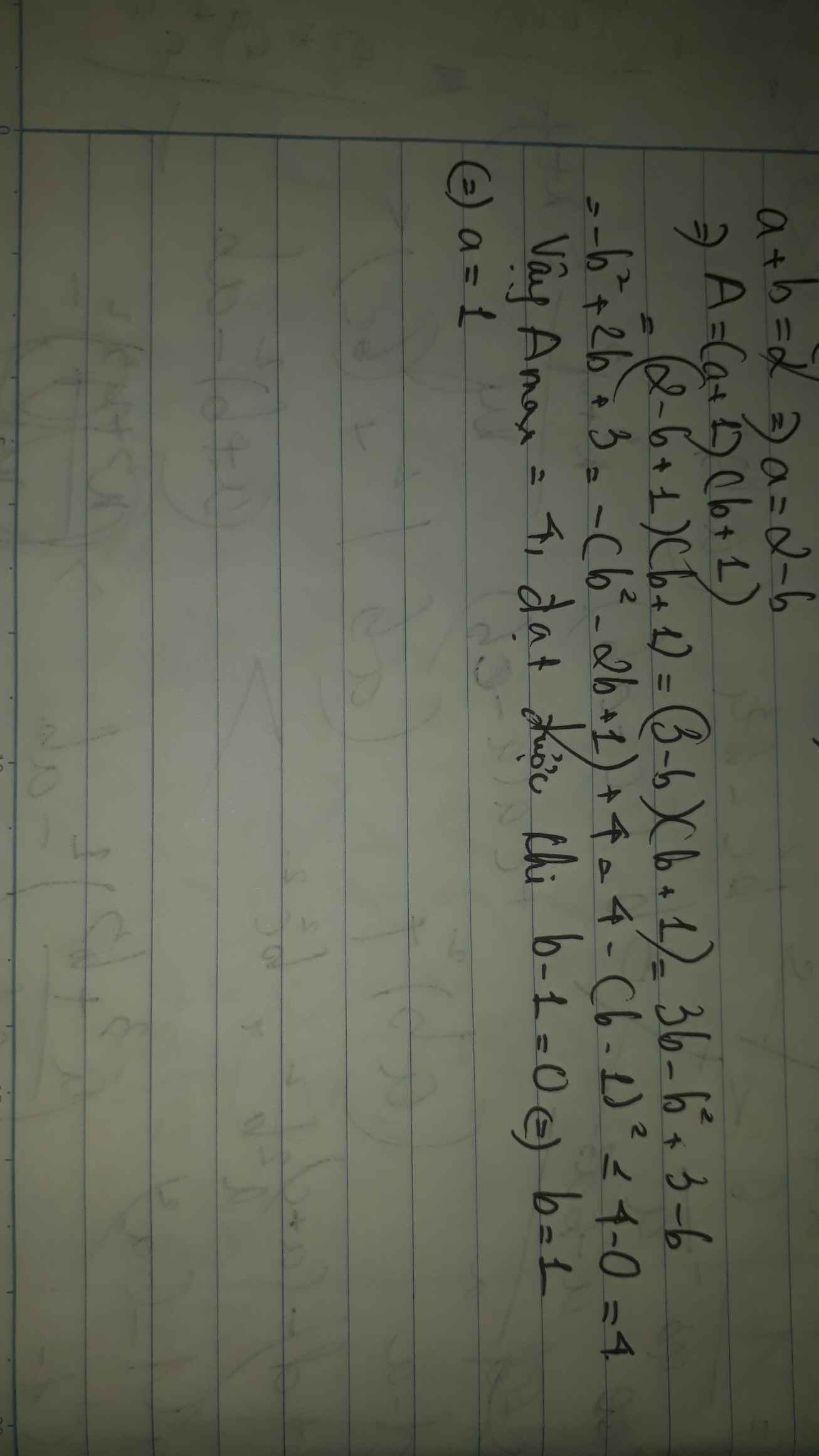
\(A=-\left(x^2-4x-3\right)=-\left(x^2-4x+4-7\right)=-\left(x-2\right)^2+7\le7\)
\(A_{max}=7\Leftrightarrow x=2\)
cảm ơn nha cậu nhìu nha!