Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Hàm số \(f\left(x\right)\) liên tục trên đoạn \(\left[\frac{1}{2};2\right]\)
+)\(f'\left(x\right)=\frac{x^2+2x}{\left(x+1\right)^2};f'\left(x\right)=0\Leftrightarrow x=0\notin\left[\frac{1}{2};2\right]\)hoặc \(x=-2\notin\left[\frac{1}{2};2\right]\)
+) \(f\left(\frac{1}{2}\right)=\frac{7}{6};f\left(2\right)=\frac{7}{3}\)
Vậy \(minf\left(x\right)_{x\in\left[\frac{1}{2};2\right]}=\frac{7}{6}\) khi \(x=\frac{1}{2}\)
\(maxf\left(x\right)_{x\in\left[\frac{1}{2};2\right]}=\frac{7}{3}\) khi \(x=2\)


Ta có :
\(f'\left(x\right)=2x\ln x-x=x\left(2\ln x-1\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=0\\\ln x=\frac{1}{2}\ln\sqrt{e}\end{array}\right.\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=0\notin\left[\frac{1}{e};e^2\right]\\x=\sqrt{e}\in\left[\frac{1}{e};e^2\right]\end{array}\right.\)
Mà : \(\begin{cases}f\left(\frac{1}{e}\right)=-\frac{1}{e^2}\\f\left(e\right)=\frac{e}{2}\\f\left(e^2\right)=2e^4\end{cases}\) \(\Rightarrow\begin{cases}Max_{x\in\left[\frac{1}{e};e^2\right]}f\left(x\right)=2e^4;x=e^2\\Min_{x\in\left[\frac{1}{e};e^2\right]}f\left(x\right)=\frac{-1}{e^2};x=\frac{1}{e}\end{cases}\)

a) f(x) = 2x3 – 3x2 – 12x + 1 ⇒ f’(x) = 6x2 – 6x – 12
f’(x) = 0 ⇔ x ∈ {-1, 2}
So sánh các giá trị:
f(x) = -3; f(-1) = 8;
f(2) = -19, f(52)=−332f(52)=−332
Suy ra:
maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19
b) f(x) = x2 lnx ⇒ f’(x)= 2xlnx + x > 0, ∀ x ∈ [1, e] nên f(x) đồng biến.
Do đó:
maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0
c) f(x) = f(x) = xe-x ⇒ f’(x)= e-x – xe-x = (1 – x)e-x nên:
f’(x) = 0 ⇔ x = 1, f’(x) > 0, ∀x ∈ (0, 1) và f’(x) < 0, ∀x ∈ (1, +∞)
nên:
maxx∈[0,+∞)f(x)=f(1)=1emaxx∈[0,+∞)f(x)=f(1)=1e
Ngoài ra f(x) = xe-x > 0, ∀ x ∈ (0, +∞) và f(0) = 0 suy ra
maxx∈[0,+∞)f(x)=f(0)=0maxx∈[0,+∞)f(x)=f(0)=0
d) f(x) = 2sinx + sin2x ⇒ f’(x)= 2cosx + 2cos2x
f’(x) = 0 ⇔ cos 2x = -cosx ⇔ 2x = ± (π – x) + k2π
⇔ x∈{−π+k2π;π3+k2π3}x∈{−π+k2π;π3+k2π3}
Trong khoảng [0,3π2][0,3π2] , phương trình f’(x) = 0 chỉ có hai nghiệm là x1=π3;x2=πx1=π3;x2=π
So sánh bốn giá trị : f(0) = 0; f(π3)=3√32;f(π)=0;f(3π2)=−2f(π3)=332;f(π)=0;f(3π2)=−2
Suy ra:
maxx∈[0,3π2]f(x)=f(π3)=3√32minx∈[0,3π2]f(x)=f(3π2)=−2

\(f\left(x\right)=\frac{x^2}{2}-4\ln\left(3-x\right)\) trên đoạn \(\left[-2;1\right]\)
Ta có :
\(f'\left(x\right)=x+\frac{4}{3-x}=\frac{-x^2+3x+4}{3-x}=0\Leftrightarrow-x^2+3x+4=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=-1\in\left[-2;1\right]\\x=4\notin\left[-2;1\right]\end{array}\right.\)
Mà :
\(\begin{cases}f\left(-2\right)=2-4\ln5\\f\left(-1\right)=\frac{1}{2}-8\ln2=\frac{1-16\ln2}{2}\\f\left(1\right)=\frac{1}{2}-4\ln2=\frac{1-8\ln2}{2}\end{cases}\) \(\Rightarrow\begin{cases}Max_{x\in\left[-2;1\right]}f\left(x\right)=\frac{1-8\ln2}{2};x=1\\Min_{x\in\left[-2;1\right]}f\left(x\right)=\frac{1-16\ln2}{2};x=-1\end{cases}\)

1/ \(f'\left(x\right)=\frac{3\sqrt{x^2+1}-\frac{x\left(3x+1\right)}{\sqrt{x^2+1}}}{x^2+1}=\frac{3\left(x^2+1\right)-3x^2-x}{\left(x^2+1\right)\sqrt{x^2+1}}=\frac{3-x}{\left(x^2+1\right)\sqrt{x^2+1}}\)
Hàm số đồng biến trên \(\left(-\infty;3\right)\) nghịch biến trên \(\left(3;+\infty\right)\)
\(\Rightarrow f\left(x\right)\) đạt GTLN tại \(x=3\)
\(f\left(x\right)_{max}=f\left(3\right)=\frac{10}{\sqrt{10}}=\sqrt{10}\)
2/ \(y'=\frac{\sqrt{x^2+2}-\frac{\left(x-1\right)x}{\sqrt{x^2+2}}}{x^2+2}=\frac{x^2+2-x^2+x}{\left(x^2+2\right)\sqrt{x^2+2}}=\frac{x+2}{\left(x^2+2\right)\sqrt{x^2+2}}\)
\(f'\left(x\right)=0\Rightarrow x=-2\in\left[-3;0\right]\)
\(y\left(-3\right)=-\frac{4\sqrt{11}}{11}\) ; \(y\left(-2\right)=-\frac{\sqrt{6}}{2}\) ; \(y\left(0\right)=-\frac{\sqrt{2}}{2}\)
\(\Rightarrow\left\{{}\begin{matrix}M=-\frac{\sqrt{2}}{2}\\N=-\frac{\sqrt{6}}{2}\end{matrix}\right.\) \(\Rightarrow MN=\frac{\sqrt{12}}{4}=\frac{\sqrt{3}}{2}\)
Tất cả các đáp án đều sai
3/ \(\left\{{}\begin{matrix}\left|x-3\right|\ge0\\\sqrt{x+1}>0\end{matrix}\right.\) \(\Rightarrow f\left(x\right)\ge0\) \(\forall x\Rightarrow N=0\) khi \(x=3\)
- Với \(0\le x< 3\Rightarrow f\left(x\right)=\left(3-x\right)\sqrt{x+1}\)
\(\Rightarrow f'\left(x\right)=-\sqrt{x+1}+\frac{\left(3-x\right)}{2\sqrt{x+1}}=\frac{-2\left(x+1\right)+3-x}{2\sqrt{x+1}}=\frac{-3x+1}{2\sqrt{x+1}}\)
\(f'\left(x\right)=0\Rightarrow x=\frac{1}{3}\)
- Với \(3< x\le4\Rightarrow f\left(x\right)=\left(x-3\right)\sqrt{x+1}\)
\(\Rightarrow f'\left(x\right)=\sqrt{x+1}+\frac{x-3}{2\sqrt{x+1}}=\frac{2\left(x+1\right)+x-3}{2\sqrt{x+1}}=\frac{3x-1}{2\sqrt{x+1}}>0\) \(\forall x>3\)
Ta có: \(f\left(0\right)=3\) ; \(f\left(\frac{1}{3}\right)=\frac{16\sqrt{3}}{9}\) ; \(f\left(4\right)=\sqrt{5}\)
\(\Rightarrow M=\frac{16\sqrt{3}}{9}\Rightarrow M+2N=\frac{16\sqrt{3}}{9}\)
Câu 2 hình như câu B mà người ta nói đạt GTLN . GTNN tại M , N nên là 0 x -2 =0


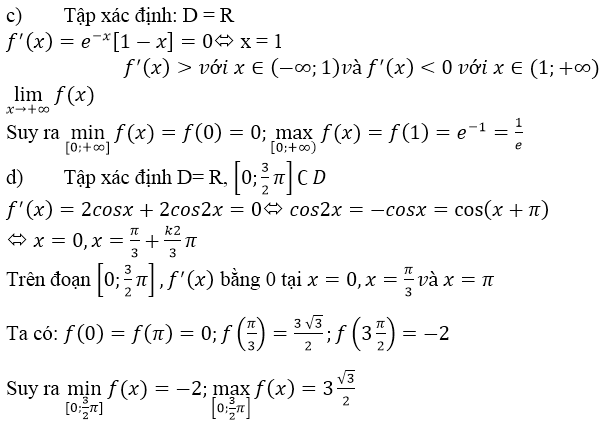
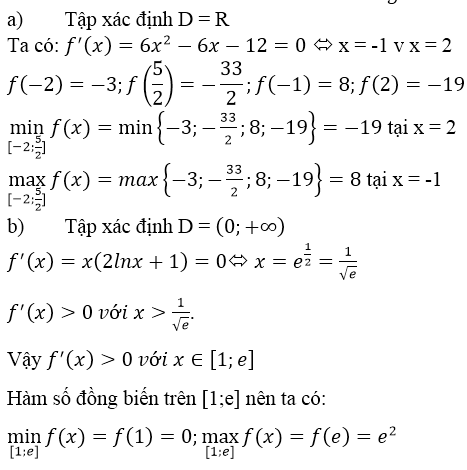
bn ơi câu a t chưa làm chưa biết nhưng câu b chắc chắn có Max tại x=-3 nhé ! Nếu bn chỉ tìm ra Min là chưa đủ