
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



\(a.\left(x-3\right)\left(x+4\right)-2\left(3x-2\right)=\left(x-4\right)^2\\\Leftrightarrow x^2+4x-3x-12-6x+4=x^2-8x+16\\\Leftrightarrow x^2-x^2+4x-3x-6x+8x=+12-4+16=0\\\Leftrightarrow 3x=24\\\Leftrightarrow x=8\)
Vậy \(x=8\) để \(A=B\)

a) A= (x-3)(x+4)-2(3x-2) và B= (x-4)²
ta có:
(x−3)(x+4)−2(3x−2)= (x-4)²
=> \(x^2-5x-8=x^2-8x+16\)
=>3x=24
=>x=8
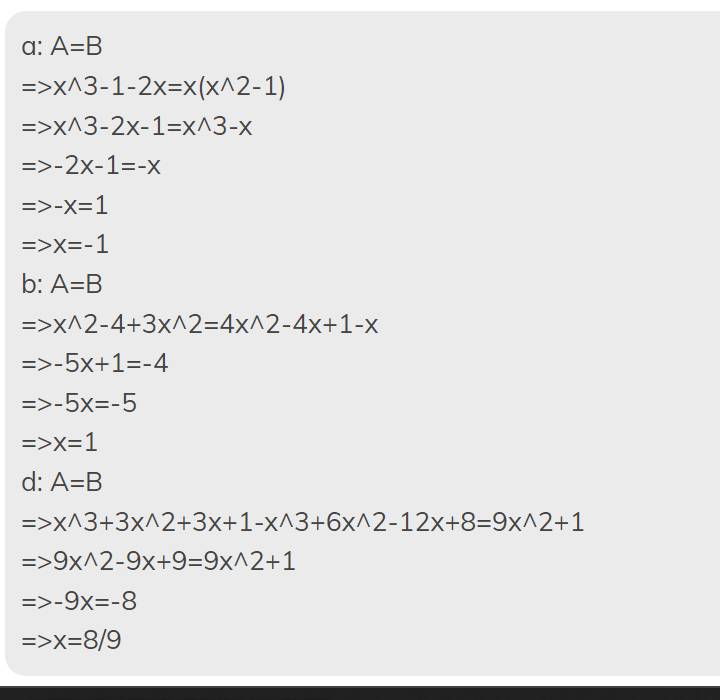
b) A= (x+2)(x-2)+3x² và B= (2x+1)² +2x
Ta có:
\(\left(x+2\right)\left(x-2\right)+3x^2=\left(2x+1\right)^2+2x\)
=> \(4x^2-4=4x^2+6x+1\)
=>6x=−5
=>\(x=-\frac{5}{6}\)
c) A= (x-1)(x²+x+1)-2x và B= x(x-1)(x+1)
ta có:
\(\left(x-1\right)\left(x^2+x+1\right)-2x=x\left(x+1\right)\left(x+1\right)\)
=>\(x^3-1-2x=x\left(x^2-1\right)\)
=>\(x^3-1-2x=x^3-x\)
=>\(x=-1\)
d) A= (x+1)³ -(x-2)³ và B= (3x-1)(3x+1)
ta có:
\(\left(x+1\right)^3-\left(x-2\right)^3=\left(3x-1\right)\left(3x+1\right)\)
=>\(9x^2+9x+9=9x^2-1\)
=>\(9x=10\)
=>x=\(\frac{10}{9}\)
chúc bạn học tốt
#Nghi

a: Để \(\dfrac{3x-2}{4}\) không nhỏ hơn \(\dfrac{3x+3}{6}\) thì \(\dfrac{3x-2}{4}>=\dfrac{3x+3}{6}\)
=>\(\dfrac{6\left(3x-2\right)}{24}>=\dfrac{4\left(3x+3\right)}{24}\)
=>18x-12>=12x+12
=>6x>=24
=>x>=4
b: Để \(\left(x+1\right)^2\) nhỏ hơn \(\left(x-1\right)^2\) thì \(\left(x+1\right)^2< \left(x-1\right)^2\)
=>\(x^2+2x+1< x^2-2x+1\)
=>4x<0
=>x<0
c: Để \(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}\) không lớn hơn \(\dfrac{x^2}{7}-\dfrac{2x-3}{5}\) thì
\(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}< =\dfrac{x^2}{7}-\dfrac{2x-3}{5}\)
=>\(\dfrac{2x-3+5x\left(x-2\right)}{35}< =\dfrac{5x^2-7\cdot\left(2x-3\right)}{35}\)
=>\(2x-3+5x^2-10x< =5x^2-14x+21\)
=>-8x-3<=-14x+21
=>6x<=24
=>x<=4