Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(f'\left(x\right)=3\left(m-1\right)x^2+4\left(m-1\right)x+m\)
- Với \(m=1\Rightarrow f'\left(x\right)=1>0\) (không thỏa mãn)
- Với \(m\ne1\Rightarrow f'\left(x\right)< 0;\forall x\) khi và chỉ khi:
\(\left\{{}\begin{matrix}\Delta'=4\left(m-1\right)^2-3m\left(m-1\right)< 0\\m-1< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}1< m< 4\\m< 1\end{matrix}\right.\)
\(\Rightarrow\) Không tồn tại m thỏa mãn yêu cầu

Bài 1:
Cho $y=0$ thì: $f(x^3)=xf(x^2)$
Tương tự khi cho $x=0$
$\Rightarrow f(x^3-y^3)=xf(x^2)-yf(y^2)=f(x^3)-f(y^3)$
$\Rightarrow f(x-y)=f(x)-f(y)$ với mọi $x,y\in\mathbb{R}$
Cho $x=0$ thì $f(-y)=0-f(y)=-f(y)$
Cho $y\to -y$ thì: $f(x+y)=f(x)-f(-y)=f(x)--f(y)=f(x)+f(y)$ với mọi $x,y\in\mathbb{R}$
Đến đây ta có:
$f[(x+1)^3+(x-1)^3]=f(2x^3+6x)=f(2x^3)+f(6x)$
$=2f(x^3)+6f(x)=2xf(x^2)+6f(x)$
$f[(x+1)^3+(x-1)^3]=f[(x+1)^3-(1-x)^3]$
$=(x+1)f((x+1)^2)-(1-x)f((1-x)^2)$
$=(x+1)f(x^2+2x+1)+(x-1)f(x^2-2x+1)$
$=(x+1)[f(x^2)+2f(x)+f(1)]+(x-1)[f(x^2)-2f(x)+f(1)]$
$=2xf(x^2)+4f(x)+2xf(1)$
Do đó:
$2xf(x^2)+6f(x)=2xf(x^2)+4f(x)+2xf(1)$
$2f(x)=2xf(1)$
$f(x)=xf(1)=ax$ với $a=f(1)$

\(f\left(x^5+y^5+y\right)=x^3f\left(x^2\right)+y^3f\left(y^2\right)+f\left(y\right)\)
Sửa lại đề câu 2 !!

1.
\(f'\left(x\right)=3x^2-6mx+3\left(2m-1\right)\)
\(f'\left(x\right)-6x=3x^2-3.2\left(m+1\right)x+3\left(2m-1\right)>0\)
\(\Leftrightarrow x^2-2\left(m+1\right)x+2m-1>0\)
\(\Leftrightarrow x^2-2x-1>2m\left(x-1\right)\)
Do \(x>2\Rightarrow x-1>0\) nên BPT tương đương:
\(\dfrac{x^2-2x-1}{x-1}>2m\Leftrightarrow\dfrac{\left(x-1\right)^2-2}{x-1}>2m\)
Đặt \(t=x-1>1\Rightarrow\dfrac{t^2-2}{t}>2m\Leftrightarrow f\left(t\right)=t-\dfrac{2}{t}>2m\)
Xét hàm \(f\left(t\right)\) với \(t>1\) : \(f'\left(t\right)=1+\dfrac{2}{t^2}>0\) ; \(\forall t\Rightarrow f\left(t\right)\) đồng biến
\(\Rightarrow f\left(t\right)>f\left(1\right)=-1\Rightarrow\) BPT đúng với mọi \(t>1\) khi \(2m< -1\Rightarrow m< -\dfrac{1}{2}\)
2.
Thay \(x=0\) vào giả thiết:
\(f^3\left(2\right)-2f^2\left(2\right)=0\Leftrightarrow f^2\left(2\right)\left[f\left(2\right)-2\right]=0\Rightarrow\left[{}\begin{matrix}f\left(2\right)=0\\f\left(2\right)=2\end{matrix}\right.\)
Đạo hàm 2 vế giả thiết:
\(-3f^2\left(2-x\right).f'\left(2-x\right)-12f\left(2+3x\right).f'\left(2+3x\right)+2x.g\left(x\right)+x^2.g'\left(x\right)+36=0\) (1)
Thế \(x=0\) vào (1) ta được:
\(-3f^2\left(2\right).f'\left(2\right)-12f\left(2\right).f'\left(2\right)+36=0\)
\(\Leftrightarrow f^2\left(2\right).f'\left(2\right)+4f\left(2\right).f'\left(2\right)-12=0\) (2)
Với \(f\left(2\right)=0\) thế vào (2) \(\Rightarrow-12=0\) ko thỏa mãn (loại)
\(\Rightarrow f\left(2\right)=2\)
Thế vào (2):
\(4f'\left(2\right)+8f'\left(2\right)-12=0\Leftrightarrow f'\left(2\right)=1\)
\(\Rightarrow A=3.2+4.1\)

\(f'\left(x\right)=2ax+b\)
\(f\left(x\right)+\left(x-1\right)f'\left(x\right)=ax^2+bx+c+\left(x-1\right)\left(2ax+b\right)\)
\(=3ax^2+\left(2b-2a\right)x+c-b\)
Yêu cầu bài toán thỏa mãn khi: \(\left\{{}\begin{matrix}3a=3\\2b-2a=0\\c-b=0\end{matrix}\right.\) \(\Leftrightarrow a=b=c=1\)

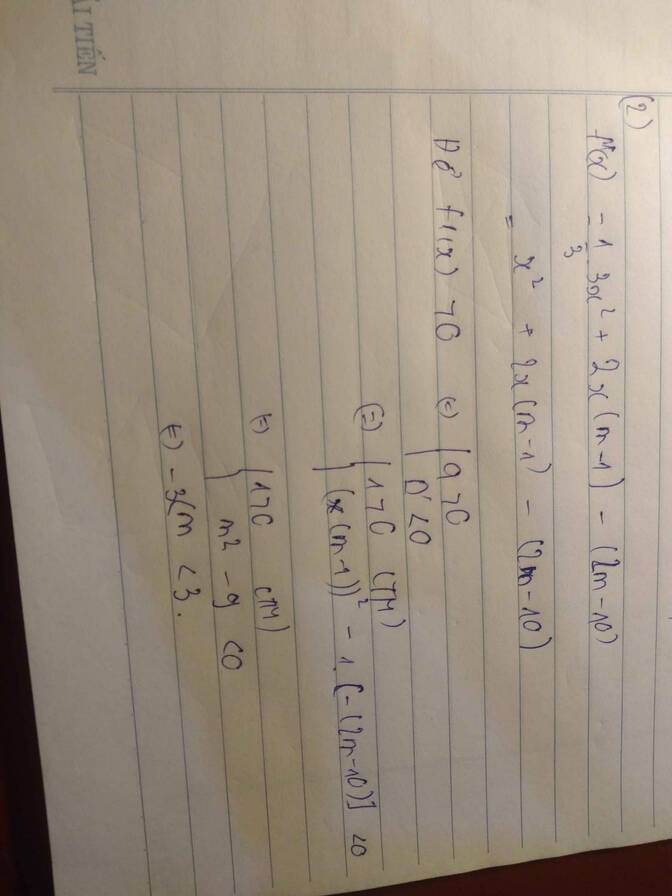
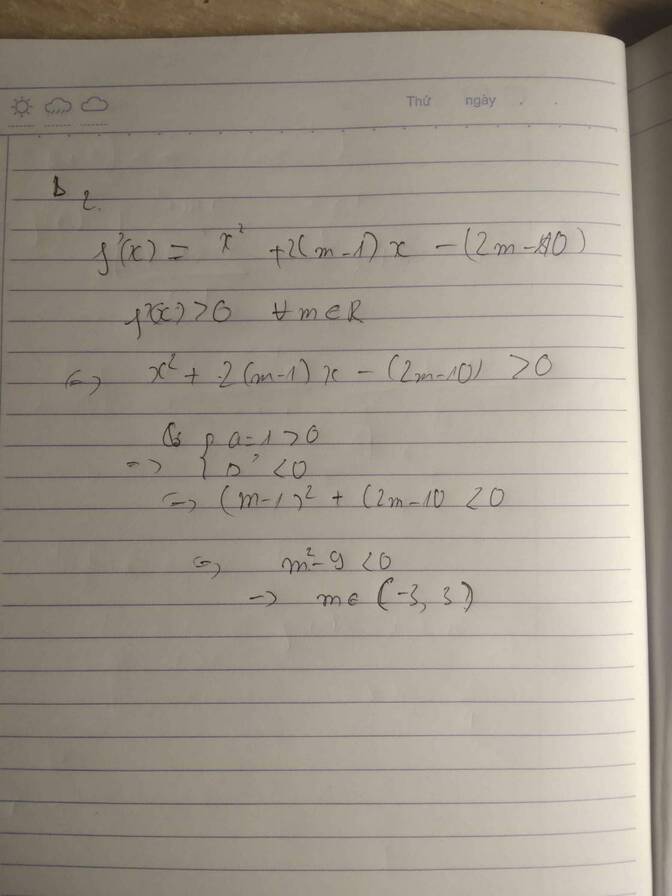
Bài này thiếu dữ kiện ; không cho P(x) là đa thức bậc mấy ?