K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
21 tháng 8 2018
cos2(x + kπ) = cos(2x + k2π) = cos2x, k ∈ Z.
Vậy hàm số y = cos 2x là hàm số chẵn, tuần hoàn, có chu kì là π.
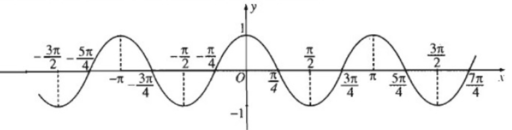
Đồ thị hàm số y = cos2x
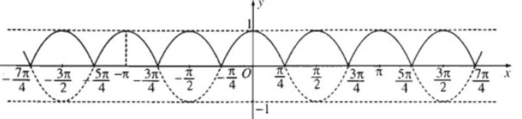
Đồ thị hàm số y = |cos2x|
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 6 2021
1.
ĐKXĐ: \(x\ne k\pi\)
\(\Leftrightarrow\left(2cos2x-1\right)\left(sinx-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=\dfrac{1}{2}\\sinx=3>1\left(ktm\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{3}+k2\pi\\2x=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k\pi\\x=-\dfrac{\pi}{6}+k\pi\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 6 2021
2. Bạn kiểm tra lại đề, pt này về cơ bản ko giải được.
3.
ĐKXĐ: \(x\ne\dfrac{k\pi}{2}\)
\(\dfrac{3\left(sinx+\dfrac{sinx}{cosx}\right)}{\dfrac{sinx}{cosx}-sinx}-2cosx=2\)
\(\Leftrightarrow\dfrac{3\left(1+cosx\right)}{1-cosx}+2\left(1+cosx\right)=0\)
\(\Leftrightarrow\left(1+cosx\right)\left(\dfrac{3}{1-cosx}+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=-1\left(loại\right)\\cosx=\dfrac{5}{2}\left(loại\right)\end{matrix}\right.\)
Vậy pt đã cho vô nghiệm
21 tháng 7 2022
a: \(\sqrt{3^2+2^2}=\sqrt{13}\)
Chia hai vế cho căn 13, ta được:
\(\dfrac{3}{\sqrt{13}}\cdot\sin2x+\dfrac{2}{\sqrt{13}}\cdot\cos2x=\dfrac{3}{\sqrt{13}}\)
Đặt \(\cos a=\dfrac{3}{\sqrt{13}}\)
Ta được phương trình: \(\sin\left(2x+a\right)=\cos a=\sin\left(\dfrac{\Pi}{2}-a\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+a=\dfrac{\Pi}{2}-a+k2\Pi\\2x+a=\dfrac{\Pi}{2}+a+k2\Pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\left(\dfrac{\Pi}{2}-2a+k2\Pi\right)\\x=\dfrac{\Pi}{4}+k\Pi\end{matrix}\right.\)
b: \(\Leftrightarrow cos^2x-sin^2x+cosx-sinx=0\)
\(\Leftrightarrow\left(cosx-sinx\right)\left(cosx+sinx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\cos x=\cos\left(\dfrac{\Pi}{2}-x\right)\\\sin\left(x-\dfrac{\Pi}{4}\right)=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Pi}{2}-x+k2\Pi\\x=-\dfrac{\Pi}{2}+x+k2\Pi\\x-\dfrac{\Pi}{4}=-\dfrac{\Pi}{2}+k2\Pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Pi}{4}+k\Pi\\x=-\dfrac{\Pi}{4}+k2\Pi\end{matrix}\right.\)
PT
1
CM
26 tháng 8 2019
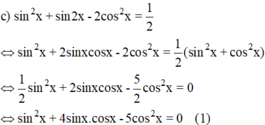
+ Xét cos x = 0 ⇒ sin2x = 1 – cos2x = 1
(1) trở thành 1 = 0 (Vô lý).
+ Xét cos x ≠ 0, chia cả hai vế cho cos2x ta được:
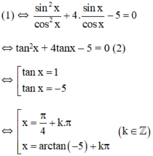
Vậy phương trình có tập nghiệm
(k ∈ Z)
3 tháng 7 2021
a) Pt \(\Leftrightarrow3.cos4x-\left(cos6x+1\right)=1\)
\(\Leftrightarrow3cos4x-cos6x-2=0\)
Đặt \(t=2x\)
Pttt:\(3cos2t-cos3t-2=0\)
\(\Leftrightarrow3\left(2cos^2t-1\right)-\left(4cos^3t-3cost\right)-2=0\)
\(\Leftrightarrow-4cos^3t+6cos^2t+3cost-5=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cost=1\\cost=\dfrac{1+\sqrt{21}}{4}\left(vn\right)\\cost=\dfrac{1-\sqrt{21}}{4}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}t=k2\pi\\t=\pm arc.cos\left(\dfrac{1-\sqrt{21}}{4}\right)+k2\pi\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=\pm\dfrac{1}{2}.arccos\left(\dfrac{1-\sqrt{21}}{4}\right)+k\pi\end{matrix}\right.\) (\(k\in Z\))
Vậy...
a2) \(2cos2x-8cosx+7=\dfrac{1}{cosx}\) (ĐK: \(x\ne\dfrac{\pi}{2}+k\pi\))
\(\Leftrightarrow2.\left(2cos^2x-1\right)-8cosx+7=\dfrac{1}{cosx}\)
\(\Leftrightarrow2.\left(2cos^2x-1\right)cosx-8cos^2x+7cosx=1\)
\(\Leftrightarrow4cos^3x-8cos^2x+5cosx-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\cosx=\dfrac{1}{2}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=\pm\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\) (tm) (\(k\in Z\))
Vậy...
a3) Đk: \(x\ne-\dfrac{\pi}{4}+k\pi;x\ne\dfrac{\pi}{2}+k\pi\)
Pt \(\Leftrightarrow\dfrac{\left(1+sinx+1-2sin^2x\right).\dfrac{1}{\sqrt{2}}\left(sinx+cosx\right)}{1+\dfrac{sinx}{cosx}}=\dfrac{1}{\sqrt{2}}cosx\)
\(\Leftrightarrow\dfrac{\left(-2sin^2x+sinx+2\right).\left(sinx+cosx\right)cosx}{cosx+sinx}=cosx\)
\(\Leftrightarrow\left(2+sinx-2sin^2x\right).cosx=cosx\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\left(ktm\right)\\2+sinx-2sin^2x=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}sinx=1\\sinx=-\dfrac{1}{2}\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}cosx=0\left(ktm\right)\\sinx=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{6}+k2\pi\\x=\dfrac{7\pi}{6}+k2\pi\end{matrix}\right.\) (\(k\in Z\))
Vậy...
3 tháng 7 2021
a4) Pt \(\Leftrightarrow9sinx+6cosx-6sinx.cosx+1-2sin^2x=8\)
\(\Leftrightarrow6cosx\left(1-sinx\right)-\left(2sin^2x-9sinx+7\right)=0\)
\(\Leftrightarrow6cosx\left(1-sinx\right)-\left(2sinx-7\right)\left(sinx-1\right)=0\)
\(\Leftrightarrow\left(1-sinx\right)\left(6cosx+2sinx+7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=1\\6cosx+2sinx=7\left(vn\right)\end{matrix}\right.\) (\(6cosx+2sinx=7\) vô nghiệm do \(6^2+2^2< 7^2\))
\(\Rightarrow sinx=1\)
\(\Leftrightarrow x=\dfrac{\pi}{2}+k2\pi;k\in Z\)
Vậy...
NV
Nguyễn Việt Lâm
Giáo viên
29 tháng 7 2021
a.
\(\left(sin^2x+cos^2x\right)^3-3sin^2x.cos^2x\left(sin^2x+cos^2x\right)=cos2x+\dfrac{1}{16}\)
\(\Leftrightarrow1-\dfrac{3}{4}sin^22x=cos2x+\dfrac{1}{16}\)
\(\Leftrightarrow\dfrac{15}{16}-\dfrac{3}{4}\left(1-cos^22x\right)=cos2x\)
\(\Leftrightarrow\dfrac{3}{4}cos^22x-cos2x+\dfrac{3}{16}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=\dfrac{4-\sqrt{7}}{6}\\cos2x=\dfrac{4+\sqrt{7}}{6}>1\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow x=\pm\dfrac{1}{2}arccos\left(\dfrac{4-\sqrt{7}}{6}\right)+k\pi\)
NV
Nguyễn Việt Lâm
Giáo viên
29 tháng 7 2021
b.
\(\left(sin^2\dfrac{x}{2}+cos^2\dfrac{x}{2}\right)^2-2sin^2\dfrac{x}{2}cos^2\dfrac{x}{2}=\dfrac{5}{2}-2sinx\)
\(\Leftrightarrow1-\dfrac{1}{2}sin^2x=\dfrac{5}{2}-2sinx\)
\(\Leftrightarrow\dfrac{1}{2}sin^2x-2sinx+\dfrac{3}{2}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=1\\sinx=3\left(loại\right)\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{2}+k2\pi\)
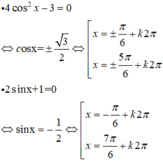
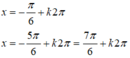
\(cos^2x=\dfrac{1}{2}cos2x+\dfrac{1}{2}\) mới đúng
Nó là công thức hạ bậc (có trong SGK đại số 10)