Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\dfrac{9x^2-6x+1}{9x^2-1}\)
\(=\dfrac{\left(3x-1\right)^2}{\left(3x-1\right)\left(3x+1\right)}\)
\(=\dfrac{3x-1}{3x+1}\)
\(=\dfrac{3\cdot\left(-3\right)-1}{3\cdot\left(-3\right)+1}=\dfrac{-9-1}{-9+1}=\dfrac{-10}{-8}=\dfrac{5}{4}\)
b) Ta có: \(\dfrac{x^2-6x+9}{3x^2-9x}\)
\(=\dfrac{\left(x-3\right)^2}{3x\left(x-3\right)}\)
\(=\dfrac{x-3}{3x}\)
\(=\dfrac{-\dfrac{1}{3}-3}{3\cdot\dfrac{-1}{3}}=\dfrac{-\dfrac{10}{3}}{-1}=\dfrac{10}{3}\)
c) Ta có: \(\dfrac{x^2-4x+4}{2x^2-4x}\)
\(=\dfrac{\left(x-2\right)^2}{2x\left(x-2\right)}\)
\(=\dfrac{x-2}{2x}\)
\(=\dfrac{\dfrac{-1}{2}-2}{2\cdot\dfrac{-1}{2}}=\dfrac{-\dfrac{5}{2}}{-1}=\dfrac{5}{2}\)

Bài 2:
a: Ta có: \(2\left(5x-8\right)-3\left(4x-5\right)=4\left(3x-4\right)+11\)
\(\Leftrightarrow10x-16-12x+15=12x-16+11\)
\(\Leftrightarrow-14x=-4\)
hay \(x=\dfrac{2}{7}\)
b: Ta có: \(2x\left(6x-2x^2\right)+3x^2\left(x-4\right)=8\)
\(\Leftrightarrow12x^2-4x^3+3x^3-12x^2=8\)
\(\Leftrightarrow x^3=-8\)
hay x=-2
Bài 1:
a: Ta có: \(I=x\left(y^2-xy^2\right)+y\left(x^2y-xy+x\right)\)
\(=xy^2-x^2y^2+x^2y^2-xy^2+xy\)
\(=xy\)
=1
b: Ta có: \(K=x^2\left(y^2+xy^2+1\right)-\left(x^3+x^2+1\right)\cdot y^2\)
\(=x^2y^2+x^3y^2+x^2-x^3y^2-x^2y^2-y^2\)
\(=x^2-y^2\)
\(=\dfrac{1}{4}-\dfrac{1}{4}=0\)

a) M = (x² + 3xy - 3x³) + (2y³ - xy + 3x³)
= x² + 3xy - 3x³ + 2y³ - xy + 3x³
= x² + (3xy - xy) + (-3x³ + 3x³) + 2y³
= x² + 2xy + 2y³
Tại x = 5 và y = 4
M = 5² + 2.5.4 + 2.4³
= 25 + 40 + 2.64
= 65 + 128
= 193
b) N = x²(x + y) - y(x² - y²)
= x³ + x²y - x²y + y³
= x³ + (x²y - x²y) + y³
= x³ + y³
Tại x = -6 và y = 8
N = (-6)³ + 8³
= -216 + 512
= 296
c) P = x² + 1/2 x + 1/16
= (x + 1/2)²
Tại x = 3/4 ta có:
P = (3/4 + 1/2)² = (5/4)² = 25/16

Bài 2:
a) Ta có: \(A=\left(7x+5\right)^2+\left(3x-5\right)^2-\left(10-6x\right)\left(5+7x\right)\)
\(=\left(7x+5\right)^2+2\cdot\left(7x+5\right)\cdot\left(3x-5\right)+\left(3x-5\right)^2\)
\(=\left(7x+5+3x-5\right)^2\)
\(=\left(10x\right)^2=100x^2\)
Thay x=-2 vào A, ta được:
\(A=100\cdot\left(-2\right)^2=100\cdot4=400\)
b) Ta có: \(B=\left(2x+y\right)\left(y^2-2xy+4x^2\right)-8x\left(x-1\right)\left(x+1\right)\)
\(=8x^3+y^3-8x\left(x^2-1\right)\)
\(=8x^3+y^3-8x^3+8x\)
\(=8x+y^3\)
Thay x=-2 và y=3 vào B, ta được:
\(B=-2\cdot8+3^3=-16+27=11\)

a) Ta có: \(\dfrac{3x^2-12x+12}{x^2-4}\)
\(=\dfrac{3\left(x^2-4x+4\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{3\left(x-2\right)^2}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{3\left(x-2\right)}{x+2}\)
\(=\dfrac{3\cdot\left(\dfrac{-1}{4}-2\right)}{\dfrac{-1}{4}+2}=-\dfrac{27}{7}\)
b) Ta có: \(\dfrac{x^2-5x-6}{x^2-9}\)
\(=\dfrac{\left(x-6\right)\left(x+1\right)}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{\left(-1-6\right)\left(-1+1\right)}{\left(-1-3\right)\left(-1+3\right)}\)
=0

Bạn chú ý đăng lẻ câu hỏi! 1/
a/ \(=x^3-2x^5\)
b/\(=5x^2+5-x^3-x\)
c/ \(=x^3+3x^2-4x-2x^2-6x+8=x^3=x^2-10x+8\)
d/ \(=x^2-x^3+4x-2x+2x^2-8=3x^2-x^3+2x-8\)
e/ \(=x^4-x^2+2x^3-2x\)
f/ \(=\left(6x^2+x-2\right)\left(3-x\right)=17x^2+5x-6-6x^3\)
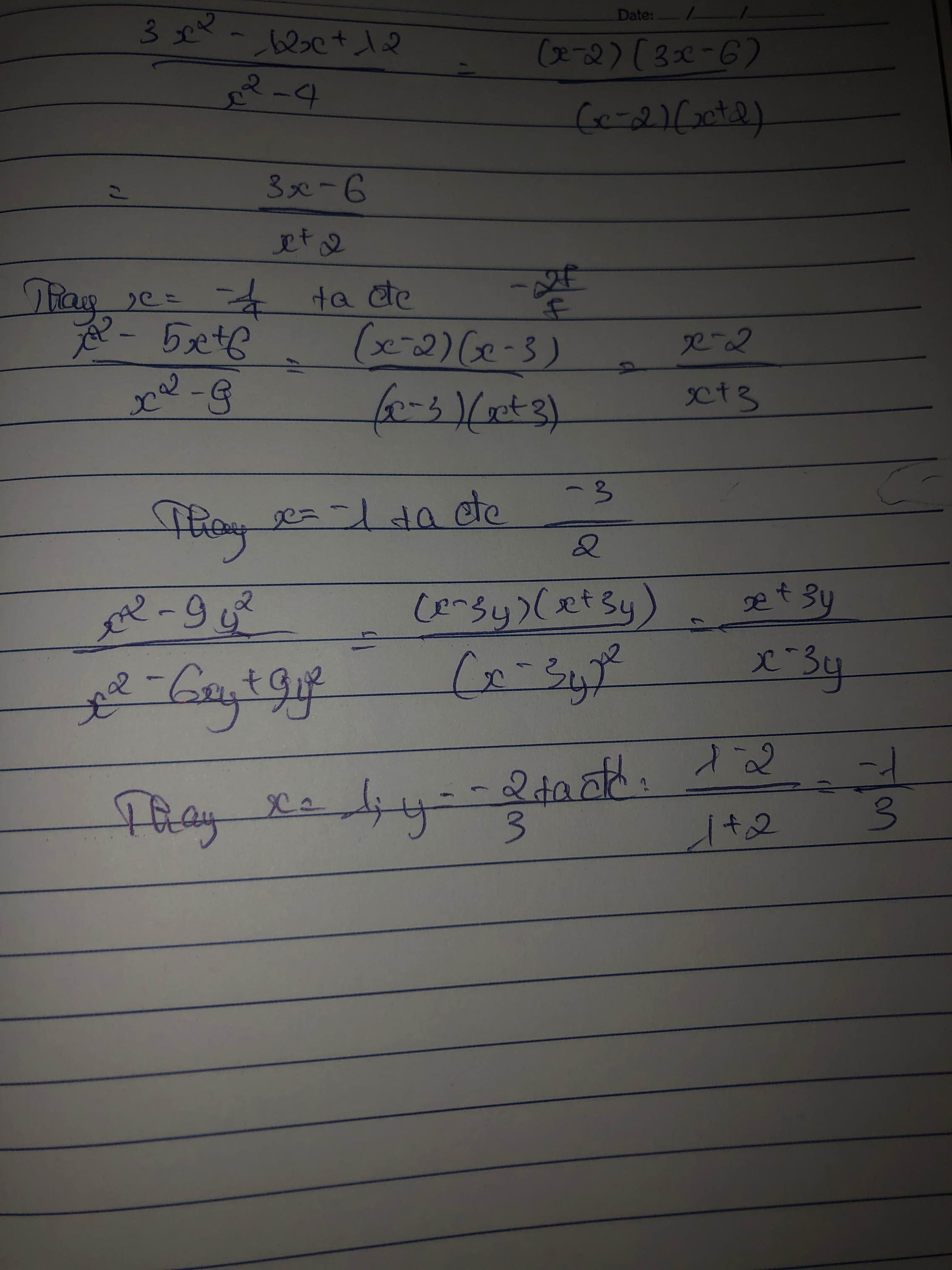
c) Ta có: \(C=4\left(3x-2\right)^2+\left(4-x\right)^2-\left(6x-4\right)\left(8-2x\right)\)
\(=4\left(9x^2-12x+4\right)+x^2-8x+16-\left(48x-12x^2-32+8x\right)\)
\(=36x^2-48x+16+x^2-8x+16-48x+12x^2+32-8x\)
\(=49x^2-112x+64\)
\(=\left(7x-8\right)^2\)
\(=\left(7\cdot149-8\right)^2\)
\(=1071225\)
d) \(\left(3x-4\right)^2-9\left(x-2\right)\left(x+2\right)\)
\(=9x^2-24x+16-9\left(x^2-4\right)\)
\(=9x^2-24x+16-9x^2+36\)
\(=-24x+52\)
\(=-24\cdot\left(-2\right)+52\)
=48+52=100
e) Ta có: \(x\left(x-3\right)^2-\left(x-1\right)\left(x+5\right)-x\left(x-2\right)\left(x+2\right)\)
\(=x\left(x^2-6x+9\right)-\left(x^2+4x-5\right)-x\left(x^2-4\right)\)
\(=x^3-6x^2+9x-x^2-4x+5-x^3+4x\)
\(=-7x^2+9x+5\)
\(=-7\cdot\left(-1\right)^2+9\cdot\left(-1\right)+5\)
\(=-7-9+5\)
=-16+5=-11