Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\sqrt{9a^4}=\sqrt{\left(3a^2\right)^2}=\left|3a^2\right|=3a^2\)
b) \(2\sqrt{a^2}-5a=2\left|a\right|-5a=-2a-5a=-7a\)
c) \(\sqrt{16\left(1+4x+4x^2\right)}=\sqrt{\left[4\left(1+2x\right)\right]^2}=\left|4\left(1+2x\right)\right|=4\left(1+2x\right)\)

Với a >= 0 ; a khác 9
\(P=\dfrac{2a-6\sqrt{a}+a+4\sqrt{a}+3-3-7\sqrt{a}}{a-9}=\dfrac{3a-9\sqrt{a}}{a-9}=\dfrac{3\sqrt{a}}{\sqrt{a}+3}\)
b, Để hàm số trêm là hàm bậc nhất khi a khác 0
Cho (d') : y = ax - 4 Để (d') cắt (d) khi a khác -3
Thay y = 5 vào (d) ta được <=> 5 = -3x + 2 <=> x = -1
(d) cắt (d') tại A(-1;5)
<=> 5 = -a - 4 <=> a = -9 (tm)

5: \(=\dfrac{1}{x-y}\cdot x^3\cdot\left(x-y\right)^2=x^3\left(x-y\right)\)

a) \(A=\dfrac{x\sqrt{y}+y\sqrt{x}}{x+2\sqrt{xy}+y}\)
\(A=\dfrac{\sqrt{xy}\left(\sqrt{x}+\sqrt{y}\right)}{\left(\sqrt{x}+\sqrt{y}\right)^2}\)
\(A=\dfrac{\sqrt{xy}}{\sqrt{x}+\sqrt{y}}\)
b) \(B=\dfrac{x\sqrt{y}-y\sqrt{x}}{x-2\sqrt{xy}+y}\)
\(B=\dfrac{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)}{\left(\sqrt{x}-\sqrt{y}\right)^2}\)
\(B=\dfrac{\sqrt{xy}}{\sqrt{x}-\sqrt{y}}\)
c) \(C=\dfrac{3\sqrt{a}-2a-1}{4a-4\sqrt{a}+1}\)
\(C=\dfrac{-\left(2a-3\sqrt{a}+1\right)}{\left(2\sqrt{a}\right)^2-2\sqrt{a}\cdot2\cdot1+1^2}\)
\(C=\dfrac{-\left(\sqrt{a}-1\right)\left(2\sqrt{a}-1\right)}{\left(2\sqrt{a}-1\right)^2}\)
\(C=\dfrac{-\sqrt{a}+1}{2\sqrt{a}-1}\)
d) \(D=\dfrac{a+4\sqrt{a}+4}{\sqrt{a}+2}+\dfrac{4-a}{\sqrt{a}-2}\)
\(D=\dfrac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}+\dfrac{\left(2-\sqrt{a}\right)\left(2+\sqrt{a}\right)}{\sqrt{a}-2}\)
\(D=\sqrt{a}+2-\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}{\sqrt{a}-2}\)
\(D=\left(\sqrt{a}+2\right)-\left(\sqrt{a}+2\right)\)
\(D=0\)

\(a,B=4\sqrt{x=1}-3\sqrt{x+1}+2\)\(\sqrt{x+1}+\sqrt{x+1}\)
\(=4\sqrt{x+1}\)
\(b,\)đưa về \(\sqrt{x+1}=4\Rightarrow x=15\)
a, Với \(x\ge-1\)
\(\Rightarrow B=4\sqrt{x+1}-3\sqrt{x+1}+2\sqrt{x+1}+\sqrt{x+1}\)
\(=4\sqrt{x+1}\)
b, Ta có B = 16 hay
\(4\sqrt{x+1}=16\Leftrightarrow\sqrt{x+1}=4\)bình phương 2 vế ta được
\(\Leftrightarrow x+1=16\Leftrightarrow x=15\)
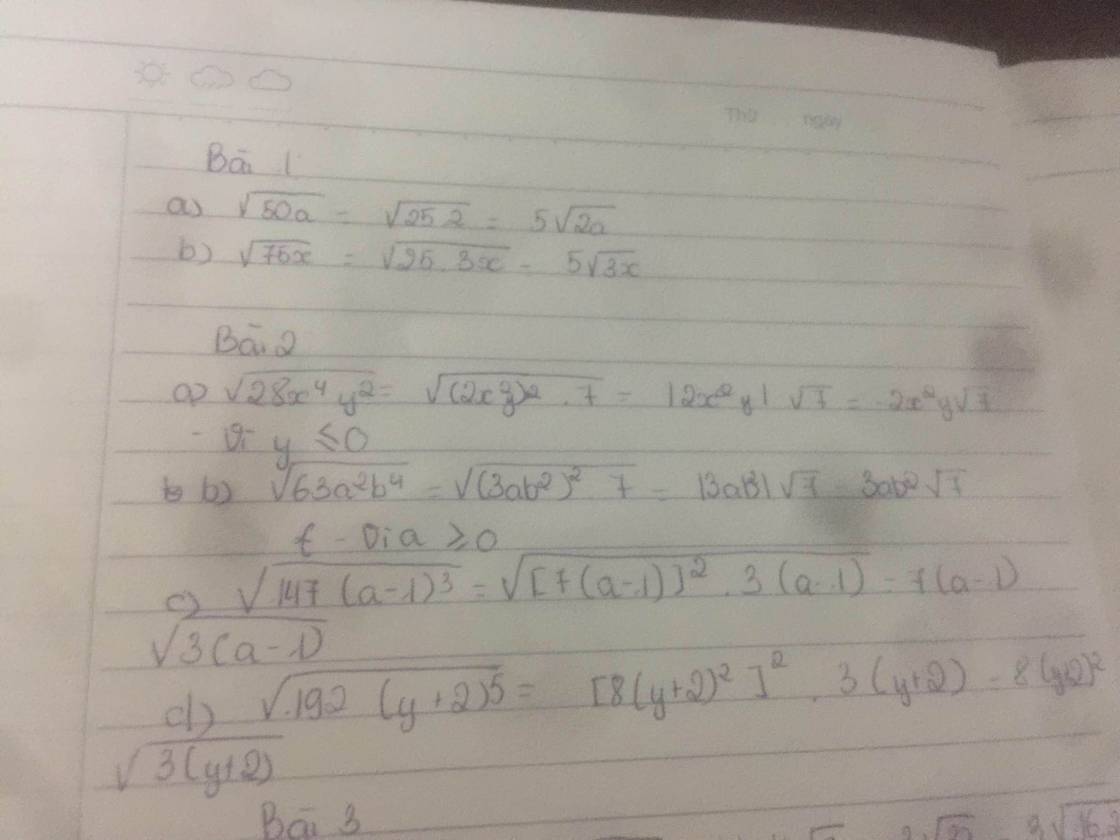

a) \(M=\sqrt{4\left(x-1\right)}-\sqrt{9\left(x-1\right)}-\sqrt{16\left(x-1\right)}\)
\(=2\sqrt{x-1}-3\sqrt{x-1}-4\sqrt{x-1}=-5\sqrt{x-1}\)
b) \(N=\sqrt{25\left(y+4\right)}+\sqrt{36\left(y+4\right)}-2\sqrt{81\left(y+4\right)}\)
\(=5\sqrt{y+4}+6\sqrt{y+4}-18\sqrt{y+4}=-7\sqrt{y+4}\)
c) \(P=\sqrt{y-2}-3\sqrt{64\left(y-2\right)}+4\sqrt{49\left(y-2\right)}\)
\(=\sqrt{y-2}-24\sqrt{y-2}+28\sqrt{y-2}=5\sqrt{y-2}\)
a) \(M=\sqrt{4\left(x-1\right)}-\sqrt{9\left(x-1\right)}-\sqrt{16\left(x-1\right)}.\)
\(M=\sqrt{4\left(x-1\right)}-\sqrt{9\left(x-1\right)}-\sqrt{16\left(x-1\right)}\)
\(=2\sqrt{x-1}-3\sqrt{x-1}-4\sqrt{x-1}\)
\(=-5\sqrt{x-1}\)
b) \(N=\sqrt{25\left(y+4\right)}+\sqrt{36\left(y+4\right)}-2\sqrt{81\left(y+4\right)}\)
\(N=\sqrt{25\left(y+4\right)}+\sqrt{36\left(y+4\right)}-2\sqrt{81\left(y+4\right)}\)
\(=5\sqrt{y+4}+6\sqrt{y+4}\)
\(=-7\sqrt{y+4}\)
c) \(P=\sqrt{\left(y-2\right)}-3\sqrt{64\left(y-2\right)}+4\sqrt{49\left(y-2\right)}\)
\(P=\sqrt{\left(y-2\right)}-3\sqrt{64\left(y-2\right)}+4\sqrt{49\left(y-2\right)}\)
\(=\sqrt{y-2}-24\sqrt{y-2}+28\sqrt{y-2}\)
\(=5\sqrt{y-2}\)