
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

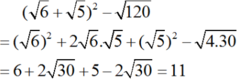

9√6 + 3√6 - √6 = 11√6
c) (√28 - 2√3 + √7)√7 + √84
= (√4.7 - 2√3 + √7)√7 + √4.21
= (2√7 - 2√3 + √7)√7 + 2√21
= (3√7 - 2√3)√7 + 2√21
= 3.7 - 2√21 + 2√21 = 21
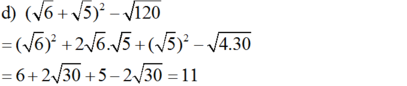

Đặt \(x=\sqrt{\dfrac{5+2\sqrt{6}}{5-\sqrt{6}}}+\sqrt{\dfrac{5-2\sqrt{6}}{5+\sqrt{6}}}>0\)
\(x^2=\dfrac{5+2\sqrt{6}}{5-\sqrt{6}}+\dfrac{5-2\sqrt{6}}{5+\sqrt{6}}+2\sqrt{\dfrac{25-24}{25-6}}=\dfrac{74}{19}+\dfrac{2\sqrt{19}}{19}\)
\(\Rightarrow x^2=\dfrac{74+2\sqrt{19}}{19}\Rightarrow x=\sqrt{\dfrac{74+2\sqrt{19}}{19}}\)
Ko thể rút gọn thêm nữa (có thể trục căn thức ở mẫu)

1.\(A=\left(\sqrt{3}+1\right)\sqrt{\dfrac{14-6\sqrt{3}}{5+\sqrt{3}}}=\left(\sqrt{3}+1\right)\sqrt{\dfrac{\left(14-6\sqrt{3}\right)\left(5-\sqrt{3}\right)}{\left(5+\sqrt{3}\right)\left(5-\sqrt{3}\right)}}\)
\(=\left(\sqrt{3}+1\right)\sqrt{\dfrac{44\left(2-\sqrt{3}\right)}{22}}=\left(\sqrt{3}+1\right)\sqrt{4-2\sqrt{3}}=\left(\sqrt{3}+1\right)\sqrt{\left(\sqrt{3}-1\right)^2}\)
\(=\left(\sqrt{3}+1\right)\left(\sqrt{3}-1\right)=2\)
2.1.a) \(x^2=\left(x-1\right)\left(3x-2\right)\Leftrightarrow x^2=3x^2-5x+2\Leftrightarrow2x^2-5x+2=0\)
\(\Leftrightarrow\left(x-2\right)\left(2x-1\right)=0\Rightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{1}{2}\end{matrix}\right.\)
b) \(9x^4+5x^2-4=0\Leftrightarrow9x^4+9x^2-4x^2-4=0\)
\(\Leftrightarrow9x^2\left(x^2+1\right)-4\left(x^2+1\right)=0\Leftrightarrow\left(x^2+1\right)\left(9x^2-4\right)=0\)
mà \(x^2+1>0\Rightarrow9x^2=4\Rightarrow x^2=\dfrac{4}{9}\Rightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-\dfrac{2}{3}\end{matrix}\right.\)
2) Gọi số xe lúc đầu của đội là a(xe) \(\left(a\in N,a>0\right)\)
Theo đề,ta có: \(\left(a-2\right)\left(\dfrac{120}{a}+3\right)=120\Leftrightarrow120+3a-\dfrac{240}{a}-6=120\)
\(\Leftrightarrow\dfrac{3a^2-6a-240}{a}=0\Rightarrow3a^2-6a-240=0\Rightarrow a^2-2a-80=0\)
\(\Leftrightarrow\left(a+8\right)\left(a-10\right)=0\) mà \(a>0\Rightarrow a=10\)

Ta có: \(\left(\sqrt{12}-2\sqrt{18}+5\sqrt{3}\right)\cdot\sqrt{3}+5\sqrt{6}\)
\(=\left(2\sqrt{3}-6\sqrt{3}+5\sqrt{3}\right)\cdot\sqrt{3}+5\sqrt{6}\)
\(=3+5\sqrt{6}\)

\(a,=\dfrac{\sqrt{2}\left(\sqrt{2}+1\right)}{\sqrt{2}+1}=\sqrt{2}\\ b,=\dfrac{\sqrt{5}\left(\sqrt{3}-1\right)}{1-\sqrt{3}}=-\sqrt{5}\\ c,=\dfrac{\sqrt{6}\left(\sqrt{2}-1\right)}{2\left(\sqrt{2}-1\right)}=\dfrac{\sqrt{6}}{2}\)

√(15 - 6√6)
=√(9-2.3.√6+6)
=√(3-√6)^2=3-√6
Chúc các bn hok tốt

Vì đây toàn là số cụ thể rồi nên không có đkxđ bạn nhé.
Lời giải:
a.
$=\sqrt{2}+4\sqrt{2}+6\sqrt{2}-3\sqrt{2}=8\sqrt{2}$
b.
$=\frac{13(5-2\sqrt{3})}{(5+2\sqrt{3})(5-2\sqrt{3})}+2\sqrt{3}=\frac{13(5-2\sqrt{3})}{13}+2\sqrt{3}$
$=5-2\sqrt{3}+2\sqrt{3}=5$
c.
$=2\sqrt{5}-|2-\sqrt{5}|=2\sqrt{5}-(\sqrt{5}-2)=\sqrt{5}+2$

\(A=\sqrt{5-2\sqrt{6}}-\sqrt{\left(\sqrt{2}-\sqrt{3}\right)^2}\)
\(=\sqrt{3}-\sqrt{2}-\sqrt{3}+\sqrt{2}\)
=0