Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, \(A=\dfrac{4\left(3-\sqrt{7}\right)}{2}+2\sqrt{7}=\dfrac{12}{2}=6\)
b, \(B=\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{2}{\sqrt{x}}\right):\dfrac{2-\sqrt{x}}{x-1}\)
\(=\left(\dfrac{\sqrt{x}-2\sqrt{x}+2}{\sqrt{x}\left(\sqrt{x}-1\right)}\right):\dfrac{2-\sqrt{x}}{x-1}=\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
nhờ bạn làm rõ vì sao \(\dfrac{\sqrt{x}-2\sqrt{x}+2}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{2-\sqrt{x}}{x-1}\) lại bằng \(\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
mình xin cảm ơn

Lời giải:
$A=\frac{10\sqrt{x}}{(\sqrt{x}-1)(\sqrt{x}+4)}-\frac{(2\sqrt{x}-3)(\sqrt{x}-1)}{(\sqrt{x}+4)(\sqrt{x}-1)}-\frac{(\sqrt{x}+1)(\sqrt{x}+4)}{(\sqrt{x}-1)(\sqrt{x}+4)}$
$=\frac{10\sqrt{x}-(2\sqrt{x}-3)(\sqrt{x}-1)-(\sqrt{x}+1)(\sqrt{x}+4)}{(\sqrt{x}+4)(\sqrt{x}-1)}$
$=\frac{-3x+10\sqrt{x}-7}{(\sqrt{x}+4)(\sqrt{x}-1)}$
$=\frac{-(\sqrt{x}-1)(3\sqrt{x}-7)}{(\sqrt{x}+4)(\sqrt{x}-1)}=\frac{7-3\sqrt{x}}{\sqrt{x}+4}$

`A=(2\sqrtx-9)(x-5sqrtx+6)-(sqrtx+3)/(sqrtx-2)-(2sqrtx+1)(3-sqrtx)(x>=0,x ne 4, x ne 9)`
`=(2\sqrtx-9)(x-5sqrtx+6)-(sqrtx+3)/(sqrtx-2)+(2sqrtx+1)(sqrtx-3)`
`=(2sqrtx-9-x+9+2x-3sqrtx-2)/(x-5sqrtx+6)`
`=(x-sqrtx-2)/(x-5sqrtx+6)`
`=((\sqrtx+1)(sqrtx-2))/((sqrtx-2)(sqrtx-3))`
`=(sqrtx+1)/(sqrtx-3)`
`A=(2\sqrtx-9)/(x-5sqrtx+6)-(sqrtx+3)/(sqrtx-2)-(2sqrtx+1)/(3-sqrtx)(x>=0,x ne 4, x ne 9)`
`=(2\sqrtx-9)/(x-5sqrtx+6)-(sqrtx+3)/(sqrtx-2)+(2sqrtx+1)/(sqrtx-3)`
`=(2sqrtx-9-x+9+2x-3sqrtx-2)/(x-5sqrtx+6)`
`=(x-sqrtx-2)/(x-5sqrtx+6)`
`=((\sqrtx+1)(sqrtx-2))/((sqrtx-2)(sqrtx-3))`
`=(sqrtx+1)/(sqrtx-3)`

Sửa đề: \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right):\dfrac{\sqrt{x}}{\sqrt{x}+1}\)
Ta có: \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right):\dfrac{\sqrt{x}}{\sqrt{x}+1}\)
\(=\left(\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}-\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}\right):\dfrac{\sqrt{x}}{\sqrt{x}+1}\)
\(=\dfrac{x+\sqrt{x}-2-x+\sqrt{x}+2}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}:\dfrac{\sqrt{x}}{\sqrt{x}+1}\)
\(=\dfrac{2\sqrt{x}}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
\(=\dfrac{2}{x-1}\)

a) \(P=\dfrac{1}{2-\sqrt{3}}+\dfrac{1}{2+\sqrt{3}}\)
\(=\dfrac{2+\sqrt{3}}{\left(2-\sqrt{3}\right)\left(2+\sqrt{3}\right)}+\dfrac{2-\sqrt{3}}{\left(2-\sqrt{3}\right)\left(2+\sqrt{3}\right)}\)
\(=\dfrac{2+\sqrt{3}+2-\sqrt{3}}{\left(2-\sqrt{3}\right)\left(2+\sqrt{3}\right)}\)
\(=\dfrac{4}{4-3}\)
\(=4\)
b) \(Q=\left(1+\dfrac{\sqrt{x}+2}{\sqrt{x}-2}\right).\dfrac{1}{\sqrt{x}}vớix>0,x\ne4\)
\(=\left(\dfrac{\sqrt{x}-2+\sqrt{x}+2}{\sqrt{x}-2}\right).\dfrac{1}{\sqrt{x}}\)
\(=\)\(\dfrac{2\sqrt{x}}{\sqrt{x}-2}.\dfrac{1}{\sqrt{x}}\)
\(=\dfrac{2\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(=\dfrac{2}{\sqrt{x}-2}\)

\(=>B=\dfrac{\left(\sqrt{x}-1\right)\sqrt{x}-5\sqrt{x}+8}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{x-\sqrt{x}-5\sqrt{x}+8}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{x-6\sqrt{x}+8}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{\left(\sqrt{x}-4\right)\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}-4}{\sqrt{x}}\)
Ta có: \(B=\dfrac{\sqrt{x}-1}{\sqrt{x}-2}-\dfrac{5\sqrt{x}-8}{x-2\sqrt{x}}\)
\(=\dfrac{x-\sqrt{x}-5\sqrt{x}+8}{\sqrt{x}\cdot\left(\sqrt{x}-2\right)}\)
\(=\dfrac{x-6\sqrt{x}+8}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}-4}{\sqrt{x}-2}\)

\(a,P=\dfrac{\sqrt{x}+2+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\cdot\dfrac{2-\sqrt{x}}{\sqrt{x}}=\dfrac{-2\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}=\dfrac{-2}{\sqrt{x}+2}\\ P=-\dfrac{3}{5}\Leftrightarrow\dfrac{2}{\sqrt{x}+2}=\dfrac{3}{5}\\ \Leftrightarrow3\sqrt{x}+6=10\Leftrightarrow\sqrt{x}=\dfrac{4}{3}\Leftrightarrow x=\dfrac{16}{9}\left(tm\right)\)
\(P=-\dfrac{3}{5}\) sao suy ra đc \(\dfrac{2}{\sqrt{x}+2}=\dfrac{3}{5}\) thế

Ta có : \(B=\left(\dfrac{\sqrt{x}}{\sqrt{x}+1}-\dfrac{1}{x+\sqrt{x}}\right)\left(\dfrac{1}{\sqrt{x}+1}+\dfrac{2}{x-1}\right)\)
\(=\left(\dfrac{\sqrt{x}}{\sqrt{x}+1}-\dfrac{1}{\sqrt{x}\left(\sqrt{x}+1\right)}\right)\left(\dfrac{1}{\sqrt{x}+1}+\dfrac{2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
\(=\left(\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}+1\right)}\right)\left(\dfrac{\sqrt{x}-1+2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
\(=\left(\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}\right)\left(\dfrac{\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
\(=\left(\dfrac{\sqrt{x}-1}{\sqrt{x}}\right)\left(\dfrac{1}{\sqrt{x}-1}\right)=\dfrac{1}{\sqrt{x}}\)
B = \(\left[\dfrac{\sqrt{x}}{\sqrt{x}+1}-\dfrac{1}{\sqrt{x}\left(\sqrt{x}+1\right)}\right].\left(\dfrac{1}{\sqrt{x}+1}+\dfrac{2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
= \(\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}+1\right)}.\dfrac{\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{1}{\sqrt{x}}\)




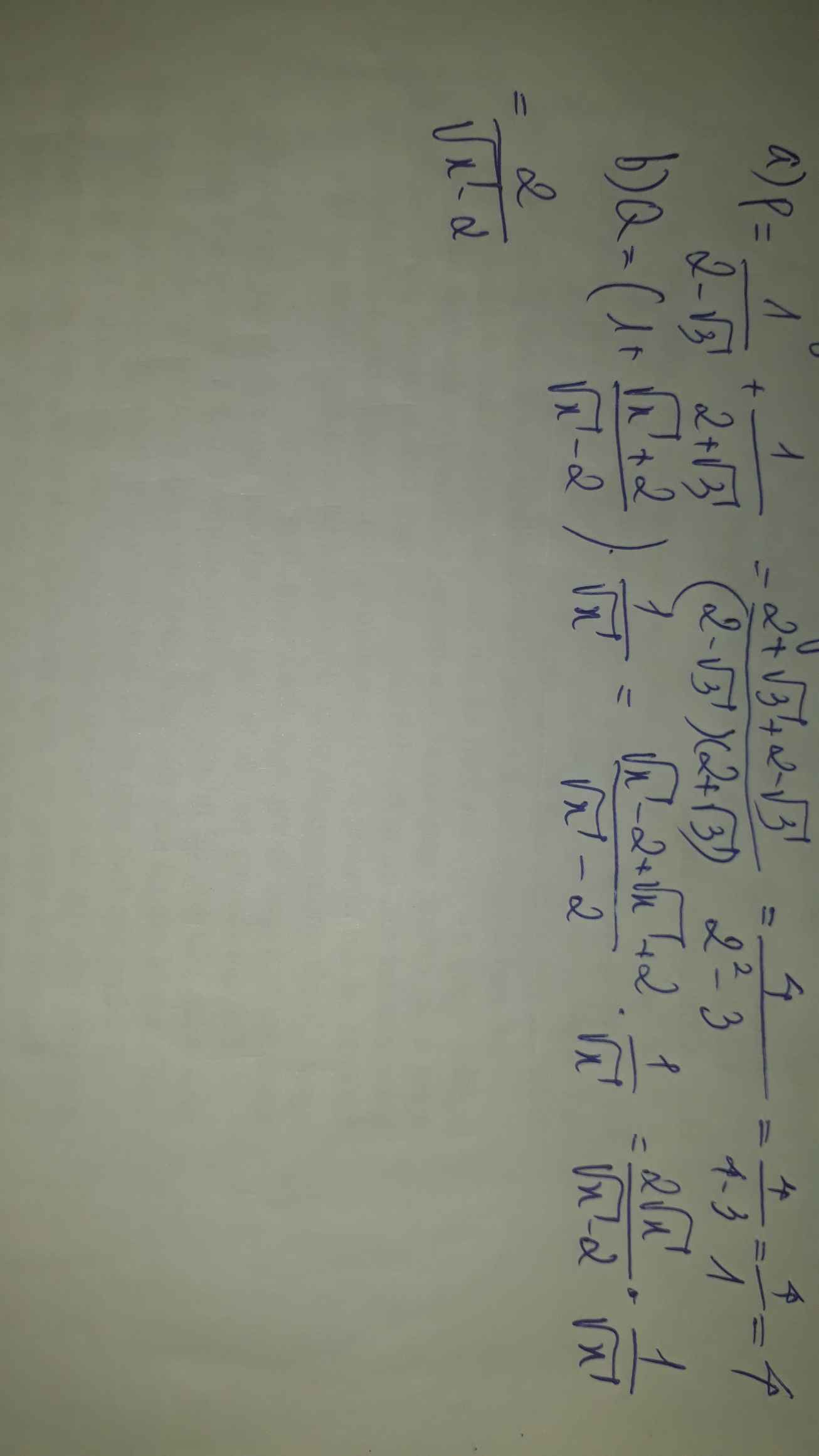
\(P=\left(\dfrac{x-\sqrt{x}+2}{x-\sqrt{x}-2}-\dfrac{x}{x-2\sqrt{x}}\right):\dfrac{1-\sqrt{x}}{2-\sqrt{x}}\)
\(=\left(\dfrac{x-\sqrt{x}+2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}-\dfrac{\sqrt{x}}{\sqrt{x}-2}\right):\dfrac{\sqrt{x}-1}{\sqrt{x}-2}\)
\(=\dfrac{x-\sqrt{x}+2-\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}-2}{\sqrt{x}-1}\)
\(=\dfrac{x-\sqrt{x}+2-x-\sqrt{x}}{\left(\sqrt{x}+1\right)}\cdot\dfrac{1}{\sqrt{x}-1}=\dfrac{-2\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=-\dfrac{2}{\sqrt{x}+1}\)