Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\begin{array}{l}a)\frac{{4{\rm{x}} - 6}}{{5{{\rm{x}}^2} - x}}.\frac{{25{{\rm{x}}^2} - 10{\rm{x}} + 1}}{{27 + 8{{\rm{x}}^3}}}\\ = \frac{{ - 2\left( {3 - 2{\rm{x}}} \right)}}{{x\left( {5{\rm{x}} - 1} \right)}}.\frac{{{{\left( {5{\rm{x}} - 1} \right)}^2}}}{{\left( {3 - 2{\rm{x}}} \right)\left( {9 + 6{\rm{x}} + 4{{\rm{x}}^2}} \right)}}\\ = \frac{{ - 2\left( {5{\rm{x}} - 1} \right)}}{{x\left( {9 + 6{\rm{x}} + 4{{\rm{x}}^2}} \right)}}\\b)\frac{{2{\rm{x}} + 10}}{{{{\left( {x - 3} \right)}^2}}}:\frac{{{{\left( {x + 5} \right)}^3}}}{{{x^2} - 9}}\\ = \frac{{2{\rm{x}} + 10}}{{{{\left( {x - 3} \right)}^2}}}.\frac{{{x^2} - 9}}{{{{\left( {x + 5} \right)}^2}}}\\ = \frac{{2\left( {x + 5} \right)\left( {x - 3} \right)\left( {x + 3} \right)}}{{{{\left( {x - 3} \right)}^2}{{\left( {x + 5} \right)}^3}}}\\ = \frac{{2\left( {x + 3} \right)}}{{\left( {x - 3} \right){{\left( {x + 5} \right)}^2}}}\end{array}\)

a) Tìm thương và dư (nếu có) trong các phép chia \(\left( {3{{\rm{x}}^4}y - 9{{\rm{x}}^3}{y^2} - 21{{\rm{x}}^2}{y^2}} \right):\left( {3{{\rm{x}}^2}y} \right)\)
• Sử dụng lệnh Division(<đa thức bị chia>, <đa thức chia>) để tìm thương và dư của phép chia hai đa thức.
• Nhập biểu thức trên dòng lệnh của cửa sổ CAS sau đó nhấn Enter, kết quả sẽ được hiển thị ngay bên dưới.
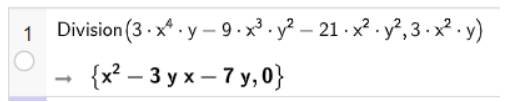
Vậy phép chia hai đa thức \(\left( {3{{\rm{x}}^4}y - 9{{\rm{x}}^3}{y^2} - 21{{\rm{x}}^2}{y^2}} \right)\) cho \(3{{\rm{x}}^2}y\), ta được thương là \({x^2} - 3{\rm{x}}y - 7y\) và dư 0.
b) Tìm thương và dư (nếu có) trong các phép chia (2x3 + 5x2 – 2x + 12) : (2x2 – x + 1).
• Sử dụng lệnh Division(<đa thức bị chia>, <đa thức chia>) để tìm thương và dư của phép chia hai đa thức.
• Nhập biểu thức trên dòng lệnh của cửa sổ CAS sau đó nhấn Enter, kết quả sẽ được hiển thị ngay bên dưới.

Vậy phép chia hai đa thức (2x3 + 5x2 – 2x + 12) cho (2x2 – x + 1), ta được thương là x + 3 và dư 9.

a) \(\left(3x+2\right)^2-\left(3x-2\right)^2=5x+8\)
\(\Rightarrow\left(3x+2+3x-2\right)\left(3x+2-3x+2\right)=5x+8\)
\(\Rightarrow4.6x=5x+8\Rightarrow24x=5x+8\)
\(\Rightarrow19x=8\Rightarrow x=\frac{8}{19}\)
b) \(3\left(x-2\right)^2+9\left(x-1\right)=3\left(x^2+x-3\right)\)
\(\Rightarrow3\left(x^2-4x+4\right)+9x-9=3x^2+3x-9\)
\(\Rightarrow3x^2-12x+12+9x-9=3x^2+3x-9\)
\(\Rightarrow-12x+12+9x-9=3x-9\)
\(\Rightarrow-3x+3=3x-9\)
\(\Rightarrow6x=12\Rightarrow x=2\)

1. (3x - 5)2 - (3x + 1)2 = 8
=> (3x - 5 - 3x - 1)(3x - 5 + 3x + 1) = 8
=> -6(6x - 4) = 8
=> 6x - 4 = \(\dfrac{-4}{3}\)
\(\Rightarrow x=\dfrac{4}{9}\)
2) 2x(8x - 3) - (4x - 3)2 = 27
=> 16x2 - 6x - 16x2 + 24x - 9 = 27
=> 18x - 9 = 27
=> x = 2
3) (2x - 3)2 - (2x + 1)2 = 3
=> (2x - 3 - 2x - 1)(2x - 3 + 2x +1) = 3
=> -4(4x - 2) = 3
=> 4x - 2 = \(\dfrac{-3}{4}\)
\(\Rightarrow x=\dfrac{5}{16}\)
4) (x + 5)2 - x2 = 45
=> (x + 5 - x)(x + 5 + x) = 45
=> 5(2x + 5) = 45
=> 2x + 5 = 9
=> x = 2
5) (x - 3)3 - (x - 3)(x2 + 3x + 9) + 9(x + 1)2 = 18
=> x3 - 9x2 + 27x - 27 - x3 + 27 + 9(x2 + 2x + 1) = 18
=> -9x2 + 27x + 9x2 + 18x + 9 = 18
=> 45x + 9 = 18
=> 45x = 9
=> x = \(\dfrac{1}{5}\)
6) x(x - 4)(x + 4) - (x - 5)(x2 + 5x + 25) = 13
=> x (x2 - 16) - (x3 - 125) = 13
=> x3 - 16x - x3 + 125 = 13
=> -16x = -112
=> x = 7.

Bài 3:
a) \(\left(x-6\right).\left(2x-5\right).\left(3x+9\right)=0\)
\(\Leftrightarrow\left(x-6\right).\left(2x-5\right).3.\left(x+3\right)=0\)
Vì \(3\ne0.\)
\(\Leftrightarrow\left[{}\begin{matrix}x-6=0\\2x-5=0\\x+3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=6\\2x=5\\x=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=6\\x=\frac{5}{2}\\x=-3\end{matrix}\right.\)
Vậy phương trình có tập hợp nghiệm là: \(S=\left\{6;\frac{5}{2};-3\right\}.\)
b) \(2x.\left(x-3\right)+5.\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right).\left(2x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\2x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\2x=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-\frac{5}{2}\end{matrix}\right.\)
Vậy phương trình có tập hợp nghiệm là: \(S=\left\{3;-\frac{5}{2}\right\}.\)
c) \(\left(x^2-4\right)-\left(x-2\right).\left(3-2x\right)=0\)
\(\Leftrightarrow\left(x^2-2^2\right)-\left(x-2\right).\left(3-2x\right)=0\)
\(\Leftrightarrow\left(x-2\right).\left(x+2\right)-\left(x-2\right).\left(3-2x\right)=0\)
\(\Leftrightarrow\left(x-2\right).\left(x+2-3+2x\right)=0\)
\(\Leftrightarrow\left(x-2\right).\left(3x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\3x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\3x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=\frac{1}{3}\end{matrix}\right.\)
Vậy phương trình có tập hợp nghiệm là: \(S=\left\{2;\frac{1}{3}\right\}.\)
Chúc bạn học tốt!

a: =>10x-4=15-9x
=>19x=19
hay x=1
b: \(\Leftrightarrow3\left(10x+3\right)=36+4\left(8x+6\right)\)
=>30x+9=36+32x+24
=>30x-32x=60-9
=>-2x=51
hay x=-51/2
c: \(\Leftrightarrow2x+\dfrac{6}{5}=5-\dfrac{13}{5}-x\)
=>3x=6/5
hay x=2/5
d: \(\Leftrightarrow\dfrac{7x}{8}-\dfrac{5\left(x-9\right)}{1}=\dfrac{20x+1.5}{6}\)
\(\Leftrightarrow21x-120\left(x-9\right)=4\left(20x+1.5\right)\)
=>21x-120x+1080=80x+60
=>-179x=-1020
hay x=1020/179
e: \(\Leftrightarrow5\left(7x-1\right)+60x=6\left(16-x\right)\)
=>35x-5+60x=96-6x
=>95x+6x=96+5
=>x=1
f: \(\Leftrightarrow6\left(x+4\right)+30\left(-x+4\right)=10x-15\left(x-2\right)\)
=>6x+24-30x+120=10x-15x+30
=>-24x+96=-5x+30
=>-19x=-66
hay x=66/19

1) điều kiện xác định : \(x\notin\left\{-1;-2;-3;-4\right\}\)
ta có : \(\dfrac{1}{x^2+3x+2}+\dfrac{1}{x^2+5x+6}+\dfrac{1}{x^2+7x+12}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{1}{\left(x+1\right)\left(x+2\right)}+\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\) \(\Leftrightarrow\dfrac{\left(x+3\right)\left(x+4\right)+\left(x+1\right)\left(x+4\right)+\left(x+1\right)\left(x+2\right)}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)\(\Leftrightarrow\dfrac{x^2+7x+12+x^2+5x+4+x^2+3x+2}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{3x^2+15x+18}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow6\left(3x^2+15x+18\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x^2+5x+6\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x+2\right)\left(x+3\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18=\left(x+1\right)\left(x+4\right)\) ( vì điều kiện xác định )
\(\Leftrightarrow18=x^2+5x+4\Leftrightarrow x^2+5x-14=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+7\right)=0\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x+7=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-7\end{matrix}\right.\left(tmđk\right)\)
vậy \(x=2\) hoặc \(x=-7\) mấy câu kia lm tương tự nha bn

\(\frac{25x-655}{95}-\frac{5\left(x-12\right)}{209}=\frac{89-3x-\frac{2\left(x-18\right)}{5}}{11}\)
\(< =>\frac{5x-131}{19}=\frac{1631-52x-\frac{38x-684}{5}}{209}\)
\(< =>\left(5x-131\right)209=\left(1631-52x-\frac{38x-684}{5}\right)19\)
\(< =>55x-1441=1631-52x-\frac{38x-684}{5}\)
\(< =>3072-107x=\frac{38x-684}{5}\)
\(< =>\left(3072-107x\right)5=38x-684\)
\(< =>15360-535x-38x-684=0\)
\(< =>14676=573x< =>x=\frac{14676}{573}=\frac{4892}{191}\)
nghệm xấu thế
\(\frac{8\left(x+22\right)}{45}-\frac{7x+149+\frac{6\left(x+12\right)}{5}}{9}=\frac{x+35+\frac{2\left(x+50\right)}{9}}{5}\)
\(< =>\frac{8x+176}{45}-\frac{41x+817}{45}=\frac{11x+415}{45}\)
\(< =>993-33x-11x-415=0\)
\(< =>578=44x< =>x=\frac{289}{22}\)
a) Chỗ sai trong phương trình là: \(5 - x + 8 = 3x + 3x - 27\) (dòng thứ 2) vì khi phá ngoặc đã không đổi dấu của số 8.
Sửa lại:
\(\begin{array}{l}5 - \left( {x + 8} \right) = 3x + 3\left( {x - 9} \right)\\\,\,\,\,5 - x - 8 = 3x + 3x - 27\\\,\,\,\,\,\,\, - 3 - x = 6x - 27\\\,\,\,\, - x - 6x = - 27 + 3\\\,\,\,\,\,\,\,\,\,\,\,\, - 7x = - 24\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = \left( { - 24} \right):\left( { - 7} \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = \frac{{24}}{7}\end{array}\)
Vậy phương trình có nghiệm \(x = \frac{{24}}{7}.\)
b) Chỗ sai trong phương trình là: \(4x + 5x = 9 - 18\) (dòng thứ 3) vì khi chuyển \( - 18\) từ vế trái sang vế phải đã không đổi dấu thành \( + 18\).
Sửa lại:
\(\begin{array}{l}3x - 18 + x = 12 - \left( {5x + 3} \right)\\\,\,\,\,\,\,\,4x - 18 = 12 - 5x - 3\\\,\,\,\,\,\,\,4x + 5x = 9 + 18\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,9x = 27\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = 27:9\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x = 3.\end{array}\)
Vậy phương trình có nghiệm \(x = 3.\)