Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\left\{{}\begin{matrix}3\left(x-6\right)< -3\\\frac{5x+m}{2}>7\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x-6< -1\\5x+m>14\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x< 5\\x>\frac{14-m}{5}\end{matrix}\right.\Leftrightarrow\frac{14-m}{5}< x< 5\)
Để hệ có nghiệm thì: \(\frac{14-m}{5}< 5\Leftrightarrow14-m< 25\Leftrightarrow m>-11\)
Chúc bạn học tốt nha![]()

+ ĐKXĐ : \(\left\{{}\begin{matrix}x\ge-3\\y\ge-4\end{matrix}\right.\)
\(gt\Rightarrow x+y=6\left(\sqrt{x+3}+\sqrt{4+y}\right)\le6\sqrt{2\left(x+y+7\right)}\)
\(\Rightarrow\left(x+y\right)^2\le72\left(x+y+7\right)\)
\(\Rightarrow\left(x+y\right)^2-72\left(x+y\right)-504\le0\)
\(\Rightarrow\left(x+y-36\right)^2\le1800\Rightarrow P\le36+30\sqrt{2}\)
max \(P=36+30\sqrt{2}\Leftrightarrow\left\{{}\begin{matrix}x+3=y+4\\x+y=36+30\sqrt{2}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\frac{37}{2}+15\sqrt{2}\\y=\frac{35}{2}+15\sqrt{2}\end{matrix}\right.\)
+ \(x+y=6\left(\sqrt{x+3}+\sqrt{y+4}\right)\)
\(\Rightarrow\left(x+y\right)^2=36\left(x+y+7+2\sqrt{\left(x+3\right)\left(y+4\right)}\right)\)
\(\Rightarrow\left(x+y\right)^2-36\left(x+y\right)-252=72\sqrt{\left(x+3\right)\left(y+4\right)}\ge0\)
\(\Rightarrow\left(x+y-42\right)\left(x+y+6\right)\ge0\Rightarrow x+y\ge42\)
Min \(P=42\Leftrightarrow\left\{{}\begin{matrix}\sqrt{\left(x+3\right)\left(y+4\right)}=0\\x+y=42\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x=-3\\y=45\end{matrix}\right.\\\left\{{}\begin{matrix}x=46\\y=-4\end{matrix}\right.\end{matrix}\right.\)

câu 4 \(\sqrt{x^2-2x}=\sqrt{2x-x^2}\Leftrightarrow x^2-2x=2x-x^2\)
\(\Leftrightarrow2\left(x^2-2x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
câu C
Câu 5 \(x\left(x^2-1\right)\sqrt{x-1}=0\)
ĐK \(x\ge1\)
\(\Leftrightarrow\left(x-1\right)\left(x+1\right)\sqrt{x-1}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+1=0\\\sqrt{x-1}=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\left(nh\right)\\x=-1\left(l\right)\end{matrix}\right.\)
vậy pt có 1 nghiệm
câu B

Lời giải:
Đặt $\sqrt{x+2}=t(t\geq 0)$ thì pt trở thành:
$t^2-2-2t-m-3=0$
$\Leftrightarrow t^2-2t-(m+5)=0(*)$
Để PT ban đầu có 2 nghiệm pb thì PT $(*)$ có 2 nghiệm không âm phân biệt.
Điều này xảy ra khi \(\left\{\begin{matrix} \Delta'=1+m+5>0\\ S=2>0\\ P=-(m+5)\geq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} m>-6\\ m\leq -5\end{matrix}\right.\)
Đáp án B.
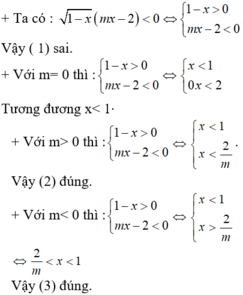
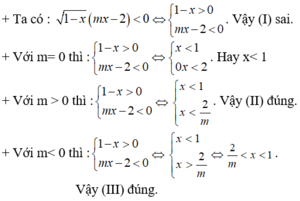



2 x + 1 = x - 1 ⇔ 2 x + 1 ≥ 0 x - 1 ≥ 0 2 x + 1 = x 2 - 2 x + 1 ⇔ x ≥ - 1 2 x ≥ 1 - x 2 + 4 x = 0 ⇔ x ≥ 1 [ x = 0 ⇔ x = 4 x = 4
Chọn A.