Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(f\left(x\right)=e^{sinx}-sinx-1\)
\(\Rightarrow f'\left(x\right)=cosx.e^{sinx}-cosx=cosx\left(e^{sinx}-1\right)\)
\(f'\left(x\right)=0\Leftrightarrow\left[{}\begin{matrix}cosx=0\\sinx=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{\pi}{2}\\x=\pi\end{matrix}\right.\)
\(f\left(0\right)=0\) ; \(f\left(\dfrac{\pi}{2}\right)=e-2\) ; \(f\left(\pi\right)=0\)
\(\Rightarrow f\left(x\right)_{min}=0\) ; \(f\left(x\right)_{max}=e-2\)

a) 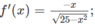
f′(x) > 0 trên khoảng (-4; 0) và f’(x) < 0 trên khoảng (0; 4).
Hàm số đạt cực đại tại x = 0 và f C Đ = 5
Mặt khác, ta có f(-4) = f(4) = 3
Vậy 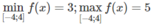
d) f(x) = | x 2 − 3x + 2| trên đoạn [-10; 10]
Khảo sát sự biến thiên và vẽ đồ thị của hàm số g(x) = x 2 – 3x + 2.
Ta có:
g′(x) = 2x − 3; g′(x) = 0 ⇔ x = 3/2
Bảng biến thiên:
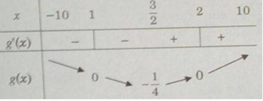
Vì
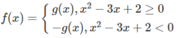
nên ta có đồ thị f(x) như sau:
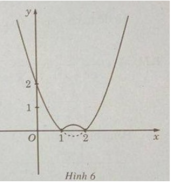
Từ đồ thị suy ra: min f(x) = f(1) = f(2) = 0; max = f(x) = f(−10) = 132
e) 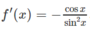
f′(x) < 0 nên và f’(x) > 0 trên (π/2; 5π/6] nên hàm số đạt cực tiểu tại x = π/2 và f C T = f(π/2) = 1
Mặt khác, f(π/3) = 2√3, f(5π/6) = 2
Vậy min f(x) = 1; max f(x) = 2
g) f(x) = 2sinx + sin2x trên đoạn [0; 3π/2]
f′(x) = 2cosx + 2cos2x = 4cos(x/2).cos3(x/2)
f′(x) = 0
⇔ 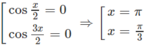
Ta có: f(0) = 0,
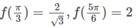
Từ đó ta có: min f(x) = −2 ; max f(x) = 3√3/2

f(x) = 2sinx + sin2x trên đoạn [0; 3 π /2]
f′(x) = 2cosx + 2cos2x = 4cos(x/2).cos3(x/2)
f′(x) = 0
⇔ 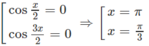
Ta có: f(0) = 0,
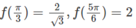
Từ đó ta có: min f(x) = −2 ; max f(x) = 3 3 /2

\(f\left(x\right)=\dfrac{2x-1}{x-3}=\dfrac{2\left(x-3\right)+5}{x-3}=1+\dfrac{5}{\left(x-3\right)}\)
f(x) có dạng \(y=\dfrac{5}{x}\Rightarrow\) f(x) luôn nghịch biến
Tất nhiên bạn có thể tính đạo hàm --> f(x) <0 mọi x khác -3
f(x) luôn nghich biến [0;2] < -3 thuộc nhánh Bên Phải tiệm cận đứng
\(\Rightarrow\left\{{}\begin{matrix}Max=f\left(0\right)=\dfrac{1}{3}\\Min=f\left(2\right)=-3\end{matrix}\right.\)

\(f'\left(x\right)=1-\dfrac{9}{x^2}\)
\(f'\left(x\right)=0\Rightarrow x=\pm3\)
\(f''\left(x\right)=\dfrac{18}{x^3}\) \(\left\{{}\begin{matrix}f''\left(3\right)>0\\f''\left(-3\right)< 0\end{matrix}\right.\) vậy f(x) đạt cực tiểu tại x=3 trong khoảng đang xét hàm liên tục [2,4]
\(f\left(3\right)=3+\dfrac{9}{3}=6\)
\(\left\{{}\begin{matrix}f\left(2\right)=2+\dfrac{9}{2}=\dfrac{13}{2}\\f\left(4\right)=4+\dfrac{9}{4}=\dfrac{25}{4}< \dfrac{13}{2}\end{matrix}\right.\)
kết luận
GTLN f(x) trên đoạn [2,4] =\(\dfrac{13}{2}\)
GTNN f(x) trên đoạn [2,4] = \(6\)
\(f'\left(x\right)=1-\dfrac{9}{x^2}=\dfrac{x^2-9}{x^2}\)
\(f'\left(x\right)=0\Leftrightarrow x=\pm3\)
Hàm số nghịch biến trong các khoảng (-3; 0), (0; 3) và đồng biến trong các khoảng \(\left(-\infty;3\right)\left(3;+\infty\right)\)
Ta có bảng biến thiên:| x | \(-\infty;-3;0\) | \(2;3;4;+\infty\) |
| f'(x) | + 0 - | - - 0 + + |
| f(x) | yCĐ | yCT +∞ |
Ta có: \(\left[2;4\right]\subset\left(0;+\infty\right);\left[{}\begin{matrix}f\left(2\right)=6,5\\f\left(3\right)=6\\f\left(4\right)=6,25\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\min\limits_{\left[2;4\right]}f\left(x\right)=f\left(3\right)=6\\\max\limits_{\left[2;4\right]}f\left(x\right)=f\left(2\right)=6,5\end{matrix}\right.\)


Ta có : \(f'\left(x\right)=2x+\frac{2}{1-2x}=\frac{-4x^2+2x+2}{1-2x}=0\Leftrightarrow-4x^2+2x+2=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=-\frac{1}{2}\in\left[-2;0\right]\\x=1\notin\left[-2;0\right]\end{array}\right.\)
Mà :
\(\begin{cases}f\left(-2\right)=4-\ln5;x=-2\\f\left(-\frac{1}{2}\right)=\frac{1}{4}-\ln2=\frac{1-4\ln2}{4};x=-\frac{1}{2}\\\end{cases}\)

\(f\left(x\right)=\frac{x^2}{2}-4\ln\left(3-x\right)\) trên đoạn \(\left[-2;1\right]\)
Ta có :
\(f'\left(x\right)=x+\frac{4}{3-x}=\frac{-x^2+3x+4}{3-x}=0\Leftrightarrow-x^2+3x+4=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=-1\in\left[-2;1\right]\\x=4\notin\left[-2;1\right]\end{array}\right.\)
Mà :
\(\begin{cases}f\left(-2\right)=2-4\ln5\\f\left(-1\right)=\frac{1}{2}-8\ln2=\frac{1-16\ln2}{2}\\f\left(1\right)=\frac{1}{2}-4\ln2=\frac{1-8\ln2}{2}\end{cases}\) \(\Rightarrow\begin{cases}Max_{x\in\left[-2;1\right]}f\left(x\right)=\frac{1-8\ln2}{2};x=1\\Min_{x\in\left[-2;1\right]}f\left(x\right)=\frac{1-16\ln2}{2};x=-1\end{cases}\)
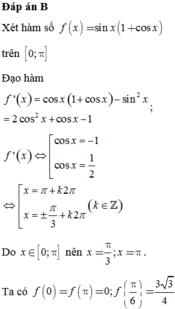
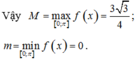
f′(x) < 0 nên và f’(x) > 0 trên ( π /2; 5 π /6] nên hàm số đạt cực tiểu tại x = π /2 và f CT = f( π /2) = 1
Mặt khác, f( π /3) = 2 3 , f(5 π /6) = 2
Vậy min f(x) = 1; max f(x) = 2