Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\Delta'=b'^2-ac=-6m+7=>\)\(m\ge\frac{7}{6}\)
Theo Vi-ét : \(\hept{\begin{cases}x_1+x_2=2\left(m-2\right)\\x_1.x_2=m^2+2m-3\end{cases}}\)Mà \(\frac{1}{x_1}+\frac{1}{x_2}=\frac{x_1+x_2}{5}=>\)\(\frac{x_1+x_2}{x_1.x_2}=\frac{x_1+x_2}{5}\)
=> \(x_1.x_2=5\)<=> \(m^2+2m-3=5\)<=> \(m^2+2m-8=0\)
Giải pt trên ta đc : \(\orbr{\begin{cases}m=2\\m=-4\end{cases}}\)Mà \(m\ge\frac{7}{6}\)=> \(m=2\)

PT ( 1 ) có \(\Delta=[-\left(m-1\right)]^2-4\cdot1\cdot\left(-m\right)\):
\(=m^2-2m+1+4m\)
\(=m^2+2m+1=\left(m+1\right)^2\)
Để PT ( 1 ) có 2 nghiệm pb \(x_1,x_2\)
\(\Leftrightarrow\Delta>0\)
\(\Leftrightarrow\left(m+1\right)^2>0\)
\(\Leftrightarrow m+1\ne0\)
\(\Leftrightarrow m\ne-1\)
Với \(m\ne-1\), áp dụng hệ thức Vi-ét cho PT ( 1 ) ta đc :
\(x_1+x_2=m-1\)
\(x_1\cdot x_2=-m\)
Theo đề bài :
\(x_1\cdot\left(3+x_1\right)+x_2\cdot\left(3+x_2\right)=-4\)
\(\Leftrightarrow3x_1+x_1^2+3x_2+x_2^2=-4\)
\(\Leftrightarrow3\cdot\left(x_1+x_2\right)+\left(x_1+x_2\right)^2-2x_1x_2=-4\)
\(\Leftrightarrow3\cdot\left(m-1\right)+\left(m-1\right)^2-2\cdot\left(-m\right)=-4\)
\(\Leftrightarrow3m-3+m^2-2m+1+2m+4=0\)
\(\Leftrightarrow m^2+3m+2=0\)\(\Leftrightarrow\left(m+1\right)\cdot\left(m+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}m+1=0\\m+2=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}m=-1\left(ktm\right)\\m=-2\left(tm\right)\end{cases}}\)
Vậy m = -2 là giá trị cần tìm
M làm hết r đấy nhưng chắc là ko tải hết lên đc
Giải đến đấy rùi thì chắc bạn tự giải tiếp đc đúng hông???

Câu a )
\(2x^4+3x^2-2=0\left(1\right)\)
Đặt \(t=x^2\left(t\ge0\right)\) phương trình (1) trở thành:
\(2t^2+3t-2=0\)
\(\Leftrightarrow t\left(2t-1\right)+4t-2=0\)
\(\Leftrightarrow t\left(2t-1\right)+2\left(2t-1\right)=0\)
\(\Leftrightarrow\left(2t-1\right)\left(t+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}2t-1=0\\t+2=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}t=\frac{1}{2}\\1=-2\left(loại\right)\end{cases}}\)
Với \(t=\frac{1}{2}\Leftrightarrow x^2=\frac{1}{2}\Rightarrow x=\pm\frac{\sqrt{2}}{2}\)
Vậy tập nghiệm của phương trình là \(S=\left\{\pm\frac{\sqrt{2}}{2}\right\}\)
Câu b )
\(\Delta=\left(m+1\right)^2-4m=m^2-2m+1=\left(m-1\right)^2\)
\(\Delta>0\Leftrightarrow\left(m-1\right)^2>0\Leftrightarrow m\ne1\)
\(\hept{\begin{cases}x_1+x_2=m+1\\x_1x_2=m\end{cases}}\)
\(x_1=3x_2\Rightarrow3x_2+x_2=m+1\Leftrightarrow4x_2=m+1\)
\(\Leftrightarrow x_2=\frac{m+1}{4}\Rightarrow x_1=\frac{3\left(m+1\right)}{4}\)
\(x_1x_2=m\Leftrightarrow\frac{3\left(m+1\right)^2}{16}=m\)
\(\Leftrightarrow3m^2+6m+3=16m\)
\(\Leftrightarrow3m^2-10m+3=0\)
\(\Leftrightarrow\left(3m-1\right)\left(m-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}m=\frac{1}{3}\\m=3\end{cases}\left(tm\right)}\)
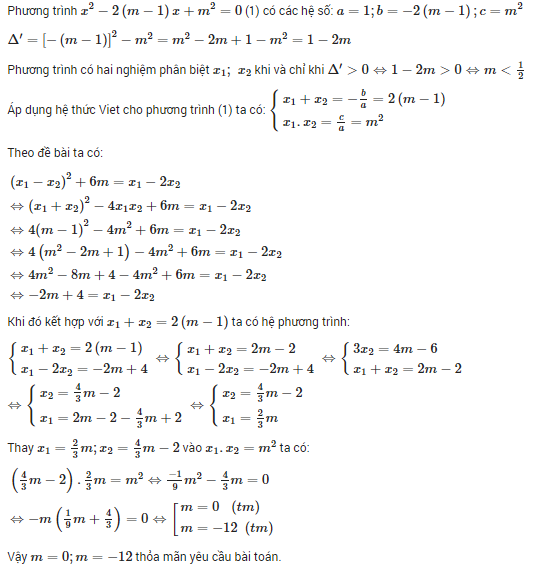
\(x^2-2\left(m-1\right)x+m^2-4=0\)
\(\Delta=b^2-4ac=\left[-2\left(m-1\right)\right]^2-4\left(m^2-4\right)\)
\(=4\left(m^2-2m+1\right)-4\left(m^2-4\right)\)
\(=4m^2-8m+4-4m^2+16\)
\(=-8m+20\)
Để pt đã cho có 2 nghiệm pb \(x_1,x_2\) thì \(\Delta>0\Leftrightarrow-8m+20>0\Leftrightarrow-8m>-20\Leftrightarrow m< \dfrac{5}{2}\)
Theo Vi-ét, ta có :
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m^2-4\end{matrix}\right.\)
Ta có : \(x_1\left(x_1-3\right)+x_2\left(x_2-3\right)=6\)
\(\Leftrightarrow x_1^2-3x_1+x^2_2-3x_2=6\)
\(\Leftrightarrow\left(x_1^2+x_2^2\right)-3\left(x_1+x_1\right)-6=0\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2-3\left(x_1+x_2\right)-6=0\)
\(\Leftrightarrow\left(2m-2\right)^2-2\left(m^2-4\right)-3\left(2m-2\right)-6=0\)
\(\Leftrightarrow4m^2-8m+4-2m^2+8-6m+6-6=0\)
\(\Leftrightarrow2m^2-14m+12=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=6\left(ktm\right)\\m=1\left(tm\right)\end{matrix}\right.\)
Vậy m = 1 thì thỏa mãn đề bài.