DT
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AH
Akai Haruma
Giáo viên
26 tháng 10 2023
Lời giải:
1.
$x^3+3x^2-16x-48=(x^3+3x^2)-(16x+48)=x^2(x+3)-16(x+3)$
$=(x+3)(x^2-16)=(x+3)(x-4)(x+4)$
2.
$4x(x-3y)+12y(3y-x)=4x(x-3y)-12y(x-3y)=(x-3y)(4x-12y)=4(x-3y)(x-3y)=4(x-3y)^2$
3.
$x^3+2x^2-2x-1=(x^3-x^2)+(3x^2-3x)+(x-1)=x^2(x-1)+3x(x-1)+(x-1)$
$=(x-1)(x^2+3x+1)$
T
26 tháng 10 2023
1)
\((x+2)(x+3)(x+4)(x+5)-24\\=[(x+2)(x+5)]\cdot[(x+3)(x+4)]-24\\=(x^2+7x+10)(x^2+7x+12)-24\)
Đặt \(x^2+7x+10=y\), khi đó biểu thức trở thành:
\(y(y+2)-24\\=y^2+2y-24\\=y^2+2y+1-25\\=(y+1)^2-5^2\\=(y+1-5)(y+1+5)\\=(y-4)(y+6)\\=(x^2+7x+10-4)(x^2+7x+10+6)\\=(x^2+7x+6)(x^2+7x+16)\)
2) Bạn xem lại đề!
TN
14 tháng 7 2016
a)xm+4+xm+3-x-1
=(xm+4-x)+(xm+3-1)
=x(xm+3-1)+(xm+3-1)
=(x+1)(xm+3-1)
Với x=-2 ta có:... bn tự thay
b)x6-x4+2x3+2x2=x6-2x5+2x4+2x5-4x4+4x3+x4-2x3+2x2
=x4(x2-2x+2)+2x3(x2-2x+2)+x2(x2-2x+2)
=(x4+2x3+x2)(x2-2x+2)
=[x2(x2+2x+1)](x2-2x+2)
=x2(x+1)2(x2-2x+2)
Với x=-2 bn tự thay nhé h mk bận
1 tháng 8 2023
Ta có :
\(AH^2=AB^2+BH^2\left(1\right)\) (Δ ABH vuông tại H)
\(AH^2=AC^2+CH^2\left(2\right)\) (Δ ACH vuông tại H)
\(\left(1\right),\left(2\right)\Rightarrow AB^2+BH^2=AC^2+CH^2\)
\(\Rightarrow CH^2=AB^2+BH^2-AC^2\)
\(\Rightarrow CH^2=81+676-121=636\)
\(\Rightarrow CH=\sqrt[]{636}=\sqrt[]{4.159}=2\sqrt[]{159}\left(cm\right)\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
31 tháng 7 2023
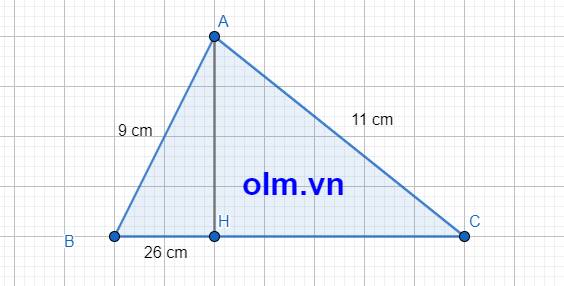
Vì AH là đường cao của tam giác ABC nên AH \(\perp\) BC \(\equiv\) H
⇒ \(\Delta\) AHB \(\perp\) \(\equiv\) H \(\Rightarrow\) AB > BH ⇒ 9 cm > 26 cm vô lý
Em có hai sựa lựa chọn: 1 là em chỉ ra cái sai của cô
2 là em xem lại đề bài của em
T
17 tháng 11 2023
\(\dfrac{4x+2}{4x-2}+\dfrac{3-6x}{6x-6}\left(dkxd:x\ne\dfrac{1}{2};x\ne1\right)\)
\(=\dfrac{2\left(2x+1\right)}{2\left(2x-1\right)}+\dfrac{3\left(1-2x\right)}{6\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2x-2}\)
\(=\dfrac{\left(2x+1\right)\left(2x-2\right)}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{\left(1-2x\right)\left(2x-1\right)}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{4x^2-6x+2}\)
NV
14 tháng 7 2016
5x2 - 4(x2 - 2x + 1) - 5 = 0
=> 5x2 - 4x2 + 8x - 4 - 5 = 0
=> x2 + 8x - 9 = 0
=> x2 + 9x - x - 9 = 0
=> x(x + 9) - (x + 9) = 0
=> (x + 9)(x - 1) = 0
=> x + 9 = 0 => x = -9
hoặc x - 1 = 0 = > x = 1
Vậy x = -9, x = 1
14 tháng 7 2016
\(5x^2-4\left(x^2-2x+1\right)-5=0\)
\(\left(5x^2-5\right)-4\left(x^2-2.1.x+1^2\right)=0\)
\(5\left(x^2-1\right)-4\left(x-1\right)^2=0\)
\(5\left(x-1\right)\left(x+1\right)-4\left(x-1\right)\left(x-1\right)=0\)
\(\left[5\left(x+1\right)-4\left(x-1\right)\right]\left(x-1\right)=0\)
\(\left(5x+5-4x+4\right)\left(x-1\right)=0\)
\(\left(x+9\right)\left(x-1\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x+9=0\\x-1=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=-9\\x=1\end{cases}}\)
Vậy \(\orbr{\begin{cases}x=-9\\x=1\end{cases}}.\)
D
28 tháng 7 2023
`x^2+x+1=x^2+x+1/4+3/4=(x+1/2)^2 +3/4`
Vì `(x+1/2)^2 >= 0` với mọi `x`
`=>(x+1/2)^2 +3/4 >= 3/4` với mọi `x`
`=>` Biểu thức Min `=3/4<=>x=-1/2`
_____________
`(x-3)(x+5)+4=x^2+2x-11=x^2+2x+1-12=(x+1)^2-12`
Vì `(x+1)^2 >= 0` với mọi `x`
`=>(x+1)^2-12 >= -12` với mọi `x`
`=>` Biểu thức Min `=-1/2<=>x=-1`
$m - 3 = 2m + 5$
=> $m - 2m = 5 + 3$
=> $-m = 8$
=> $m = -8$