
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




\(\dfrac{1}{n\left(n+1\right)}=\dfrac{1+n-n}{n\left(n+1\right)}=\dfrac{n+1}{n\left(n+1\right)}-\dfrac{n}{n\left(n+1\right)}=\dfrac{1}{n}-\dfrac{1}{n+1}\)

4.
\(\dfrac{x-7}{y-6}=\dfrac{7}{6}\Rightarrow\dfrac{x-7}{7}=\dfrac{y-6}{6}=\dfrac{-y+6}{-6}\)
Áp dụng tính chất dãy tỉ số bằng nhau:
\(\dfrac{x-7}{7}=\dfrac{-y+6}{-6}=\dfrac{x-7-y+6}{7-6}=\dfrac{x-y-1}{1}=-5\)
\(\Rightarrow\left\{{}\begin{matrix}x-7=7.\left(-5\right)=-35\\-y+6=\left(-6\right).\left(-5\right)=30\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=-28\\y=-24\end{matrix}\right.\)
5.
Ta có:
\(A^2=\dfrac{2^2.4^2.6^2...4998^2.5000^2}{3^2.5^2.7^2...4999^2.5001^2}< \dfrac{2^2.4^2.6^2.4998^2.5000^2}{\left(3^2-1\right)\left(5^2-1\right)\left(7^2-1\right)...\left(4999^2-1\right)\left(5001^2-1\right)}\)
\(\Rightarrow A^2< \dfrac{2^2.4^4.6^2...4998^2.5000^2}{2.4.4.6.6.8...4998.5000.5000.5002}=\dfrac{2^2.4^4.6^2...4998^2.5000^2}{2.4^4.6^2...4998^2.5000^2.5002}\)
\(\Rightarrow A^2< \dfrac{2}{5002}=\dfrac{1}{2501}< \dfrac{1}{2500}\)
\(\Rightarrow A< \dfrac{1}{50}\)
\(\Rightarrow A< 0,02\)
Bài 3:
\(A=B\) khi:
\(\dfrac{7}{y-2}=x+1\left(y\ne2\right)\)
\(\Rightarrow\left(x+1\right)\left(y-2\right)=7\)
Mà: x,y nguyên \(\Rightarrow x+1,y-2\inƯ\left(7\right)=\left\{1;-1;7;-7\right\}\)
Ta có bảng sau:
| x + 1 | 1 | -1 | 7 | -7 |
| y - 2 | 7 | -7 | 1 | -1 |
| x | 0 | -2 | 6 | -8 |
| y | 9 | -5 | 3 | 1 |

\(\dfrac{15}{34}+\dfrac{1}{3}+\dfrac{19}{34}-\dfrac{4}{3}+\dfrac{3}{7}=\left(\dfrac{15}{34}+\dfrac{19}{34}\right)+\left(\dfrac{1}{3}-\dfrac{4}{3}\right)+\dfrac{3}{7}=1-1+\dfrac{3}{7}=\dfrac{3}{7}\)

Lời giải:
a.
$=\frac{3}{5}-\frac{7}{4}=\frac{12-35}{20}=\frac{-23}{20}$
b.
$=-(2+\frac{5}{8})=-\frac{21}{8}$
c.
$=-(\frac{1}{8}+\frac{5}{9})=-\frac{9+8.5}{8.9}=\frac{-49}{72}$
d.
$=\frac{6}{13}-\frac{14}{39}=\frac{18}{39}-\frac{14}{39}=\frac{4}{39}$
e.
$=\frac{-3}{4}+\frac{5}{7}=\frac{5}{7}-\frac{3}{4}$
$=\frac{20-21}{7.4}=\frac{-1}{28}$

Bài 5
1) x ∈ Ư(18) = {1; 2; 3; 6; 9; 18}
x ∈ B(4) = {0; 4; 8; 12; 16; 20; ...}
Vậy không tìm được x thỏa mãn đề bài
2) x ∈ Ư(20) = {1; 2; 4; 5; 10; 20}
x ∈ B(2) = {0; 2; 4; 6; 8; 10; 12; 14; 16; 18; 20; ...}
⇒ x ∈ {2; 4; 10; 20}
3) x ∈ B(12) = {0; 12; 24; 36; 48; ...; 96; 108; ...}
Mà 30 ≤ x ≤ 100
⇒ x ∈ {36; 48; ...; 96}
4) x ∈ Ư(150) = {1; 2; 3; 5; 6; 10; 15; 25; 30; 50; 75; 150}
Mà x ≤ 50
⇒ x ∈ {1; 2; 3; 5; 6; 10; 15; 25; 30; 50}
5) 70 ⋮ x và 168 ⋮ x
⇒ x ∈ ƯC(70; 168)
Ta có:
70 = 2.5.7
168 = 2³.3.7
⇒ ƯCLN(70; 168) = 2.7 = 14
⇒ x ∈ ƯC(70; 168) = Ư(14) = {1; 2; 7; 14}
Mà x > 10
⇒ x = 14
6) Ta có:
(1995 + 2005 + x) ⋮ 5
1995 ⋮ 5
2005 ⋮ 5
⇒ x ⋮ 5
⇒ x ∈ B(5) = {0; 5; 10; 15; 20; 25; 30; 35; 40; ...}
Mà 23 < x ≤ 35
⇒ x ∈ {25; 30; 35}
Bài 6
1) Do 17x2y chia hết cho 2 và 5 nên y = 0
⇒ Số đã cho có dạng: 17x20
Để 17x20 chia hết cho 3 thì (1 + 7 + x + 2 + 0) ⋮ 3
⇒ (10 + x) ⋮ 3
⇒ x ∈ {2; 5; 8}
Vậy x ∈ {2; 5; 8}; y = 0
2) Do 234xy chia hết cho 2 và 5 nên y = 0
⇒ Số đã cho có dạng: 234x0
Để 234x0 chia hết cho 9 thì (2 + 3 + 4 + x + 0) ⋮ 9
⇒ (9 + x) ⋮ 9
⇒ x ∈ {0; 9}
Vậy x ∈ {0; 9}; y = 0
3) Do 4x6y chia hết cho 2 và 5 nên y = 0
Mà x - y = 4
⇒ x = 4 + y
⇒ x = 4
Vậy x = 4; y = 0
4) Do 57x2y chia hết cho 5 nhưng không chia hết cho 2 nên y = 5
⇒ Số đã cho có dạng 57x25
Để 57x25 chia hết cho 9 thì (5 + 7 + x + 2 + 5) ⋮ 9
⇒ (19 + x) ⋮ 9
⇒ x = 8
Vậy x = 8; y = 5


\(a,-\dfrac{5}{7}+1+\dfrac{30}{-7}\le x\le-\dfrac{1}{6}+\dfrac{1}{3}+\dfrac{5}{6}\\ \dfrac{-5+1.7-30}{7}\le x\le\dfrac{-1+1.2+5}{6}\\ -\dfrac{28}{7}\le x\le\dfrac{6}{6}\\ -4\le x\le1\\ Vậy:x\in\left\{-4;-3;-2;-1;0;1\right\}\)
\(b,\dfrac{-8}{13}+\dfrac{7}{17}+\dfrac{21}{13}\le x\le-\dfrac{9}{14}+3+\dfrac{5}{-14}\\ \left(\dfrac{21}{13}-\dfrac{8}{13}\right)+\dfrac{7}{17}\le x\le\left(-\dfrac{9}{14}-\dfrac{5}{14}\right)+3\\ 1+\dfrac{7}{17}\le x\le-1+3\\ 1\dfrac{7}{17}\le x\le2\\ Vậy:x=2\)


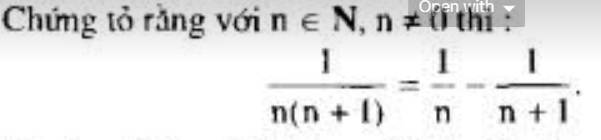

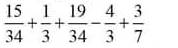
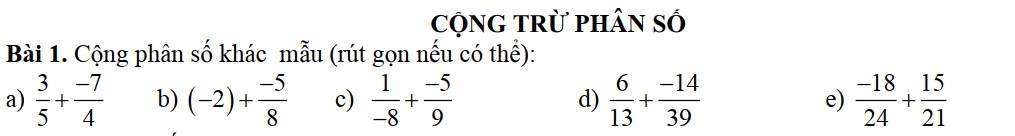

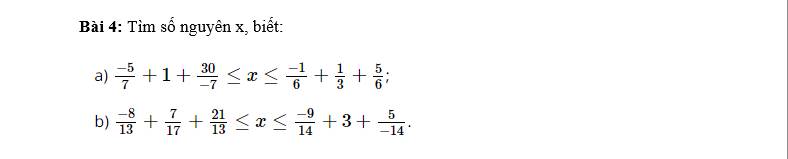
o: \(\dfrac{-35}{120}=\dfrac{-35\cdot3}{120\cdot3}=\dfrac{-105}{360}\)
\(\dfrac{19}{-45}=\dfrac{-19}{45}=\dfrac{-19\cdot8}{45\cdot8}=\dfrac{-152}{360}\)
mà -105>-152
nên \(-\dfrac{35}{120}>-\dfrac{19}{45}\)
p: \(\dfrac{-12}{48}=\dfrac{-1}{4}=\dfrac{-13}{52}\)
\(\dfrac{-7}{26}=\dfrac{-7\cdot2}{26\cdot2}=\dfrac{-14}{52}\)
mà -13>-14
nên \(-\dfrac{12}{48}>-\dfrac{7}{26}\)
q: \(\dfrac{-8}{-90}=\dfrac{8}{90}\)
\(\dfrac{14}{42}=\dfrac{1}{3}=\dfrac{30}{90}\)
mà 8<30
nên \(\dfrac{-8}{-90}< \dfrac{14}{42}\)
r: \(\dfrac{-34}{68}=\dfrac{-1}{2}=\dfrac{-28}{56}\)
\(\dfrac{28}{-51}=\dfrac{-28}{51}\)
56>51
=>\(\dfrac{28}{56}< \dfrac{28}{51}\)
=>\(-\dfrac{28}{56}>-\dfrac{28}{51}\)
=>\(\dfrac{-34}{68}>\dfrac{28}{-51}\)
t: \(\dfrac{-19}{60}< 0\)
\(0< \dfrac{42}{45}=\dfrac{-42}{-45}\)
Do đó: \(\dfrac{-19}{60}< \dfrac{-42}{-45}\)
v: \(\dfrac{1}{-2024}< 0\)
\(0< \dfrac{-2023}{-45}=\dfrac{2023}{45}\)
Do đó: \(\dfrac{1}{-2024}< \dfrac{-2023}{-45}\)
x: Đặt \(A=\dfrac{2024^{2025}+1}{2024^{2026}+1};B=\dfrac{2024^{2000}+1}{2024^{2001}+1}\)
\(2024A=\dfrac{2024^{2026}+2024}{2024^{2026}+1}=1+\dfrac{2023}{2024^{2026}+1}\)
\(2024B=\dfrac{2024^{2001}+2024}{2024^{2001}+1}=1+\dfrac{2023}{2024^{2001}+1}\)
\(2024^{2026}+1>2024^{2001}+1\)
=>\(\dfrac{2023}{2024^{2026}+1}< \dfrac{2023}{2024^{2001}+1}\)
=>2024A<2024B
=>A<B