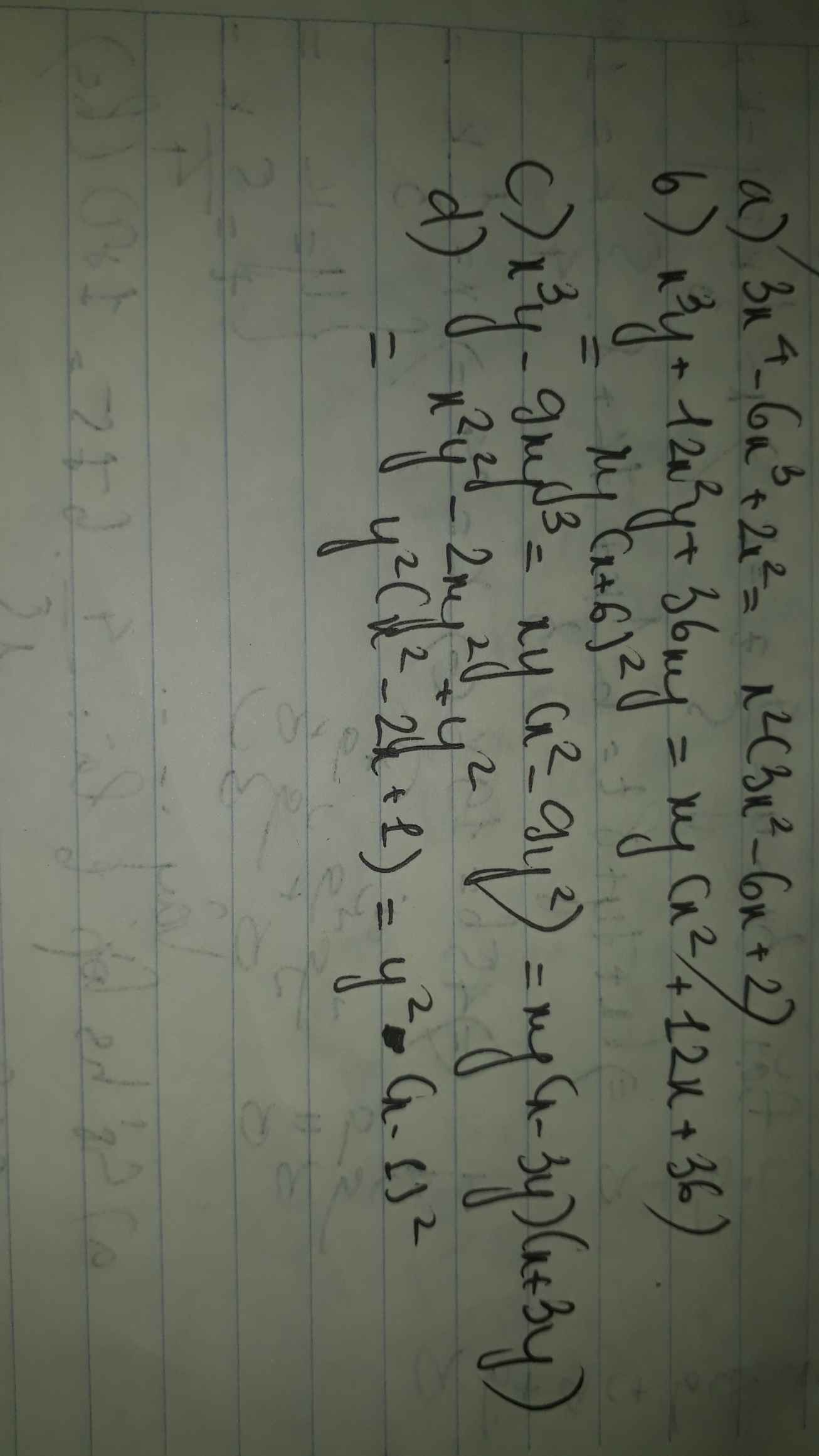LC
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
30 tháng 10 2021
\(=x\left(x^4-x^2-2x-1\right)\)
\(=x\left(x^4-x^3-x^2+x^3-x^2-x+x^2-x-1\right)\)
\(=x\left(x^2-x-1\right)\left(x^2+x+1\right)\)
26 tháng 10 2021
a. \(2x^{^2}\left(2x^{^3}-3x+1\right)\)
b. \(\left(4m-25n\right)\left(4m+25n\right)\)
26 tháng 10 2021
b: \(4m^2-25n^2=\left(2m-5n\right)\left(2m+5n\right)\)
AH
Akai Haruma
Giáo viên
7 tháng 9 2021
Lời giải:
a.
$3x^2+xy-4y^2=(3x^2-3xy)+(4xy-4y^2)=3x(x-y)+4y(x-y)=(x-y)(3x+4y)$
b.
$x^8-5x^4+4=(x^8-x^4)-(4x^4-4)$
$=x^4(x^4-1)-4(x^4-1)=(x^4-1)(x^4-4)$
$=(x^2-1)(x^2+1)(x^2-2)(x^2+2)$
$=(x-1)(x+1)(x^2+1)(x-\sqrt{2})(x+\sqrt{2})(x^2+2)$
c.
$x^3+3x^2+3x-7=(x^3+3x^2+3x+1)-8$
$=(x+1)^3-2^3=(x+1-2)[(x+1)^2+2(x+1)+4]$
$=(x-1)(x^2+4x+7)$
S
7 tháng 9 2021
a) \(3x^2+xy-4y^2=3x^2-3xy+4xy-4y^2\)
\(=3x(x-y)+4y(x-y)=(3x+4y)(x-y)\)
b)\(x^8-5x^4+4=x^8-x^4-4x^4+4\)
\(=x^2(x^4-1)-4(x^4-1)=(x^2-4)(x^4-1)\)
\(=(x-2)(x+2)(x^2-1)(x^2+1)=(x-2)(x+2)(x-1)(x+1)(x^2+1)\)
c)\(x^3+3x^2+3x-7=x^3+3x^2+3x+1-8\)
\(\left(x+1\right)^3-\sqrt{2}^3=\left(x+1-\sqrt[]{2}\right)\left(\left(x+1\right)^2+2\sqrt{2}x+2\right)\)
3 tháng 9 2021
e: \(x^2+6x+9-y^2\)
\(=\left(x+3\right)^2-y^2\)
\(=\left(x+3-y\right)\left(x+3+y\right)\)
f: \(x^2-2x+7x-14\)
\(=x\left(x-2\right)+7\left(x-2\right)\)
=(x-2)(x+7)
h: \(5x^2-10xy+5y^2-20\)
\(=5\left(x^2-2xy+y^2-4\right)\)
\(=5\left(x-y-2\right)\left(x-y+2\right)\)
3 tháng 9 2021
a: \(3x^4-6x^3+2x^2=x^2\left(3x^2-6x+2\right)\)
b: \(x^3y+12x^2y+36xy=xy\left(x^2+12x+36\right)=xy\left(x+6\right)^2\)
c: \(x^3y-9xy^3=xy\left(x^2-9y^2\right)=xy\left(x-3y\right)\left(x+3y\right)\)
d: \(x^2y^2-2xy^2+y^2=y^2\left(x-1\right)^2\)
4 tháng 9 2021
\(2x^2+3x-27\)
\(=2x^2+9x-6x-27\)
\(=x\left(2x+9\right)-3\left(2x+9\right)\)
\(=\left(2x+9\right)\left(x-3\right)\)
CM
26 tháng 7 2019
a) Đặt 3x làm nhân tử chung ta được 3x( 8 x 4 + 3 x 2 + 6x).
Thực hiện phép chia được thương 8 x 4 - 3 x 2 + 6x.
b) Thực hiện phép chia từng đơn thức được kết quả 5 3 x 2 + 6 x + 13 2 .
PB
1
21 tháng 10 2021
1: \(-x^2+2x+8\)
\(=-\left(x^2-2x-8\right)\)
\(=-\left(x-4\right)\left(x+2\right)\)
2: \(2x^2-3x+1=\left(x-1\right)\left(2x-1\right)\)