VH
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PV
1
2 tháng 3 2022
-Đặt \(t=\left(x^2-x+1\right)\)
\(\left(x^2-x+1\right)^2-5x\left(x^2-x+1\right)+4x^2\)
\(=t^2-5xt+4x^2\)
\(=t^2-4xt-xt+4x^2\)
\(=t\left(t-4x\right)-x\left(t-4x\right)\)
\(=\left(t-4x\right)\left(t-x\right)\)
\(=\left(x^2-x+1-4x\right)\left(x^2-x+1-x\right)\)
\(=\left(x^2-5x+1\right)\left(x^2-2x +1\right)\)
\(=\left(x^2-5x+1\right)\left(x-1\right)^2\)
21 tháng 8 2021
\(\left(x^2+x+1\right)\left(x^2+x+5\right)-21=x^4+x^3+5x^2+x^3+x^2+5x+x^2+x+5-21=x^4+2x^3+7x^2+6x-16=\left(x-1\right)\left(x+2\right)\left(x^2+x+8\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 8 2021
\(=\left(x^2+x+1\right)\left(x^2+x+1+4\right)-21\)
\(=\left(x^2+x+1\right)^2+4\left(x^2+x+1\right)-21\)
\(=\left(x^2+x+1\right)^2-3\left(x^2+x+1\right)+7\left(x^2+x+1\right)-21\)
\(=\left(x^2+x+1\right)\left(x^2+x-2\right)+7\left(x^2+x-2\right)\)
\(=\left(x^2+x-2\right)\left(x^2+x+8\right)\)
\(=\left(x-1\right)\left(x-2\right)\left(x^2+x+8\right)\)
16 tháng 11 2021
\(=3\left(x-1\right)+x\left(x-1\right)\)
\(=\left(x-1\right)\left(x+3\right)\)
PT
1
22 tháng 11 2021
\(1,\\ 12x^6y^3:4x^3y=3x^3y^2\\ \left(x+1\right)\left(x^2-x+1\right)=x^3+1\\ 2x^2y\left(x^2+3xy\right)=3x^4y+6x^3y^2\\ 2,\\ a,=2xy\left(2x+3y-4\right)\\ b,=\left(x-3\right)\left(x+y\right)\\ c,=\left(x-2\right)\left(x+2\right)+y\left(x-2\right)=\left(x+y+2\right)\left(x-2\right)\\ d,=x^2-2x-5x+10=\left(x-2\right)\left(x-5\right)\\ 3,\\ a,\Leftrightarrow x^2-x^2+2x=2\\ \Leftrightarrow2x=2\Leftrightarrow x=1\\ b,\Leftrightarrow\left(x-2\right)\left(x-2+1\right)=0\\ \Leftrightarrow\left(x-2\right)\left(x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)
CM
22 tháng 12 2019
a) Áp dụng HĐT 1 thu được ( 2 x + y ) 2 .
b) Áp dụng HĐT 3 với A = 2x + l; B = x - l thu được
[(2x +1) + (x -1)] [(2x +1) - (x -1)] rút gọn thành 3x(x + 2).
c) Ta có: 9 - 6x + x 2 - y 2 = ( 3 - x ) 2 - y 2 = (3 - x - y)(3 -x + y).
d) Ta có: -(x + 2) + 3( x 2 - 4) = -{x + 2) + 3(x + 2)(x - 2)
= (x + 2) [-1 + 3(x - 2)] = (x + 2)(3x - 7).
GL
1 tháng 7 2023
\(\Leftrightarrow x^3-2x^2+x^2-2x+x-2\)
\(\Leftrightarrow x^2\left(x-2\right)+x\left(x-2\right)+\left(x-2\right)\)
\(\Leftrightarrow\left(x-2\right)\left(x^2+x+1\right)\)
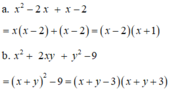
Đặt \(a=x^2+x+1\)\(\Rightarrow\)\(a+1=x^2+x+2\)
Ta có: \(\left(x^2+x+1\right)\left(x^2+x+2\right)-6=a.\left(a+1\right)-6\)
\(=a^2+a-6\)
\(=\left(a^2-2a\right)+\left(3a-6\right)\)
\(=a.\left(a-2\right)+3\left(a-2\right)\)
\(=\left(a+3\right).\left(a-2\right)\)
\(=\left(x^2+x+1+3\right).\left(x^2+x+1-2\right)\)
\(=\left(x^2+x+4\right)\left(x^2+x-1\right)\)
Chúc bn hok tốt
( x2 + x + 1 )( x2 + x + 2 ) - 6 (*)
Đặt x2 + x + 1 = t
(*) = t( t + 1 ) - 6
= t2 + t - 6
= t2 - 2t + 3t - 6
= t( t - 2 ) + 3( t - 2 )
= ( t - 2 )( t + 3 )
= ( x2 + x + 1 - 2 )( x2 + x + 1 + 3 )
= ( x2 + x - 1 )( x2 + x + 4 )