VL
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
VL
1
26 tháng 10 2021
Câu 1:
1: \(\overrightarrow{OM}=\dfrac{\overrightarrow{OA}+\overrightarrow{OD}}{2}=\dfrac{\overrightarrow{BO}+\overrightarrow{OA}}{2}=\dfrac{\overrightarrow{BA}}{2}=-\dfrac{1}{2}\overrightarrow{AB}\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
\(\overrightarrow{BD}=2\cdot\overrightarrow{BO}=-2\cdot\overrightarrow{OB}\)
nên y=-2
2: \(2\cdot\overrightarrow{ON}=\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{DO}+\overrightarrow{OC}=\overrightarrow{DC}=\overrightarrow{AB}\)
Vậy: Các vecto u thỏa mãn là vecto DC và vecto AB
26 tháng 10 2021
Câu 5:
\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}\)
\(=\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{BC}\)
\(=\overrightarrow{AB}-\dfrac{2}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\)
\(=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\)
26 tháng 10 2021
\(c5\) \(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}=\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{BC}\)
\(=\overrightarrow{AB}+\dfrac{2}{3}\left(\overrightarrow{BA}+\overrightarrow{AC}\right)=\overrightarrow{AB}-\dfrac{2}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\)
\(=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\left(đpcm\right)\)
\(c4:\Rightarrow\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{GG'}\)
\(\overrightarrow{G'A'}+\overrightarrow{G'B'}+\overrightarrow{G'C'}=\overrightarrow{GG'}\)\(\Leftrightarrow\overrightarrow{G'A}+\overrightarrow{AA'}+\overrightarrow{G'B}+\overrightarrow{B'B}+\overrightarrow{G'C}+\overrightarrow{CC'}=\overrightarrow{GG'}\)
\(\Leftrightarrow\overrightarrow{AA'}+\overrightarrow{BB'}+\overrightarrow{CC'}=3\overrightarrow{GG'}\)\(\left(dpcm\right)\)
\(c3:a,\) \(2\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}=2\overrightarrow{IA}+2\overrightarrow{IM}=2\overrightarrow{MI}+2\left(\overrightarrow{IM}+\overrightarrow{MI}\right)=2.\overrightarrow{0}=\overrightarrow{0}\left(đpcm\right)\)
\(b,2\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}\)
\(=2\left(\overrightarrow{OI}+\overrightarrow{IA}\right)+\overrightarrow{OI}+\overrightarrow{IB}+\overrightarrow{OI}+\overrightarrow{IC}\)
\(=4\overrightarrow{OI}+2\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}\)\(=4\overrightarrow{OI}+\overrightarrow{0}=4\overrightarrow{OI}\left(đpcm\right)\)
\(c2:\) \(\left\{{}\begin{matrix}3AH=2AB\\3AK=AC\\4BM=3MC\\\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\overrightarrow{AB}=\dfrac{3}{2}\overrightarrow{AH}\\\overrightarrow{AC}=3\overrightarrow{AK}\\\overrightarrow{BM}=\dfrac{3}{7}\overrightarrow{BC}\Rightarrow\overrightarrow{BC}=\dfrac{7}{3}\overrightarrow{BM}\end{matrix}\right.\)
\(\Rightarrow\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}=3\overrightarrow{AK}-\dfrac{3}{2}\overrightarrow{AH}\)
\(\Rightarrow\dfrac{7}{3}\overrightarrow{BM}=3\overrightarrow{AK}-\dfrac{3}{2}\overrightarrow{AH}\Leftrightarrow\overrightarrow{BM}=\dfrac{9}{7}\overrightarrow{AK}-\dfrac{9}{14}\overrightarrow{AH}\)
SG
1
15 tháng 4 2017
a) Bảng phân bố tần số (về tuổi thọ bóng đèn điện) có thể viết dưới dạng như sau:
Số trung bình về tuổi thọ của bóng đèn trong bảng phân bố trên là:
.(3x1150 + 6x1160 + 12x1170 + 6x1180 + 3x1190)
= 1170.
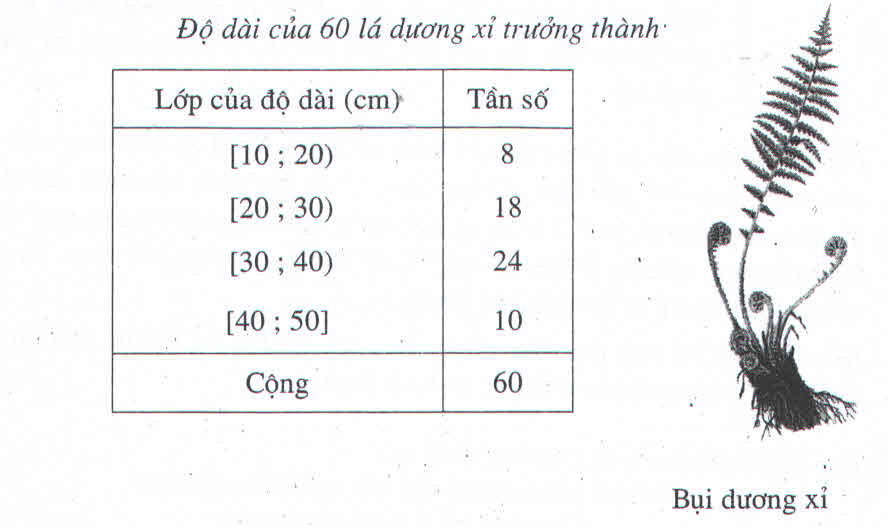
b) Số trung bình về chiều dài lá cây dương xỉ trong bài tập 2 trong là:
.(8x15 + 18x25 + 24x35 + 10x45) = 31 (cm).
Q
29 tháng 5 2017
a) Phương sai và độ lệch chuẩn trong bài tập 1. Bảng phân bố tần số viết lại là
Số trung bình: \(\overline{x} = 1170\)
Phương sai: \(S_{x}^{2}=\frac{1}{30}(3x1150^{2}+6x1160^{2}+12x1170^{2}+6x1180^{2}+3x1190^{2})-1170^{2} = 120\)
Độ lệch chuẩn: Sx.= \(\sqrt{S_{x}^{2}}=\sqrt{120} ≈ 10,9545\)
b) Phương sai và độ lệch chuẩn, bảng thống kê trong bài tập 2 \(\S 1.\)
\(S_{x}^{2}=\frac{1}{60}(8x15^{2}+18x25^{2}+24x35^{2}+10x45^{2}) - 312 = 84 \)
Sx ≈ 9,165.
15 tháng 8 2023
11:
a: \(BD=AC=\sqrt{\left(3a\right)^2+\left(4a\right)^2}=5a\)
|vecto AB+vecto AD|
=|vecto AB+vecto BC|
=|vecto AC|
=5a
b: Gọi M là trung điểm của BC
=>BM=CM=BC/2=2a
\(AM=\sqrt{AB^2+BM^2}=a\sqrt{13}\)
Xét ΔABC có AM là trung tuyến
nên vecto AB+vecto AC=2*vecto AM
=>|vecto AB+vecto AC|=2|vecto AM|
=>\(\left|\overrightarrow{AB}+\overrightarrow{AC}\right|=2\cdot AM=2a\sqrt{13}\)
16 tháng 8 2023
12:
a: Gọi M là trung điểm của BC
trên tia đối của tia MA, lấy D sao cho M là trung điểm của AD
Xét tứ giác ABDC có
M là trung điểm chung của AD và BC
góc BAC=90 độ
=>ABDC là hình chữ nhật
=>\(\overrightarrow{AB}+\overrightarrow{AC}=\overrightarrow{AD}\)
=>\(\overrightarrow{v}=\overrightarrow{AD}\)
b: \(\left|\overrightarrow{v}\right|=\left|\overrightarrow{AD}\right|=AD=2\cdot AM=2\cdot\dfrac{BC}{2}=BC=\sqrt{\left(6a\right)^2+\left(8a\right)^2}=10a\)
16 tháng 8 2023
hình như đó đâu phải là câu 12 đâu ạ 🤔🤔🤔 trong đề câu 12 có chi tiết nào liên quan tới M đâu 🤔
Câu 1:
1: \(\overrightarrow{OM}=\dfrac{\overrightarrow{OA}+\overrightarrow{OD}}{2}=\dfrac{\overrightarrow{BO}+\overrightarrow{OA}}{2}=\dfrac{\overrightarrow{BA}}{2}=-\dfrac{1}{2}\overrightarrow{AB}\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
\(\overrightarrow{BD}=2\cdot\overrightarrow{BO}=-2\cdot\overrightarrow{OB}\)
nên y=-2
2: \(2\cdot\overrightarrow{ON}=\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{DO}+\overrightarrow{OC}=\overrightarrow{DC}=\overrightarrow{AB}\)
Vậy: Các vecto u thỏa mãn là vecto DC và vecto AB