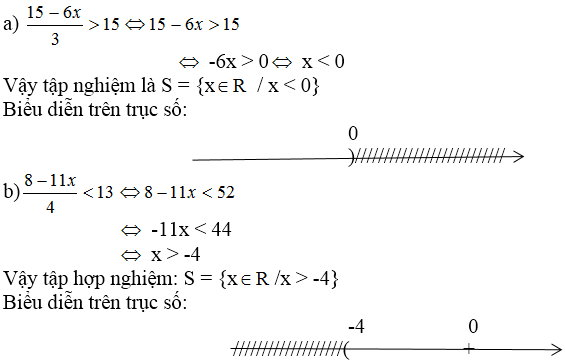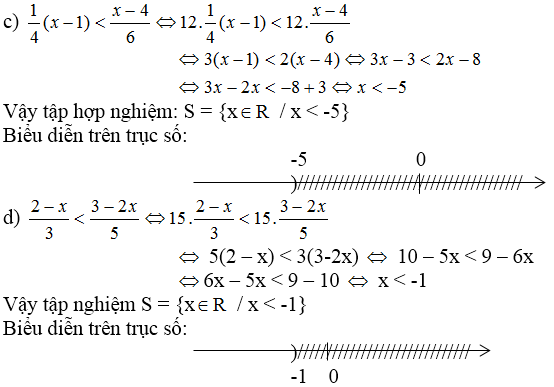Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a)<=> (x^2-3)^2 +1 <=0 => vô nghiệm biểu diễn gạch hết
b)\(\Leftrightarrow\left(3x-4\right)\left(2x-3\right)\ge0\) \(\Rightarrow\left[{}\begin{matrix}x\ge\dfrac{3}{2}\\x\le\dfrac{4}{3}\end{matrix}\right.\) Trên trục số gạch phần giữa 4/3 và 3/2 chú ý 4/3 <3/2
c)
x^2 (x-4) <11x-30
x^3 -4x^2-11x +30 <0
(x^3 -8) -4x(x-2) -19(x-2)<0
(x-2)(x^2+2x+4-4x-19)<0
(x-2)(x^2-2x+15)<0
(x-2)(x-5)(x-3)<0
\(\left[{}\begin{matrix}x< -3\\2< x< 5\end{matrix}\right.\)
Biểu diễn gạch hết đoạn từ -3 đến 2 và >= 5

a/ \(\left(x+\dfrac{1}{9}\right)\left(2x-5\right)< 0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x+\dfrac{1}{9}>0\\2x-5< 0\end{matrix}\right.\\\left\{{}\begin{matrix}x+\dfrac{1}{9}< 0\\2x-\dfrac{1}{5}>0\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x>-\dfrac{1}{9}\\x< \dfrac{5}{2}\end{matrix}\right.\\\left\{{}\begin{matrix}x< -\dfrac{1}{9}\\x>\dfrac{5}{2}\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow\dfrac{-1}{9}< x< \dfrac{5}{2}\)
Biểu diễn:
0 -1/9 5/2
b/ \(\left(4x-1\right)\left(x^2+12\right)\left(-x+4\right)>0\)
\(\Leftrightarrow\left(4x-1\right)\left(x^2+12\right)\left(4-x\right)>0\)
vì \(x^2+12\ge12>0\) nên:
\(bpt\Leftrightarrow\left(4x-1\right)\left(4-x\right)>0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}4x-1>0\\4-x>0\end{matrix}\right.\\\left\{{}\begin{matrix}4x-1< 0\\4-x< 0\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x>\dfrac{1}{4}\\x< 4\end{matrix}\right.\\\left\{{}\begin{matrix}x< \dfrac{1}{4}\\x>4\end{matrix}\right.\end{matrix}\right.\)\(\Rightarrow\dfrac{1}{4}< x< 4\)
Vậy..............
biểu diễn:........(tự biểu diễn nha bn)
c/ \(x^2-6x+9< 0\)
\(\Leftrightarrow\left(x-3\right)^2< 0\) (vô lí)
=> bpt vô nghiệm
\(a.\left(x+\dfrac{1}{9}\right)\left(2x-5\right)< 0\)
Lập bảng xét dấu , ta có :
x x + 1/9 Tích số 2x - 5 -1/9 5/2 0 0 0 0 - + + - - + + - + Vậy , nghiệm của BPT : \(\dfrac{-1}{9}< x< \dfrac{5}{2}\)
b) ( 4x - 1)( x2 + 12)( 4 - x) > 0
Do : x2 + 12 > 0
⇒ ( 4x - 1)( 4 - x) > 0
Lập bảng xét dấu , ta có :
x 4x - 1 4 - x Tích số 1/4 4 0 0 0 0 - + + + + - - + - Vậy , nghiệm của BPT : \(\dfrac{1}{4}< x< 4\)
c) x2 - 6x + 9 < 0
⇔ ( x - 3)2 < 0 ( vô lý )
Vậy , BPT vô nghiệm
P/s : Bạn tự biểu diễn nhé.

a) 4x - 5 >0
\(\Leftrightarrow\)4x>5
\(\Leftrightarrow\)x>\(\frac{5}{4}\)
vậy s={x/x>\(\frac{5}{4}\)}
b) −23x−4>0
\(\Leftrightarrow\)-23x>4
\(\Leftrightarrow\)x<\(\frac{4}{23}\)
vậy s={x/x<\(\frac{4}{23}\)}
tối rồi nên lúc khác làm tiếp

a: \(\Leftrightarrow x^2-2x+1< x^2+3x\)
=>-5x<-1
hay x>1/5
b: \(\Leftrightarrow x^2-4x< x^2-4\)
=>-4x<-4
hay x>1
c: \(\Leftrightarrow2x+3< 6-3+4x\)
=>2x+3<4x+3
=>-2x<0
hay x>0
d: =>-2-7x>3+2x-5+6x
=>-7x-2>8x-2
=>-15x>0
hay x<0

nhiều thế
a) \(\frac{5x-2}{2}\ge\frac{3-x}{3}\Leftrightarrow\frac{3\left(5x-2\right)}{6}\ge\frac{2\left(3-x\right)}{6}\Leftrightarrow15x-6\ge6-2x\Leftrightarrow x\ge\frac{12}{17}\)
0 [ 12/17