Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Vận tốc của chất điểm:
\(v\left(t\right)=s'\left(t\right)=3t^2-6t+9=3\left(t-1\right)^2+6\ge6\)
Dấu "=" xảy ra khi \(t-1=0\Rightarrow t=1s\)
Dạ em cảm ơn rất nhiều ạ, nhưng nếu được thầy có thể giải thích giúp em làm sao ra đc :S'(t) ạ ?

\(\Leftrightarrow\left(sin^2x+cos^2x\right)^2-2sin^2x.cos^2x=2cos2x\)
\(\Leftrightarrow1-\frac{1}{2}sin^22x=2cos2x\)
\(\Leftrightarrow2-\left(1-cos^22x\right)=4cos2x\)
\(\Leftrightarrow cos^22x-4cos2x+1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=2+\sqrt{3}>1\left(l\right)\\cos2x=2-\sqrt{3}\end{matrix}\right.\)
\(\Leftrightarrow x=\pm\frac{1}{2}arccos\left(2-\sqrt{3}\right)+k\pi\)

\(\sqrt[n]{y}=4x+1\)
\(y^{\dfrac{1}{n}}=4x+1\)
đạo cấp 1
\(\dfrac{1}{n}y^{\left(\dfrac{1}{n}-1\right)}=\dfrac{1}{n}\sqrt[n]{y^{\left(1-n\right)}}=4\)
thay y=(4x+1)^n vào
\(\dfrac{1}{n}\sqrt[n]{\left(4x+1\right)^{n\left(1-n\right)}}=\dfrac{1}{n}\left(4x+1\right)^{\left(1-n\right)}\)
từ đó: \(y'=\dfrac{4}{\dfrac{1}{n}\left(4x+1\right)^{\left(1-n\right)}}=4.n\left(4x+1\right)^{n-1}\)
Có đúng không: cấp n có thể phải làm lấy vài cái--> quy luật nào đó

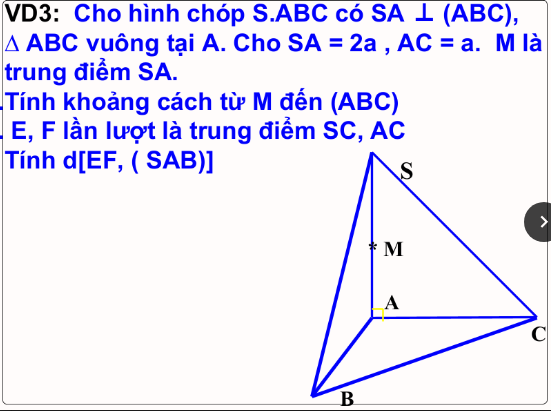
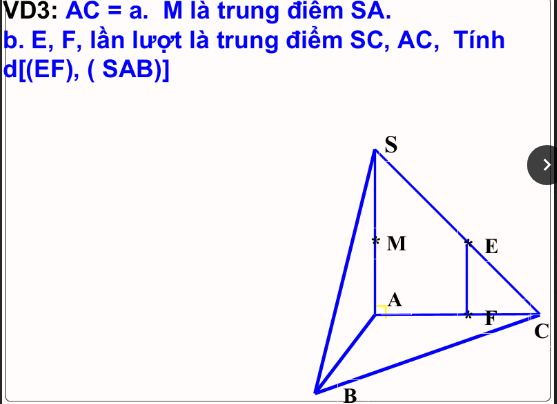
Khoảng cách từ M để ABC bằng MA
Khoảng cách từ EF đến SAB bằng EM = AF

13 . b ) SH \(\perp\left(ABCD\right)\Rightarrow SH\perp DI\) .
Dễ dàng c/m : DI \(\perp HC\) . Suy ra : \(DI\perp\left(SHC\right)\Rightarrow DI\perp SC\) ( đpcm )
Thấy : \(\left(SBC\right)\cap\left(ABCD\right)=BC\)
C/m : SB \(\perp BC\) . Thật vậy : \(BC\perp AB;BC\perp SH\Rightarrow BC\perp\left(SAB\right)\Rightarrow BC\perp SB\)
Có : \(AB\perp BC\) nên : \(\left(\left(SBC\right);\left(ABCD\right)\right)=\left(SB;AB\right)=\widehat{SBA}=60^o\)



a/ \(y=cos^34x\)
\(y'=3cos^24x.\left(cos4x\right)'=-12.cos^24x.sin4x\)
b/ \(y=sin^23x\)
\(y'=2sin3x.\left(sin3x\right)'=6sin3x.cos3x=3sin6x\)