Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\frac{A}{3x-1}=\frac{12x^2+4x}{9x^2-1}\)
\(\Rightarrow A.\left(9x^2-1\right)=\left(3x-1\right)\left(12x^2+4x\right)\)
\(\Rightarrow A.\left(3x-1\right)\left(3x+1\right)=\left(3x-1\right)4x\left(3x+1\right)\)
\(\Rightarrow A=4x\)

d: \(\Leftrightarrow\dfrac{\left(x+2\right)^2}{\left(x+2\right)\left(x-2\right)}=\dfrac{\left(x+1\right)\left(x+2\right)}{A}\)
hay A=x-2

a)\(\frac{x^2+5x+4}{x^2-1}=\frac{A}{x^2-2x+1}\)
\(\Leftrightarrow\frac{\left(x+1\right)\left(x+4\right)}{\left(x+1\right)\left(x-1\right)}=\frac{A}{\left(x-1\right)^2}\)
\(\Leftrightarrow\frac{x+4}{x-1}=\frac{A}{\left(x-1\right)^2}\). Nhân 2 vế ở tử với x-1 ta có:
\(x+4=\frac{A}{x-1}\Leftrightarrow A=\left(x-1\right)\left(x+4\right)=x^2+3x-4\)
b)\(\frac{x^2-3x}{2x^2-7x+3}=\frac{x^2+4x}{A}\)
\(\Leftrightarrow\frac{x\left(x-3\right)}{\left(2x-1\right)\left(x-3\right)}=\frac{x\left(x+4\right)}{A}\)
\(\Leftrightarrow\frac{x}{2x-1}=\frac{x\left(x+4\right)}{A}\).Nhân 2 vế ở mẫu với x ta có:
\(2x-1=\frac{x+4}{A}\)\(\Leftrightarrow\left(2x-1\right)\left(x+4\right)=A\Leftrightarrow A=2x^2+7x-4\)

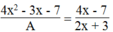 ⇒
4
x
2
-
3
x
-
7
2
x
+
3
=
A
4
x
-
7
⇒
4
x
2
-
3
x
-
7
2
x
+
3
=
A
4
x
-
7
⇒ 4 x 2 + 4 x - 7 x - 7 2 x + 3 = A 4 x - 7
⇒ [4x(x + 1) – 7(x + 1)](2x+ 3) = A(4x - 7)
⇒ (x + 1)(4x – 7)(2x + 3) = A(4x – 7)
⇒ A = (x + 1)(2x + 3) = 2 x 3 + 3 x + 2 x + 3 = 2 x 2 + 5 x + 3
Vậy 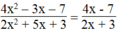

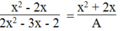 ⇒
x
2
-
2
x
.
A
=
2
x
2
-
3
x
-
2
x
2
+
2
x
⇒
x
2
-
2
x
.
A
=
2
x
2
-
3
x
-
2
x
2
+
2
x
⇒ x x - 2 . A = 2 x 2 - 4 x + x - 2 . x x + 2
⇒ x x - 2 . A = 2 x x - 2 + x - 2 . x x + 2
⇒ x(x – 2).A = (x – 2)(2x + 1).x.(x + 2)
⇒ A = (2x + 1)(x + 2) = 2 x 2 + 4 x + x + 2 = 2 x 2 + 5 x + 2
Vậy 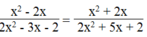

A = 4x nha
A=4x đúng đó