Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lấy M là trung điểm của CD
\(AC^2-AD^2=BC^2-BD^2\)
<=> \(\left(\overrightarrow{AC}-\overrightarrow{AD}\right)\left(\overrightarrow{AC}+\overrightarrow{AD}\right)=\left(\overrightarrow{BC}-\overrightarrow{BD}\right)\left(\overrightarrow{BC}+\overrightarrow{BD}\right)\)
<=> \(2.\overrightarrow{DC}.\overrightarrow{AM}=2.\overrightarrow{DC}.\overrightarrow{BM}\)
<=> \(2.\overrightarrow{DC}.\left(\overrightarrow{AM}-\overrightarrow{BM}\right)=0\)
<=> \(2.\overrightarrow{DC}.\overrightarrow{AB}=0\)
<=> DC vuông góc với AB
1/Tìm x biết: (1/2x-1004)^2008 = (1/2x-1004)^2006
2/Cho tam giác ABC cân tại A. D là 1 điểm nằm trong tam giác, biết góc ADB > góc ADC. Chứng minh: DB<DC
giúp e với

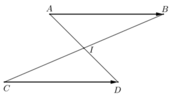
Gọi trung điểm của AD là I, trung điểm BC là J.
Khi đó ta có: 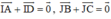
Mà theo quy tắc ba điểm ta có:
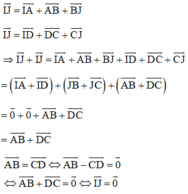
⇔ I ≡ J hay trung điểm AD và BC trùng nhau (đpcm)

Với 4 điểm A, B, C, D ta có: \(\overrightarrow {AB} = \overrightarrow {CD} \) khi và chỉ khi tứ giác ABDC là hình bình hành
Theo tính chất của hình bình hành thì giao điểm của hai đường chéo là trung điểm của mỗi đường và ngược lại.
Nói cách khác: trung điểm của hai đoạn thẳng AD và BC trùng nhau.
Vậy ta có điều phải chứng minh.
Với 4 điểm A, B, C, D ta có: \(\overrightarrow {AB} = \overrightarrow {CD} \) khi và chỉ khi tứ giác ABDC là hình bình hành
Theo tính chất của hình bình hành thì giao điểm của hai đường chéo là trung điểm của mỗi đường và ngược lại.
Nói cách khác: trung điểm của hai đoạn thẳng AD và BC trùng nhau.
Vậy ta có điều phải chứng minh.

Nếu \(\overrightarrow{AB}=\overrightarrow{CD}\) thì AD và BC có trung điểm trùng nhau. Gọi I là trung điểm của AD ta chứng minh I cũng là trung điểm của BC.
Theo quy tắc của ba điểm của tổng, ta có
\(\overrightarrow{AB}=\overrightarrow{AI}+\overrightarrow{IB};\overrightarrow{CD}=\overrightarrow{CI}+\overrightarrow{ID}\)
Vì \(\overrightarrow{AB}=\overrightarrow{CD}\) nên \(\overrightarrow{AI}+\overrightarrow{IB}=\overrightarrow{CI}+\overrightarrow{ID}\)
\(\Rightarrow\overrightarrow{AI}-\overrightarrow{ID}=\overrightarrow{CI}-\overrightarrow{IB}\)
\(\Rightarrow\overrightarrow{AI}+\overrightarrow{DI}=\overrightarrow{CI}+\overrightarrow{BI}\left(1\right)\)
Vì I là trung điểm của AD nên \(\overrightarrow{AI}+\overrightarrow{DI}=\overrightarrow{0}\left(2\right)\)
Từ (1) và (2) suy ra \(\overrightarrow{CI}+\overrightarrow{BI}=\overrightarrow{0}\left(3\right)\)
Từ (3) ta có chung điểm I, ta chứng minh \(\overrightarrow{AB}=\overrightarrow{CD}\)
I là trung điểm AD \(\Rightarrow\overrightarrow{AI}+\overrightarrow{DI}=\overrightarrow{0}\Rightarrow\overrightarrow{AI}-\overrightarrow{ID}=\overrightarrow{0}\)
I là trung điểm BC \(\Rightarrow\overrightarrow{CI}+\overrightarrow{BI}=0\Rightarrow\overrightarrow{CI}-\overrightarrow{IB}=\overrightarrow{0}\)
Suy ra \(\overrightarrow{AI}-\overrightarrow{ID}=\overrightarrow{CI}-\overrightarrow{IB}\)
\(\Rightarrow\overrightarrow{AI}+\overrightarrow{IB}=\overrightarrow{CI}+\overrightarrow{ID}\Rightarrow\overrightarrow{AB}=\overrightarrow{CD}\)

a) ta có : \(\overrightarrow{AB}-\overrightarrow{CD}=\overrightarrow{AB}+\overrightarrow{DC}=\overrightarrow{AP}+\overrightarrow{PQ}+\overrightarrow{QB}+\overrightarrow{DP}+\overrightarrow{PQ}+\overrightarrow{QC}\)
\(=2\overrightarrow{PQ}+\left(\overrightarrow{AP}+\overrightarrow{DP}\right)+\left(\overrightarrow{QB}+\overrightarrow{QC}\right)=2\overrightarrow{PQ}\) ..................(1)
\(\overrightarrow{AC}-\overrightarrow{BD}=\overrightarrow{AC}+\overrightarrow{DB}=\overrightarrow{AP}+\overrightarrow{PQ}+\overrightarrow{QC}+\overrightarrow{DP}+\overrightarrow{PQ}+\overrightarrow{QB}\)\(=2\overrightarrow{PQ}+\left(\overrightarrow{AP}+\overrightarrow{DP}\right)+\left(\overrightarrow{QB}+\overrightarrow{QC}\right)=2\overrightarrow{PQ}\) ..................(2)
từ (1) và (2) ta có : \(\overrightarrow{AB}-\overrightarrow{CD}=\overrightarrow{AC}-\overrightarrow{BD}=2\overrightarrow{PQ}\left(đpcm\right)\)

không cần đk là a,b,c là số thực cũng được @@
Sử dụng bất đẳng thức phụ \(x^2+y^2\ge2xy\)
chứng minh : \(x^2+y^2\ge2xy< =>\left(x-y\right)^2\ge0\)*đúng*
Áp dụng vào bài toán ta được :
\(2.LHS\ge ab+bc+ca+ab+bc+ca=2\left(ab+bc+ca\right)\)
\(< =>LHS\ge ab+bc+ca\)
Dấu = xảy ra \(< =>a=b=c\)

a) ta có : \(\overrightarrow{AB}+\overrightarrow{CD}=\overrightarrow{AD}+\overrightarrow{DB}+\overrightarrow{CB}+\overrightarrow{BD}=\overrightarrow{AD}+\overrightarrow{CB}\left(đpcm\right)\)
b) ta có : \(\overrightarrow{AC}+\overrightarrow{BD}=\overrightarrow{AI}+\overrightarrow{IJ}+\overrightarrow{JC}+\overrightarrow{BI}+\overrightarrow{IJ}+\overrightarrow{JD}\)
\(=2\overrightarrow{IJ}+\left(\overrightarrow{AI}+\overrightarrow{BI}\right)+\left(\overrightarrow{JC}+\overrightarrow{JD}\right)=2\overrightarrow{IJ}\) .........(1)
ta có : \(\overrightarrow{AD}+\overrightarrow{BC}=\overrightarrow{AI}+\overrightarrow{IJ}+\overrightarrow{JD}+\overrightarrow{BI}+\overrightarrow{IJ}+\overrightarrow{JC}\)
\(=2\overrightarrow{IJ}+\left(\overrightarrow{AI}+\overrightarrow{BI}\right)+\left(\overrightarrow{JC}+\overrightarrow{JD}\right)=2\overrightarrow{IJ}\) .........(2)
từ (1) và (2) ta có \(2\overrightarrow{IJ}=\overrightarrow{AC}+\overrightarrow{BD}=\overrightarrow{AD}+\overrightarrow{BC}\left(đpcm\right)\)
c) ta có : \(\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}=\overrightarrow{0}\)
\(2\overrightarrow{OI}+2\overrightarrow{OJ}=\overrightarrow{0}\Leftrightarrow\overrightarrow{OI}+\overrightarrow{OJ}=\overrightarrow{0}\)
\(\Rightarrow O\) là trung điểm \(IJ\)

A B C D P M
a) \(\overrightarrow{MP}.\overrightarrow{BC}=\dfrac{1}{2}\left(\overrightarrow{MA}+\overrightarrow{MD}\right).\left(\overrightarrow{BM}+\overrightarrow{MC}\right)\)
\(=\dfrac{1}{2}\left(\overrightarrow{MA}.\overrightarrow{BM}+\overrightarrow{MA}.\overrightarrow{MC}+\overrightarrow{MD}.\overrightarrow{BM}+\overrightarrow{MD}.\overrightarrow{MC}\right)\)
\(=\dfrac{1}{2}\left(\overrightarrow{MA}.\overrightarrow{BM}+\overrightarrow{MA}.\overrightarrow{MC}-\overrightarrow{MB}.\overrightarrow{MD}+\overrightarrow{MD}.\overrightarrow{MC}\right)\)
\(=\dfrac{1}{2}\left(\overrightarrow{MA}.\overrightarrow{BM}+\overrightarrow{MD}.\overrightarrow{MC}\right)\)
\(=\dfrac{1}{2}\left(0+0\right)=0\) (vì \(AC\perp BD\) nên \(\overrightarrow{MA}.\overrightarrow{BM}=0;\overrightarrow{MD}.\overrightarrow{MC}=0\)).
Vậy \(\overrightarrow{MP}.\overrightarrow{BC}=0\) nên \(MP\perp BC\).
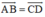
Xét tứ giác ABDC có
AD cắt BC tại trung điểm của mỗi đường
nên ABDC là hình bình hành
Suy ra: vecto AC=vecto BD