Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

3x^2 - 5x - 2 = 0
(a = 3; b = -5; c = -2)
ta có x1, x2 là nghiệm của pt nên : x1 + x2 = -b/a = -(-5)/3 = 5/3
vậy_
Áp dụng hệ thức Vi - ét ta có : x1 + x2 = \(-\frac{b}{a}=-\frac{-5}{3}=\frac{5}{3}\)
Hoặc chưa học ở lớp 8 thì \(3x^2-5x-2=3x^2-6x+x-2=3x\left(x-2\right)+\left(x-2\right)\)
\(=\left(3x+1\right)\left(x-2\right)=0\Leftrightarrow\orbr{\begin{cases}x1=-\frac{1}{3}\\x2=2\end{cases}}\Leftrightarrow x1+x2=-\frac{1}{3}+2=\frac{5}{3}\)
cho x1, x2, x3 là 3 nghiệm của phương trình x^3-19x-30=0. Giá trị của Bt B= x1^2 + x2^2 + x3^2 là...

x3 - 19x - 30 = 0
<=> x3 - 5x2 + 5x2 - 25x + 6x - 30 = 0
<=> x2( x - 5 ) + 5x( x - 5 ) + 6( x - 5 ) = 0
<=> ( x - 5 )( x2 + 5x + 6 ) = 0
<=> ( x - 5 )( x2 + 3x + 2x + 6 ) = 0
<=> ( x - 5 )[ x( x + 3 ) + 2( x + 3 ) ] = 0
<=> ( x - 5 )( x + 3 )( x + 2 ) = 0
đến đây dễ rồi :)
\(x^3-19x-30=0\)
\(\Leftrightarrow\left(x-5\right)\left(x+2\right)\left(x+3\right)=0\)
\(\Leftrightarrow\hept{\begin{cases}x-5=0\\x+2=0\\x+3=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=5\\x=-2\\x=-3\end{cases}}}\)
Vậy B=x12+x22+x32
B=52+(-2)2+(-3)2
B=25+4+9
B=38
#H

Ta có hằng đẳng thức:
\(a^3+b^3+c^3-3abc=\left(a+b+c\right)\left(a^2+b^2+c^2-ab-bc-ca\right)\)
Ta thấy \(\left(x-1\right)+\left(x-2\right)+\left(3-2x\right)=0\)
do đó \(\left(x-1\right)^3+\left(x-2\right)^3+\left(3-2x\right)^3=3\left(x-1\right)\left(x-2\right)\left(3-2x\right)\)
suy ra \(\left(x-1\right)\left(x-2\right)\left(3-2x\right)=0\)
\(\Leftrightarrow\hept{\begin{cases}x_1=1\\x_2=2\\x_3=\frac{3}{2}\end{cases}}\)
\(S=\frac{29}{4}\).

Đa thức \(P\left(x\right)=x^3-3x+1\)có ba nghiệm phân biệt \(x_1,x_2,x_3\) có:
\(\hept{\begin{cases}x_1+x_2+x_3=0\\x_1x_2+x_2x_3+x_3x_1=-3\\x_1x_2x_3=-1\end{cases}}\)
\(E=Q\left(x_1\right)Q\left(x_2\right)Q\left(x_3\right)=\left(x_1^2-1\right)\left(x_2^2-1\right)\left(x_3^2-1\right)\)
\(=\left(x_1x_2x_3\right)^2-\left(x_1^2x_2^2+x_2^2x_3^2+x_3^2x_1^2\right)+\left(x_1^2+x_2^2+x_3^2\right)-1\)
\(=\left(x_1x_2x_3\right)^2-\left[\left(x_1x_2+x_2x_3+x_3x_1\right)^2-2x_1x_2x_3\left(x_1+x_2+x_3\right)\right]+\left[\left(x_1+x_2+x_3\right)^2-2\left(x_1x_2+x_2x_3+x_3x_1\right)\right]-1\)
\(=\left(-1\right)^2-3^2+2.3-1=-3\)

\(\frac{x+4}{2000}+\frac{x+3}{2001}=\frac{x+2}{2002}+\frac{x+1}{2003}\)
\(\Leftrightarrow2+\frac{x+4}{2000}+\frac{x+3}{2001}=2+\frac{x+2}{2002}+\frac{x+1}{2003}\)
\(\Leftrightarrow\left(\frac{x+4}{2000}+1\right)+\left(\frac{x+3}{2001}+1\right)=\left(\frac{x+2}{2002}+1\right)+\left(\frac{x+1}{2001}+1\right)\)
\(\Leftrightarrow\frac{x+2004}{2000}+\frac{x+2004}{2001}=\frac{x+2004}{2002}+\frac{x+2004}{2003}\)
\(\Leftrightarrow\left(x+2004\right)\left(\frac{1}{2000}+\frac{1}{2001}-\frac{1}{2002}-\frac{1}{2003}\right)=0\)
Mà \(\frac{1}{2000}+\frac{1}{2001}-\frac{1}{2002}-\frac{1}{2003}\ne0\)
Suy ra x+2004=0
\(\Leftrightarrow x=-2004\)

T a c ó 4 3 x - 5 2 - 9 9 x 2 - 25 2 = 0 4 3 x - 5 2 - 9 3 x 2 - 5 2 2 = 0 4 3 x - 5 2 - 9 3 x - 5 3 x + 5 2 = 0 4 3 x - 5 2 - 9 3 x - 5 2 3 x + 5 2 = 0 3 x - 5 2 4 - 3 3 x + 5 2 = 0 3 x - 5 2 4 - 3 3 x + 5 2 = 0 3 x - 5 2 2 2 - 9 x + 15 2 = 0 3 x - 5 2 2 + 9 x + 15 2 – 9 x – 15 = 0 3 x - 5 2 9 x + 17 - 9 x – 13 = 0
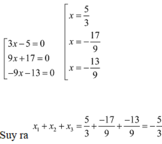
Đáp án cần chọn là: C

Ta có x(3x – 1) – 5(1 – 3x) = 0
ó x(3x – 1) + 5(3x – 1) = 0 ó (3x – 1)(x + 5) = 0
ó x + 5 = 0 3 x - 1 = 0 ó x = - 5 3 x = 1 ó x = - 5 x = 1 3
Suy ra
x 1 = 1 3 ; x 2 = - 5 ⇒ 3 x 1 - x 2 = 3 . 1 3 - - 5 = 6
Đáp án cần chọn là: C
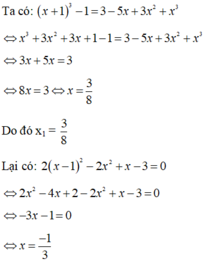
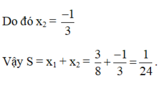
\(3x^2-5x-2=0\)
\(\Leftrightarrow3x^2-6x+x-2=0\)
\(\Leftrightarrow\left(3x-1\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{1}{3}\\x=2\end{cases}}\)
\(A=x_1+x_2=\frac{1}{3}+2=\frac{7}{3}\).