Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Áp dụng định lí sin trong tam giác ABC ta có:
\(\dfrac{a}{{\sin A}} = \dfrac{b}{{\sin B}} = \dfrac{c}{{\sin C}} = 2R\)
\( \Rightarrow R = \dfrac{a}{{2\sin A}};\;\;b = \dfrac{{a.\sin B}}{{\sin A}}\)
Mà \(a = 10,\widehat A = {45^o},\widehat B = {70^o}\)
\( \Rightarrow R = \dfrac{{10}}{{2\sin {{45}^o}}} = 5\sqrt 2 ;\;\;b = \dfrac{{a.\sin {{70}^o}}}{{\sin {{45}^o}}} \approx 13,29\)
Mặt khác: \(\widehat A = {45^o},\widehat B = {70^o} \Rightarrow \widehat C = {65^o}\)
Từ định lí sin ta suy ra: \(c = \dfrac{{a.\sin C}}{{\sin A}} = \dfrac{{10.\sin {{65}^o}}}{{\sin {{45}^o}}} \approx 12,82.\)
Vậy \(R = 5\sqrt 2 ;\;\;b \approx 13,29\); \(c \approx 12,82.\)

Áp dụng định lí sin trong tam giác ABC, ta có:
\(\frac{{AC}}{{\sin B}} = \frac{{AB}}{{\sin C}}\)
\( \Rightarrow AC = \sin B.\frac{{AB}}{{\sin C}} = \sin {60^o}.\frac{{12}}{{\sin {{45}^o}}} = 6\sqrt 6 \)
Lại có: \(\widehat A = {180^o} - ({60^o} + {45^o}) = {75^o}\)
\( \Rightarrow \)Diện tích tam giác ABC là:
\(S = \frac{1}{2}AB.AC.\sin A = \frac{1}{2}.12.6\sqrt 6 .\sin {75^o} \approx 85,2\)
Vậy diện tích tam giác ABC là 85,2.

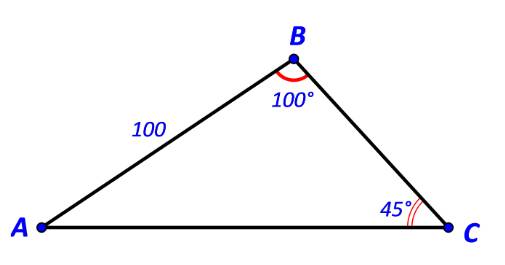
a)
Ta có: \(\widehat A = {180^o} - (\widehat B + \widehat C)\) \( \Rightarrow \widehat A = {180^o} - ({100^o} + {45^o}) = {35^o}\)
Áp dụng định lí sin trong tam giác ABC ta có:
\(\frac{{AB}}{{\sin C}} = \frac{{AC}}{{\sin B}} = \frac{{BC}}{{\sin A}}\)
\( \Rightarrow \left\{ \begin{array}{l}AC = \sin B.\frac{{AB}}{{\sin C}}\\BC = \sin A.\frac{{AB}}{{\sin C}}\end{array} \right.\)\( \Leftrightarrow \left\{ \begin{array}{l}AC = \sin {100^o}.\frac{{100}}{{\sin {{45}^o}}} \approx 139,3\\BC = \sin {35^o}.\frac{{100}}{{\sin {{45}^o}}} \approx 81,1\end{array} \right.\)
b)
Diện tích tam giác ABC là: \(S = \frac{1}{2}.BC.AC.\sin C = \frac{1}{2}.81,1.139,3.\sin {45^o} \approx 3994,2.\)

Ta có: \(\widehat B = {65^o},\widehat C = {85^o}.\)
\( \Rightarrow \widehat A = {180^o} - \left( {{{65}^o} + {{85}^o}} \right) = {30^o}.\)
Áp dụng định lí sin trong tam giác ABC, ta có:
\(\frac{{BC}}{{\sin A}} = 2R \Rightarrow BC = 2R.\sin A\)
Mà \(\widehat A = {30^o},R = 6.\)
\( \Rightarrow BC = 2.6.\sin {30^o} = 6.\)
Vậy BC = 6.

S2= p(p-AB)(p-AC)(p-BC) *
mà p=(a+b+c):2
=> p= (7+9+12):2
=> p= 14 (đvđđd)
*<=> S2=14(14-7)(14-9)(14-12)
<=>S=\(\sqrt{\left(980\right)}\)
<=> S=\(14\sqrt{5}\)
S= (abc):4R => S=(7x9x12):4R => S=756:4R
=> R=6
S=pr
=> S=14r
=> r= \(\sqrt{\left(5\right)}\)

Ta có: \(\widehat B = {75^o},\widehat C = {45^o}\)\( \Rightarrow \widehat A = {180^o} - \left( {{{75}^o} + {{45}^o}} \right) = {60^o}\)
Áp dụng định lí sin trong tam giác ABC ta có:
\(\frac{{AB}}{{\sin C}} = \frac{{BC}}{{\sin A}}\)
\( \Rightarrow AB = \sin C.\frac{{BC}}{{\sin A}} = \sin {45^o}.\frac{{50}}{{\sin {{60}^o}}} \approx 40,8\)
Vậy độ dài cạnh AB là 40,8.

Áp dụng định lí sin cho tam giác ABC ta có:
\(\frac{b}{{\sin B}} = \frac{c}{{\sin C}}\)
\( \Rightarrow c = \sin C.\frac{b}{{\sin B}} = \sin {45^o}.\frac{2}{{\sin {{30}^o}}} = 2\sqrt 2 \)
Lại có: \(\;\widehat A = {180^o} - \widehat B - \widehat C = {180^o} - {30^o} - {45^o} = {105^o}\)
Do đó diện tích tích S của tam giác ABC là:
\(S = \frac{1}{2}bc.\sin A = \frac{1}{2}.2.2\sqrt 2 .\sin {105^o} = 1 + \sqrt 3 .\)
Vậy diện tích tam giác ABC là \(1 + \sqrt 3 \).

Ta có \(\widehat{A}+\widehat{B}+\widehat{C}=180^o\Rightarrow\widehat{A}=75^o\)
* \(\dfrac{BC}{sinA}=\dfrac{AB}{sinC}\Rightarrow AB=\dfrac{BCsinC}{sinA}=a\left(1+\sqrt{3}\right)\)
* \(\dfrac{BC}{sinA}=\dfrac{AC}{sinB}\Rightarrow AC=\dfrac{BCsinB}{sinA}=a\left(\dfrac{-6+3\sqrt{2}}{2}\right)\)
Theo định lí sin: \(\frac{a}{{\sin A}} = \frac{b}{{\sin B}} = \frac{c}{{\sin C}} = 2R\quad (*)\)
+) Ta có: \(\hat A = {180^o} - \left( {\hat B + \;\hat C} \right) = {180^o} - \left( {{{60}^o} + {{45}^o}} \right) = {75^o}\)
\( \Rightarrow a = \frac{b}{{\sin B}}.\sin A = \frac{{10}}{{\sin {{60}^o}}}.\sin {75^o} \approx 11,154\)
+) \((*) \Rightarrow R = \frac{b}{{2\sin B}} = \frac{{10}}{{2\sin {{60}^o}}} = \frac{{10}}{{2.\frac{{\sqrt 3 }}{2}}} = \frac{{10\sqrt 3 }}{3}.\)
+) Diện tích tam giác ABC là: \(S = \frac{1}{2}ab.\sin {\mkern 1mu} \hat C\) \( \approx \frac{1}{2}.11,154.10.\sin {45^o}\)\( \approx 39,44\)
+) Lại có: \(R = \frac{c}{{2\sin C}}\)\( \Rightarrow c = 2.\frac{{10\sqrt 3 }}{3}.\sin {45^o} = \frac{{10\sqrt 6 }}{3} \approx 8,165\)
\( \Rightarrow p = \frac{{a + b + c}}{2} \approx \frac{{11,154 + 10 + 8,165}}{2} \approx 14,66\)
\( \Rightarrow r = \frac{S}{p} \approx \frac{{39,44}}{{14,66}} \approx 2,7\)