NP
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LT
0
SM
0
NV
Nguyễn Việt Lâm
Giáo viên
29 tháng 5 2020
\(cos2A+cos2B+cos2C=2cos\left(A+B\right).cos\left(A-B\right)+2cos^2C-1\)
\(=-2cosC.cos\left(A-B\right)+2cos^2C-1\)
\(=-2cosC\left[cos\left(A-B\right)-cosC\right]-1\)
\(=-2cosC\left[cos\left(A-B\right)+cos\left(A+B\right)\right]-1\)
\(=-4cosC.cosA.cosB-1\)
\(sin2A+sin2B+sin2C=2sin\left(A+B\right)cos\left(A-B\right)+2sinC.cosC\)
\(=2sinC.cos\left(A-B\right)+2sinC.cosC\)
\(=2sinC\left[cos\left(A-B\right)+cosC\right]=2sinC\left[cos\left(A-B\right)-cos\left(A+B\right)\right]\)
\(=-4sinC.sinA.sin\left(-B\right)=4sinA.sinB.sinC\)
JK
1
TT
17 tháng 4 2022
cos2a+cos2b+cos2c=1
\(\Leftrightarrow\)(cos2a+cos2b)+(cos2c-1)=0
\(\Leftrightarrow\)2cos(a+b)cos(a-b)+2cos2c=0
\(\Leftrightarrow\)2cos(180-c)cos(a-b)+2cos2c=0
\(\Leftrightarrow\)-2cosccos(a-b)+2cos2c=0
\(\Leftrightarrow\)-2cosc[cos(a-b)-cosc]=0
\(\Leftrightarrow\)-2cosc[cos(a-b)+cos(180-c)]=0
\(\Leftrightarrow\)-2cosc[cos(a-b)+cos(a+b)]=0
\(\Leftrightarrow\)-2cosc(2cosacosb)=0
\(\Rightarrow\) một trong ba giá trị cosc cosb cosa bằng 0\(\Rightarrow\) abc là tam giác vuông
đây là nếu đề của bạn là ...=1 còn nếu là ...=-1 thì mình không biết cách giải!
HY
7 tháng 4 2017
Có: cos 2A + 2√2.cos B + 2√2.cos C = 3
⇔2cos²A - 1 + 2√2.2.cos[(B + C)/2] . cos[(B - C)/2] - 3 = 0
⇔2cos²A + 4√2.sin (A/2) . cos[(B - C)/2] - 4 = 0(1)
Ta thấy: sin(A/2) > 0 ; cos[(B - C)/2] ≤ 1
⇒VT ≤ 2cos²A + 4√2.sin(A/2) - 4
Vì tam giác ABC không tù nên 0 ≤ cos A < 1
⇒cos²A ≤ cos A
⇒VT ≤ 2cos A + 4√2.sin(A/2) - 4
⇒VT ≤ 2.[1 - 2.(sin A/2)²] + 4√2.sin(A/2) - 4
⇒VT ≤ -4.(sin A/2)² + 4√2.sin(A/2) - 2
⇒VT ≤ -2(√2.sin A/2 - 1)² ≤ 0(2)
Kết hợp (1)(2) thì đẳng thức xảy ra khi tất cả các dấu = ở trên xảy ra
⇔cos [(B - C)/2] = 1 và cos²A = cos A và √2.sin A/2 - 1 = 0
⇔góc B = góc C và cos A = 0 và sin A/2 = 1/√2
⇔ góc B = góc C và góc A = 90 độ
Vậy góc A = 90 độ, góc B = góc C = 45 độ
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 6 2019
\(P=sin^22a+cos^22a+sin^22b+cos^22b+2sin2a.sin2b+2cos2a.cos2b\)
\(P=2+2\left(sin2a.sin2b+cos2a.cos2b\right)=2+2cos\left(2a-2b\right)\)
\(P=2+2cos\frac{\pi}{3}=3\)
NL
1
28 tháng 6 2022
\(VT=\dfrac{1+\cos^2a-\sin^2a+2\cdot\sin a\cdot\cos a}{1+2\cdot\sin a\cdot\cos a-\cos^2a+\sin^2a}\)
\(=\dfrac{2\cdot\cos^2a+2\cdot\sin a\cdot\cos a}{2\cdot\sin^2a+2\cdot\sin a\cdot\cos a}\)
\(=\dfrac{2\cdot\cos a\left(\cos a+\sin a\right)}{2\cdot\sin a\cdot\left(\sin a+\cos a\right)}\)
\(=\dfrac{\cos a}{\sin a}=\cot a\)
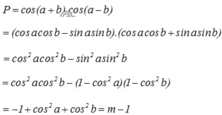
Lời giải:
Sử dụng các công thức lượng giác ta thực hiện biến đổi biểu thức như sau:
\(\cos 2A+\cos 2B+\cos =2\cos \frac{2A+2B}{2}\cos \frac{2A-2B}{2}+\cos ^2C-\sin ^2C\)
\(=2\cos (A+B)\cos (A-B)+2\cos ^2C-(\sin ^2C+\cos ^2C)\)
\(=2\cos (\pi -C)\cos (A-B)+2\cos ^2C-1\)
\(=2\cos ^2C-2\cos C\cos ^2(A-B)-1\)
\(=2[\cos ^2C-\cos C\cos (A-B)+\frac{1}{4}\cos ^2(A-B)]-\frac{1}{2}\cos ^2(A-B)-1\)
\(=2[\cos C-\frac{1}{2}\cos (A-B)]^2-\frac{1}{2}\cos ^2(A-B)-1\)
Ta thấy :
\(2[\cos C-\frac{1}{2}\cos (A-B)]^2\geq 0\)
\(\cos ^2(A-B)\leq 1\) (tính chất hàm cos)
\(\Rightarrow \cos 2A+\cos 2B+\cos 2C\geq 2.0-\frac{1}{2}.1-1=\frac{-3}{2}\)
Ta có đpcm.