Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. thay m=-4 vào (1) ta có:
\(x^2-5x-6=0\)
Δ=b\(^2\)-4ac= (-5)\(^2\) - 4.1.(-6)= 25 + 24= 49 > 0
\(\sqrt{\Delta}=\sqrt{49}=7\)
x\(_1\)=\(\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{5+7}{2}\)=6
x\(_2\)=\(\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{5-7}{2}\)=-1
vậy khi x=-4 thì pt đã cho có 2 nghiệm x\(_1\)=6; x\(_2\)=-1

\(\Delta'=\left(m-1\right)^2-m^2+m-1=m^2-2m+1-m^2+m-1=-m.\)
Để phương trình có 2 nghiệm thì \(\Delta'\ge0\Leftrightarrow-m\ge0\Leftrightarrow m\le0\)
Theo vi ét:
\(\hept{\begin{cases}x_1+x_2=-2\left(m-1\right)\\x_1.x_2=m^2-m+1=\left(m-\frac{1}{2}\right)^2+\frac{3}{4}>0\end{cases}}\)
\(\left|x_1\right|+\left|x_2\right|=4\Leftrightarrow x_1+x_2+2\left|x_1.x_2\right|=16\)
\(\Leftrightarrow1-2m+2\left|m^2-m+1\right|=16\)
\(\Leftrightarrow1-2m+2m^2-2m+2=16\)(Vì \(m^2-m+1>0\Rightarrow\left|m^2-m+1\right|=m^2-m+1\))
\(\Leftrightarrow2m^2-4m-13=0\)
Đến đây bạn tự giải \(\Delta\)tìm m đối chiếu điều kiện là ok.

a, bạn tự làm
b, Thay x = 3 vào pt trên ta được
\(9-3m-3=0\Leftrightarrow6-3m=0\Leftrightarrow m=2\)
Thay m = 2 vào ta được \(x^2-2x-3=0\)
Ta có a - b + c = 1 + 2 - 3 = 0
vậy pt có 2 nghiệm x = -1 ; x = 3
c, \(\Delta=m^2-4\left(-3\right)=m^2+12>0\)
vậy pt luôn có 2 nghiệm pb
\(x_1x_2+5\left(x_1+x_2\right)-1997=0\)
\(\Rightarrow-3+5m-1997=0\Leftrightarrow5m-2000=0\Leftrightarrow m=400\)

a: Thay m=3 vào pt, ta được:
\(x^2-4x-1=0\)
\(\Leftrightarrow\left(x-2\right)^2=5\)
hay \(\left[{}\begin{matrix}x=\sqrt{5}+2\\x=-\sqrt{5}+2\end{matrix}\right.\)
b: \(\text{Δ}=\left(-4\right)^2-4\left(-2m+5\right)\)
\(=16+8m-20=8m-4\)
Để phương trình có hai nghiệm thì 8m-4>=0
hay m>=1/2
Theo đề, ta có: \(\left(x_1+x_2\right)^2+3x_1x_2-3\left(x_1+x_2\right)=0\)
\(\Leftrightarrow4^2-3\cdot4+3\left(-2m+5\right)=0\)
\(\Leftrightarrow4-6m+15=0\)
=>-6m+19=0
hay m=19/6(nhận)

\(\Delta=\left[-2\left(m+1\right)\right]^2-4\left(m^2-3\right)\)
\(=4m^2+8m+4-4m^2+12=8m+16\)
Để phương trình có hai nghiệm thì 8m+16>=0
hay m>=-2
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=m^2-3\end{matrix}\right.\)
Theo đề, ta có: \(x_1^2+x_2^2+1=3x_1x_2\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-5x_1x_2+1=0\)
\(\Leftrightarrow\left(2m+2\right)^2-5\left(m^2-3\right)+1=0\)
\(\Leftrightarrow4m^2+8m+4-5m^2+15+1=0\)
\(\Leftrightarrow-m^2+8m+20=0\)
=>(m-10)(m+2)=0
=>m=10 hoặc m=-2
a, \(\Delta'=\left(m+1\right)^2-\left(m^2-3\right)=m^2+2m+1-m^2+3=2m+4\)
Để pt có 2 nghiệm x1 ; x2 khi \(\Delta'\ge0\Leftrightarrow m\ge-2\)
Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=m^2-3\end{matrix}\right.\)
Ta có : \(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}+\dfrac{1}{x_1x_2}=3\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-2x_1x_2+1}{x_1x_2}=3\)
\(\Leftrightarrow\dfrac{4\left(m^2+2m+1\right)-2\left(m^2-3\right)+1}{m^2-3}=3\)
\(\Rightarrow2m^2+8m+11=3m^2-9\Leftrightarrow m^2-8m-20=0\Leftrightarrow m=10;m=-2\)(tm)

a) \(x_1^2+x_2^2=23\)
\(\Leftrightarrow x_1^2+2x_1x_2+x_2^2-2x_1x_2=23\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=23\)
\(\Leftrightarrow5^2-2\left(m+4\right)=23\)
<=> m=-3
b) \(x_1^3+x_2^3=35\)
\(\Leftrightarrow\left(x_1+x_2\right)\left(x_1^2+x_1x_2+x_2^2\right)=35\)
\(\Leftrightarrow\left(x_1+x_2\right)\left[\left(x_1+x_2\right)^2-3x_1x_2\right]=35\)
\(\Leftrightarrow5\left[5^2-3\left(m+4\right)\right]=35\)
<=> m=2
c) \(\left|x_2-x_1\right|=3\)
\(\Leftrightarrow\left(\left|x_2-x_1\right|\right)^2=3^2\)
\(\Leftrightarrow x_1^2-2x_1x_2+x_1^2=3^2\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=9\)
<=> m=0
ĐK để pt có hai nghiệm phân biệt là: \(\Delta>0\Leftrightarrow25-4\left(m+4\right)>0\Leftrightarrow m< \frac{9}{4}\) ( @@)
Gọi \(x_1;x_2\) là hai nghiệm của phương trình
Theo định lí Viet ta có: \(x_1+x_2=5;x_1.x_2=m+4\)
a) \(x_1^2+x_2^2=23\)
<=> \(x_1^2+x_2^2+2x_1x_2=23+2x_1x_2\)
<=> \(\left(x_1+x_2\right)^2=23+2x_1x_2\)
=> \(25=23+2\left(m+4\right)\)
<=>m = -3 ( thỏa mãn @@)
b) \(x_1^3+x_2^3=35\)
<=> \(\left(x_1+x_2\right)^3-3\left(x_1+x_2\right)x_1x_2=35\)
=> \(5^3-3.5.\left(m+4\right)=35\)
<=> m = 2 ( thỏa mãn @@)
c) \(\left|x_2-x_1\right|=3\)
<=> \(\left(x_1-x_2\right)^2=9\)
<=> \(\left(x_1+x_2\right)^2-4x_1x_2=9\)
=> \(5^2-4\left(m+4\right)=9\)
<=> m = 0 ( thỏa mãn @@)

b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)

Đề bài sai bạn
Biểu thức \(\left|\dfrac{x_1+x_2+4}{x_1+x_2}\right|=\left|1+\dfrac{1}{m}\right|\) này ko tồn tại max, chỉ tồn tại min
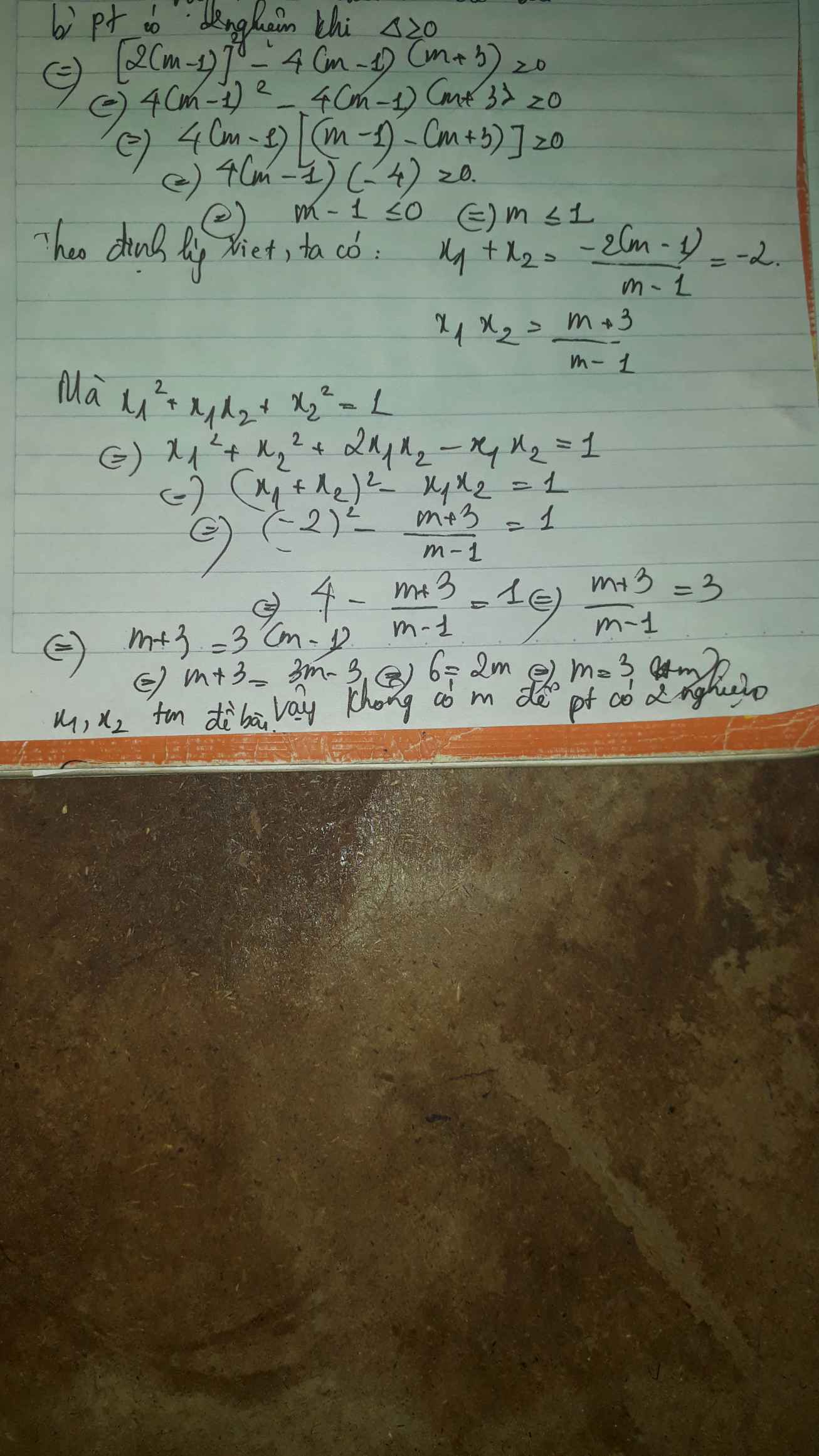
a, bạn tự làm
b, \(\Delta'=\left(m+2\right)^2-\left(m^2+m+3\right)=m^2+4m+4-m^2-m-3\)
\(=3m+1\)để pt có 2 nghiệm \(m\ge-\dfrac{1}{3}\)
Ta có \(\dfrac{x_1^2+x_2^2}{x_1x_2}=4\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-2x_1x_2}{x_1x_2}=4\Rightarrow\left(x_1+x_2\right)^2-6x_1x_2=0\)
\(\Rightarrow4\left(m+2\right)^2-6\left(m^2+m+3\right)=0\)
\(\Leftrightarrow4m^2+16m+16-6m^2-6m-18=0\)
\(\Leftrightarrow-2m^2+10m-2=0\Leftrightarrow m^2-5m+1=0\Leftrightarrow m=\dfrac{5\pm\sqrt{21}}{2}\)(tm)