Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có M là trung điểm của AC nên
K là trung điểm của BC nên
Bạn tự vẽ hình minh họa nha :>
Gọi G là giao điểm của AK, BM thì G là trọng tâm của tam giác.
Ta có 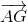 =
= 
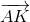 =>
=> 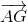 =
=

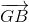 = -
= -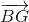 = -
= -
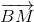 = -
= -

Theo quy tắc 3 điểm đối với tổng vec-tơ:
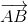 =
= 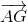 +
+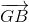 =>
=> 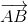 =
= 
 -
- 
 =
=  (
( -
-  ).
).
AK là trung tuyến thuộc cạnh BC nên
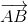 +
+ 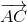 = 2
= 2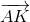 =>
=> 
 -
- 
 +
+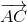 = 2
= 2
Từ đây ta có 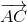 =
= 
 +
+
 =>
=> 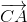 = -
= -
 -
- 
 .
.
BM là trung tuyến thuộc đỉnh B nên:
 +
+ 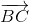 = 2
= 2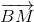 => -
=> - 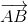 +
+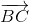 = 2
= 2
=> 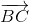 =
= 
 +
+
 .
.

a: \(\overrightarrow{AI}=\dfrac{1}{2}\left(\overrightarrow{AM}+\overrightarrow{AN}\right)=\dfrac{1}{4}\overrightarrow{AB}+\dfrac{1}{4}\overrightarrow{AC}\)

Câu 1:
A B C K M G
Gọi G là giao điểm AK và BM => G là trọng tâm \(\Delta ABC\)
\(\Rightarrow\) Theo tính chất trọng tâm \(\left\{{}\begin{matrix}AG=\frac{2}{3}AK\\BG=\frac{2}{3}BM\end{matrix}\right.\)
\(\Rightarrow\overrightarrow{AB}=\overrightarrow{AG}+\overrightarrow{GB}=\frac{2}{3}\overrightarrow{AK}-\frac{2}{3}\overrightarrow{BM}\\ \Rightarrow\overrightarrow{AC}=\overrightarrow{AK}+\overrightarrow{KC}=\overrightarrow{AK}+\frac{1}{2}\overrightarrow{BC}\\ =\overrightarrow{AK}+\frac{1}{2}\left(\overrightarrow{BA}+\overrightarrow{AC}\right)=\overrightarrow{AK}-\frac{1}{2}\overrightarrow{AB}+\frac{1}{2}\overrightarrow{AC}\\ \Rightarrow\frac{1}{2}\overrightarrow{AC}=\overrightarrow{AK}-\frac{1}{2}\left(\frac{2}{3}\overrightarrow{AK}-\frac{2}{3}\overrightarrow{BM}\right)\\ =\overrightarrow{AK}-\frac{1}{3}\overrightarrow{AK}+\frac{1}{3}\overrightarrow{BM}\\ =\frac{2}{3}\overrightarrow{AK}+\frac{1}{3}\overrightarrow{BM}\\ \Rightarrow\overrightarrow{AC}=\frac{4}{3}\overrightarrow{AK}+\frac{2}{3}\overrightarrow{BM}\\ \Rightarrow\overrightarrow{BC}=\overrightarrow{BA}+\overrightarrow{AC}=\overrightarrow{-AB}+\overrightarrow{AC}\\ =-\left(\frac{2}{3}\overrightarrow{AK}-\frac{2}{3}\overrightarrow{BM}\right)+\left(\frac{4}{3}\overrightarrow{AK}+\frac{2}{3}\overrightarrow{BM}\right)\\ =-\frac{2}{3}\overrightarrow{AK}+\frac{2}{3}\overrightarrow{BM}+\frac{4}{3}\overrightarrow{AK}+\frac{2}{3}\overrightarrow{BM}\\ =\frac{2}{3}\overrightarrow{AK}+\frac{4}{3}\overrightarrow{BM}\)
1/ Theo quy tắc TĐ: \(\overrightarrow{AK}=\frac{\overrightarrow{AB}+\overrightarrow{AC}}{2};\overrightarrow{BM}=\frac{\overrightarrow{BA}+\overrightarrow{BC}}{2}\)
Theo quy tắc 3 điểm: \(\overrightarrow{AB}=\overrightarrow{AK}+\overrightarrow{KB}\)
Vậy cần phân tích \(\overrightarrow{KB}\)
\(\overrightarrow{KB}=\frac{\overrightarrow{CB}}{2}=\frac{\overrightarrow{BA}-2\overrightarrow{BM}}{2}\)
\(\Rightarrow\overrightarrow{AB}=\overrightarrow{AK}+\frac{\overrightarrow{BA}-2\overrightarrow{BM}}{2}\Leftrightarrow2\overrightarrow{AB}=2\overrightarrow{AK}-\overrightarrow{AB}-2\overrightarrow{BM}\)
\(\Leftrightarrow\overrightarrow{AB}=\frac{2}{3}\overrightarrow{AK}-\frac{2}{3}\overrightarrow{BM}\)
Tìm \(\overrightarrow{BC};\overrightarrow{AC}\) tương tự
2/ Theo quy tắc 3 điểm có:
\(\overrightarrow{AN}=\overrightarrow{AB}+\overrightarrow{BN}\)
\(\overrightarrow{BP}=\overrightarrow{BC}+\overrightarrow{CP}\)
\(\overrightarrow{CM}=\overrightarrow{CA}+\overrightarrow{AM}\)
Cộng vế vs vế:
\(\overrightarrow{AN}+\overrightarrow{BP}+\overrightarrow{CM}=\overrightarrow{AB}+\overrightarrow{BC}-\overrightarrow{AC}+\frac{1}{3}\left(\overrightarrow{BC}+\overrightarrow{CA}-\overrightarrow{BA}\right)=0\)

\(\overrightarrow{BM}=\dfrac{\overrightarrow{BA}+\overrightarrow{BC}}{2}=\dfrac{\overrightarrow{BA}+\overrightarrow{BA}+\overrightarrow{AC}}{2}=-\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}\)
\(\overrightarrow{AN}=\overrightarrow{AB}+\overrightarrow{BN}=\overrightarrow{AB}+\dfrac{2}{5}\overrightarrow{BA}+\dfrac{2}{5}\overrightarrow{AC}=\dfrac{3}{5}\overrightarrow{AB}+\dfrac{2}{5}\overrightarrow{AC}\)

Lời giải:
Ta có:
\(\overrightarrow{AB}=\overrightarrow{AM}+\overrightarrow{MB}=\overrightarrow{AM}+\overrightarrow{MN}+\overrightarrow{NB}=\overrightarrow{AM}-\overrightarrow{BN}+\overrightarrow{MN}\)
Vì $AM,BN$ là trung tuyến nên $M,N$ lần lượt là trung điểm của $BC, AC$
$\Rightarrow MN$ là đường trung bình của tam giác $ABC$ ứng với $AB$
\(\Rightarrow \overrightarrow{MN}=\frac{1}{2}\overrightarrow{BA}=-\frac{1}{2}\overrightarrow{AB}\). Do đó:
\(\overrightarrow{AB}=\overrightarrow{AM}-\overrightarrow{BN}-\frac{1}{2}\overrightarrow{AB}\Leftrightarrow \frac{3}{2}\overrightarrow{AB}=\overrightarrow{AM}-\overrightarrow{BN}\)
\(\Leftrightarrow \overrightarrow{AB}=\frac{2}{3}\overrightarrow{AM}-\frac{2}{3}\overrightarrow{BN}\)


Gọi G là giao điểm của AK, BM thì G là trọng tâm của tam giác.
Ta có 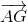 =
= 
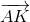 =>
=> 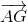 =
=

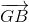 = -
= -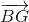 = -
= -
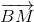 = -
= -

Theo quy tắc 3 điểm đối với tổng vec tơ:
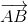 =
= 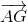 +
+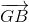 =>
=> 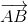 =
= 
 -
- 
 =
=  (
( -
-  ).
).
AK là trung tuyến thuộc cạnh BC nên
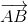 +
+ 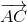 = 2
= 2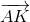 =>
=> 
 -
- 
 +
+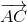 = 2
= 2
Từ đây ta có 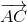 =
= 
 +
+
 =>
=> 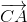 = -
= -
 -
- 
 .
.
BM là trung tuyến thuộc đỉnh B nên
 +
+ 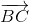 = 2
= 2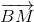 => -
=> - 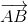 +
+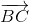 = 2
= 2
=> 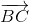 =
= 
 +
+
 .
.

\(\overrightarrow{BM}=-\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}\)
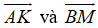
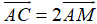
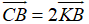
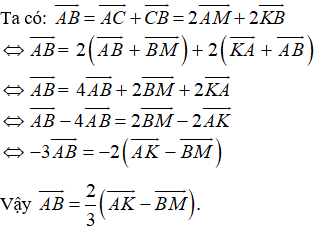

Tự vẽ hình nha bạn :)
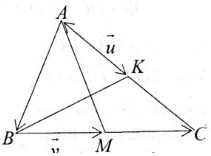
Gọi G là trọng tâm của tam giác ABC . Ta có :
\(\left\{{}\begin{matrix}\overrightarrow{AG}=\frac{2}{3}\overrightarrow{u}\\\overrightarrow{GM}=\frac{1}{3}\overrightarrow{v}\end{matrix}\right.\Rightarrow\overrightarrow{AM}=\overrightarrow{AG}+\overrightarrow{GM}=\frac{2}{3}\overrightarrow{u}+\frac{1}{3}\overrightarrow{v}\)
\(\Rightarrow\overrightarrow{AC}=2\overrightarrow{AM}=\frac{4}{3}\overrightarrow{u}+\frac{2}{3}\overrightarrow{v}\)
\(\left\{{}\begin{matrix}\overrightarrow{GK}=\frac{1}{3}\overrightarrow{u}\\\overrightarrow{BG}=\frac{2}{3}\overrightarrow{v}\end{matrix}\right.\Rightarrow\overrightarrow{BK}=\overrightarrow{BG}+\overrightarrow{GK}=\frac{1}{3}\overrightarrow{u}+\frac{2}{3}\overrightarrow{v}\)
\(\Rightarrow\overrightarrow{BC}=2\overrightarrow{BK}=\frac{2}{3}\overrightarrow{u}+\frac{4}{3}\overrightarrow{v}\)
\(\Rightarrow\overrightarrow{AB}=\overrightarrow{CB}-\overrightarrow{CA}=-\frac{2}{3}\overrightarrow{u}-\frac{4}{3}\overrightarrow{v}-\frac{4}{3}\overrightarrow{u}-\frac{2}{3}\overrightarrow{v}=-2\overrightarrow{u}-2\overrightarrow{v}\)