Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Gọi biểu thức là \(A\). Ta có :
\(A=\dfrac{3}{1.2.3}+\dfrac{5}{2.3.4}+\dfrac{7}{3.4.5}+...+\dfrac{2017}{1008.1009.1010}\)
\(A=\left(\dfrac{1.2}{1.2.3}+\dfrac{2.2}{2.3.4}+\dfrac{3.2}{3.4.5}+...+\dfrac{1008.2}{1008.1009.1010}\right)+\left(\dfrac{1}{1.2.3}+\dfrac{1}{2.3.4}+\dfrac{1}{3.4.5}+...+\dfrac{1}{1008.1009.1010}\right)\)\(A=\left(\dfrac{2}{2.3}+\dfrac{2}{3.4}+\dfrac{2}{4.5}+...+\dfrac{2}{1009.1010}\right)+\dfrac{1}{2}\left(\dfrac{1}{1.2}-\dfrac{1}{2.3}+\dfrac{1}{2.3}-\dfrac{1}{3.4}+\dfrac{1}{3.4}-\dfrac{1}{4.5}+...+\dfrac{1}{1008.1009}-\dfrac{1}{1009.1010}\right)\)
\(A=2\left(\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{5}+...+\dfrac{1}{1009}-\dfrac{1}{1010}\right)+\dfrac{1}{2}\left(\dfrac{1}{2}-\dfrac{1}{1009.1010}\right)\)
\(A< 2.\dfrac{1}{2}+\dfrac{1}{2}.\dfrac{1}{2}=1+\dfrac{1}{4}=\dfrac{5}{4}\)
a: (x+1/2)(2/3-2x)=0
=>x+1/2=0 hoặc 2/3-2x=0
=>x=-1/2 hoặc x=1/3
b: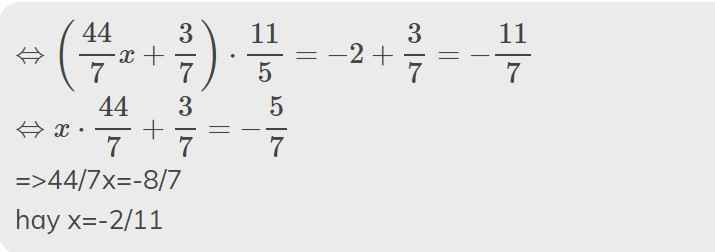 
c: \(\Leftrightarrow x\cdot\left(\dfrac{13}{4}-\dfrac{7}{6}\right)=\dfrac{5}{12}+\dfrac{5}{3}=\dfrac{5}{12}+\dfrac{20}{12}=\dfrac{25}{12}\)
\(\Leftrightarrow x=\dfrac{25}{12}:\dfrac{39-14}{12}=\dfrac{25}{25}=1\)
\(A=\dfrac{3}{4}\cdot\dfrac{8}{9}\cdot\dfrac{15}{16}\cdot...\cdot\dfrac{899}{900}\)
\(A=\dfrac{1\cdot3}{2\cdot2}\cdot\dfrac{2\cdot4}{3\cdot3}\cdot\dfrac{3\cdot5}{4\cdot4}\cdot...\cdot\dfrac{29\cdot31}{30\cdot30}\)
\(A=\dfrac{1\cdot\left(2\cdot3\cdot4\cdot5\cdot...\cdot29\right)^2\cdot30\cdot31}{\left(2\cdot3\cdot4\cdot...\cdot30\right)^2}\)
\(A=\dfrac{1\cdot\left(2\cdot3\cdot4\cdot5\cdot...\cdot29\right)^2\cdot30\cdot31}{\left(2\cdot3\cdot4\cdot5\cdot...\cdot29\right)^2\cdot30\cdot30}\)
\(A=\dfrac{1\cdot31}{30}=\dfrac{31}{30}\)
Ta có : \(\dfrac{1}{101}>\dfrac{1}{300}\)
...
\(\dfrac{1}{299}>\dfrac{1}{300}\)
Do đó :
\(\dfrac{1}{101}+\dfrac{1}{102}+..+\dfrac{1}{300}>\dfrac{1}{300}+\dfrac{1}{300}..+\dfrac{1}{300}\)
\(\Rightarrow\dfrac{1}{101}+\dfrac{1}{102}+..+\dfrac{1}{300}>\dfrac{200}{300}=\dfrac{2}{3}\)
Vậy...
a) (1/7.x-2/7).(-1/5.x-2/5)=0
=> 1/7.x-2/7=0hoặc-1/5.x-2/5=0
*1/7.x-2/7=0
1/7.x=0+2/7
1/7.x=2/7
x=2/7:1/7
x=2
b)1/6.x+1/10.x-4/5.x+1=0
(1/6+1/10-4/5).x+1=0
(1/6+1/10-4/5).x=0-1
(1/6+1/10-4/5).x=-1
(-8/15).x=-1
x=-1:(-8/15) =15/8
1.a) Dễ nhận thấy đề toán chỉ giải được khi đề là tìm x,y. Còn nếu là tìm x ta nhận thấy ngay vô nghiệm. Do đó: Sửa đề: \(\left|x-3\right|+\left|2-y\right|=0\)
\(\Leftrightarrow\left|x-3\right|=\left|2-y\right|=0\)
\(\left|x-3\right|=0\Rightarrow\left\{{}\begin{matrix}x-3=0\\-\left(x-3\right)=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\) (1)
\(\left|2-y\right|=0\Rightarrow\left\{{}\begin{matrix}2-y=0\\-\left(2-y\right)=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=2\\y=-2\end{matrix}\right.\) (2)
Từ (1) và (2) có: \(\left[{}\begin{matrix}\left\{{}\begin{matrix}x_1=3\\x_2=-3\end{matrix}\right.\\\left\{{}\begin{matrix}y_1=2\\y_2=-2\end{matrix}\right.\end{matrix}\right.\)
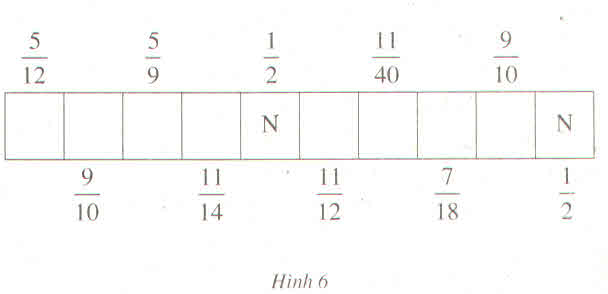
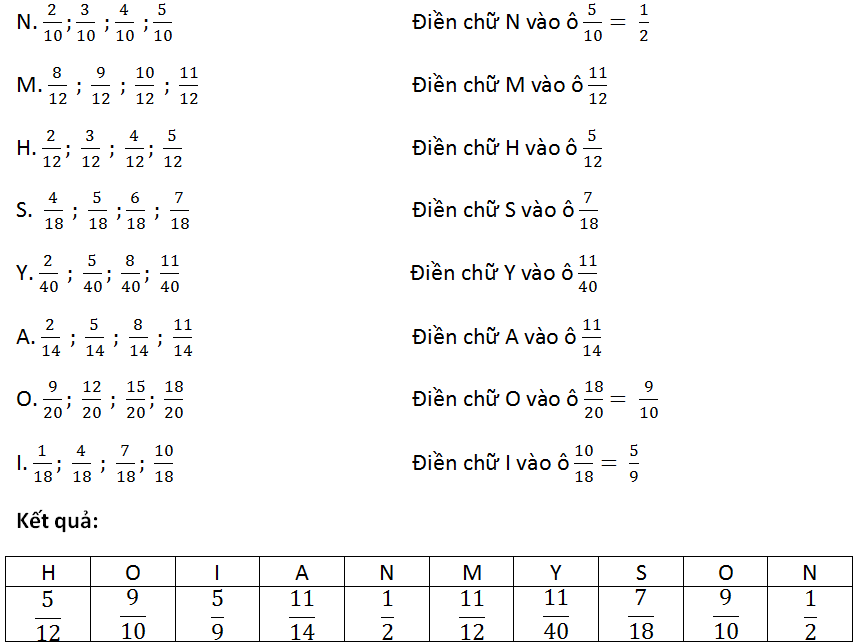Đó là hai địa danh HỘI AN và MỸ SƠN của Viêt Nam được UNESCO công nhân là di sản văn hóa thế giới vào năm 1999.
Câu 1: D
Câu 2:
THam khảo: