
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Có gì khó đâu bạn -..-
( 2x + 5 )( 2x - 7 ) - ( -4x - 3 )2 = 16
<=> 2x( 2x - 7 ) + 5( 2x - 7 ) - [ (-4x)2 - 2.3.(-4x) + 32 ] = 16
<=> 4x2 - 14x + 10x - 35 - [ 16x2 + 24x + 9 ] = 16
<=> 4x2 - 4x - 35 - 16x2 - 24x - 9 = 16
<=> -12x2 - 28x - 44 - 16 = 0
<=> -12x2 - 28x - 60 = 0
<=> -4( 3x2 + 7x + 15 ) = 0
<=> 3x2 + 7x + 15 = 0
Ta có : 3x2 + 7x + 15 = 3( x2 + 7/3x + 49/36 ) + 131/12 = 3( x + 7/6 )2 + 131/12 ≥ 131/12 > 0 ∀ x
=> Vô nghiệm
\(4x^2-14x+10x-35-\left(16x^2+24x+9\right)=16\)
\(4x^2-4x-35-16x^2-24x-9-16=0\)
\(-12x^2-28x-60=0\)
\(-4\left(3x^2+7x+15\right)=0\)
\(3x^2+7x+15=0\)
\(3\left(x^2+\frac{7}{3}x+5\right)=0\)
\(x^2+\frac{7}{3}x+5=0\)
\(x^2+2\cdot x\cdot\frac{7}{6}+\left(\frac{7}{6}\right)^2-\left(\frac{7}{6}\right)^2+5=0\)
\(\left(x+\frac{7}{6}\right)^2+\frac{131}{36}=0\)
\(\left(x+\frac{7}{6}\right)^2=-\frac{131}{36}\) ( vô lí vì \(\left(x+\frac{7}{6}\right)^2\ge0\forall x\) )
Vậy phương trình vô nghiệm

\(\dfrac{2\left(5x+2\right)}{9}-1=\dfrac{4\left(33+2x\right)}{5}-\dfrac{5\left(1-11x\right)}{9}\)
\(\dfrac{10\left(5x+2\right)}{45}-\dfrac{45}{45}=\dfrac{36\left(33+2x\right)}{45}-\dfrac{25\left(1-11x\right)}{45}\)
\(50x-20-45=1188+72x-25+275x\)
\(50x-25=347x+1163\)
\(50x-347x=25+1163\)
\(-297x=1188\)
\(x=4\\ \)
d)
\(\dfrac{2\left(x-4\right)}{3}+\dfrac{3x+13}{8}=\dfrac{2\left(2x-3\right)}{5}+12\)
\(\dfrac{80\left(x-4\right)}{120}+\dfrac{15\left(3x+13\right)}{120}=\dfrac{40\left(2x-3\right)}{120}+\dfrac{1440}{120}\)
\(80x-320+45x+195=80x-120+1440\)
\(125x-125=80x+1320\)
\(125x-80x=125+1320\)
\(45x=1445\)
\(x=\dfrac{1445}{45}\) \(=\dfrac{289}{9}\)
Sai rồi anh ơi 😢
c)S={-4}
d)S={49}
Sách nó viết thế chứ em ko biết nha

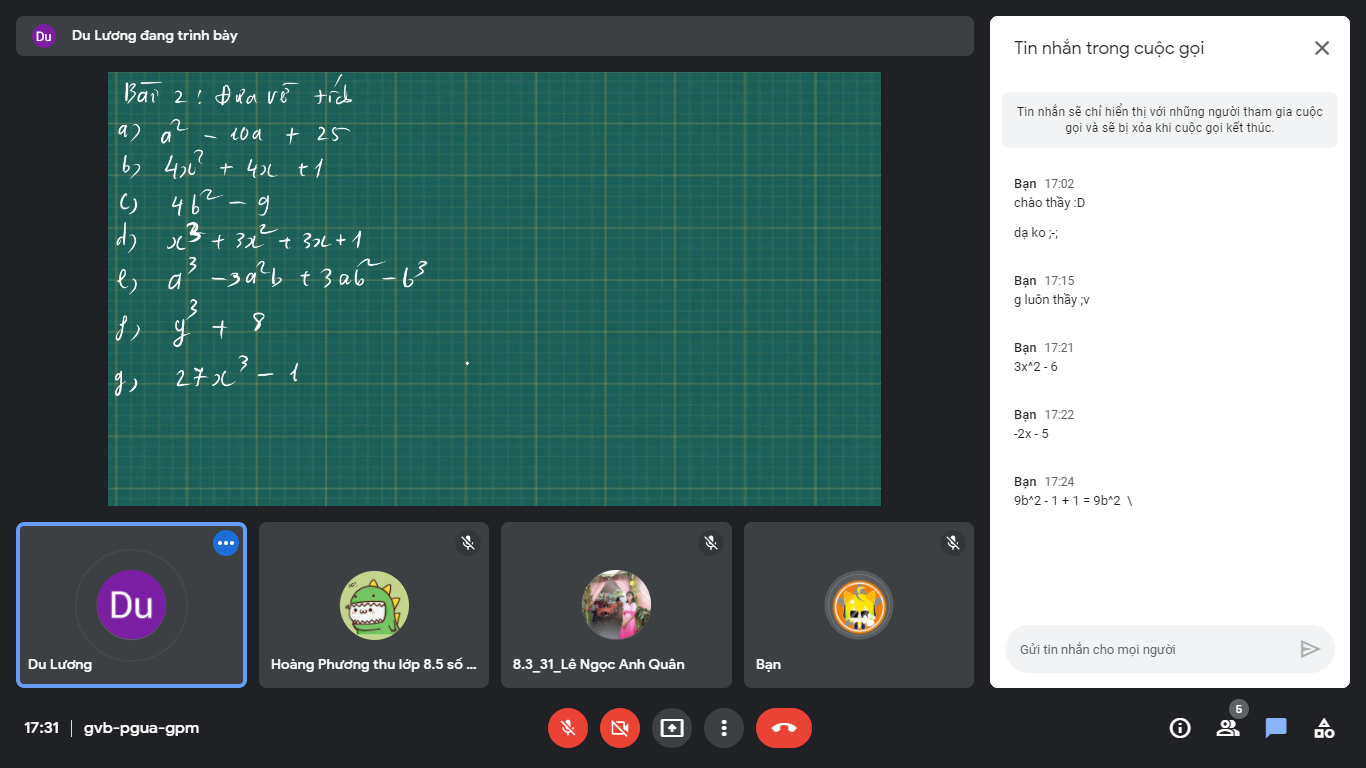
\(a,a^2-10a+25=\left(a-5\right)^2\\ b,4x^2+4x+1=\left(2x+1\right)^2\\ c,4x^2-9=\left(2x-3\right)\left(2x+3\right)\\ d,x^3+3x^2+3x+1=\left(x+1\right)^3\\ e,a^3-3a^2b+3ab^2-b^3=\left(a-b\right)^3\\ f,y^3+8=\left(y+2\right)\left(y^2-2y+4\right)\\ g,27x^3-1=\left(3x-1\right)\left(9x^2+3x+1\right)\)
\(a^2-10x+25=\left(a-5\right)^2\)
b/ \(4x^2+4x+1=\left(2x+1\right)^2\)
c/ \(4b^2-9=\left(2b-3\right)\left(2b+3\right)\)
d/ \(x^3+3x^2+3x+1=\left(x+1\right)^3\)
e/ \(a^3-3a^2b+3ab^2-b^3=\left(a-b\right)^3\)
f/ \(y^3+8=\left(y+2\right)\left(y^2-2y+4\right)\)
g/ \(27x^3-1=\left(3x-1\right)\left(9x^2+3x+1\right)\)

Bài 2:
\(\left\{{}\begin{matrix}Z-N=-1\\2Z-N=12\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-Z=-13\\Z-N=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}Z=13\\N=14\end{matrix}\right.\)

Bài 1:
a: \(=-10x^3+20x^4-5x\)
b: \(=\dfrac{1}{3}a^2b+7a^5-1\)
c: \(=a^3+8+25-a^3=33\)
d: \(=x^2-16+8-x^3=-x^3+x^2-8\)
e: \(=a^3+1+8-a^3=9\)
f: \(=\dfrac{7-2x+4x-8}{2x+3}=\dfrac{2x-1}{2x+3}\)
g: \(=\dfrac{3}{2\left(x+3\right)}-\dfrac{2}{x\left(x+3\right)}\)
\(=\dfrac{3x-4}{2x\left(x+3\right)}\)






\(x^2+5y^2+2x-4xy-10y+14\)
\(=\left(x^2+4y^2+1-4xy+2x-4y\right)+\left(y^2-6y+9\right)+4\)
\(=\left(x-2y+1\right)^2+\left(y-3\right)^2+4>0\) ; \(\forall x;y\) (đpcm)