Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Thay m=-5 vào (1), ta được:
\(x^2+2\left(-5+1\right)x-5-4=0\)
\(\Leftrightarrow x^2-8x-9=0\)
=>(x-9)(x+1)=0
=>x=9 hoặc x=-1
b: \(\text{Δ}=\left(2m+2\right)^2-4\left(m-4\right)=4m^2+8m+4-4m+16=4m^2+4m+20>0\)
Do đó: Phương trình luôn có hai nghiệm phân biệt
\(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}=-3\)
\(\Leftrightarrow x_1^2+x_2^2=-3x_1x_2\)
\(\Leftrightarrow\left(x_1+x_2\right)^2+x_1x_2=0\)
\(\Leftrightarrow\left(2m+2\right)^2+m-4=0\)
\(\Leftrightarrow4m^2+9m=0\)
=>m(4m+9)=0
=>m=0 hoặc m=-9/4

b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)

Δ=(m+2)^2-4*2m=(m-2)^2
Để PT có hai nghiệm pb thì m-2<>0
=>m<>2
\(\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{x_1x_2}{4}\)
=>\(\dfrac{x_1+x_2}{x_1x_2}=\dfrac{x_1x_2}{4}\)
=>\(\dfrac{m+2}{2m}=\dfrac{2m}{4}=\dfrac{m}{2}\)
=>2m^2=2m+4
=>m^2-m-2=0
=>m=2(loại) hoặc m=-1

\(x^2-2\left(m+4\right)x+m^2+8m-9=0\left(1\right)\)
Ta giải \(\Delta=[-2\left(m+4\right)]^2-4\left(m^2+8m-9\right)=100>0\forall m\)
suy ra pt có 2 nghiệm phân biệt \(x_1,x_2\forall m\).
Ta có: \(x_1=m-1\), \(x_2=m+1\) (thay \(\Delta\) vào công thức tìm nghiệm phân biệt).
Gọi \(A=\dfrac{x_1^2+x_2^2-48}{x_1^2+x_2^2}\).
\(\Rightarrow A=1-\dfrac{48}{x_1^2+x_2^2}=1-\dfrac{48}{\left(m-1\right)^2+\left(m+1\right)^2}=1-\dfrac{24}{m^2+1}\).
Để biểu thức A nguyên thì \(\dfrac{24}{m^2+1}\) nguyên, suy ra \(m^2+1\inƯ\left(24\right)\).
\(\Rightarrow m^2+1\in\left\{1;2;4;6;8;12;24\right\}\)
\(\Rightarrow m\in\left\{0;\pm1\right\}\) (vì m nhận giá trị nguyên)
Vậy \(m\in\left\{0;\pm1\right\}\) là giá trị cần tìm.
Mình chỉnh sửa lại một chút nhé.
\(A=1-\dfrac{24}{m^2+2}\)
\(\Rightarrow...\)\(\Rightarrow\)\(m^2+2\in\left\{1;2;3;4;6;8;12;24\right\}\)
\(\Rightarrow m\in\left\{0;\pm1;\pm2\right\}\)
Vậy...

a. Với m=6 thì phương trình (1) có dạng
x^2 - 5x +4= 0
<=> (x-1)(x-4)=0
<=> x=1 hoặc x=4
Vậy m=6 thì phương trình có nghiệm x=1 hoặc x=4
b. Xét \(\text{ Δ}=\left(-5\right)^2-4\cdot1\cdot\left(m-2\right)=33-4m\)
Để (1) có nghiệm phân biệt khi \(m< \dfrac{33}{4}\)
Theo Vi-et ta có: \(x_1x_2=m-2;x_1+x_2=5\)
Để 2 nghiệm phương trình (1) dương khi m>2
Ta có:
\(\dfrac{1}{\sqrt{x_1}}+\dfrac{1}{\sqrt{x_2}}=\dfrac{3}{2}\Leftrightarrow\dfrac{1}{x_1}+\dfrac{1}{x_2}+\dfrac{2}{\sqrt{x_1x_2}}=\dfrac{9}{4}\\ \Leftrightarrow\dfrac{x_1+x_2}{x_1x_2}+\dfrac{2}{\sqrt{x_1x_2}}=\dfrac{9}{4}\\ \Leftrightarrow\dfrac{5}{m-2}+\dfrac{2}{\sqrt{m-2}}=\dfrac{9}{4}\Leftrightarrow20+8\sqrt{m-2}=9\left(m-2\right)\\ \Leftrightarrow\left(\sqrt{m-2}-2\right)\left(9\sqrt{m-2}+10\right)=0\Leftrightarrow\sqrt{m-2}=2\Leftrightarrow m-2=4\Leftrightarrow m=6\left(t.m\right)\)

(a) Khi \(m=2,\left(1\right)\Leftrightarrow x^2-4x-5=0\left(2\right)\).
Phương trình (2) có \(a-b+c=1-\left(-4\right)+\left(-5\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{c}{a}=5\end{matrix}\right.\).
Vậy: Khi \(m=2,S=\left\{-1;5\right\}\).
(b) Điều kiện: \(x_1,x_2\ne0\Rightarrow m\in R\)
Phương trình có nghiệm khi:
\(\Delta'=\left(-m\right)^2-1\cdot\left(-m^2-1\right)\ge0\)
\(\Leftrightarrow2m^2+1\ge0\left(LĐ\right)\)
Suy ra, phương trình (1) có nghiệm với mọi \(m\).
Theo định lí Vi-ét: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2m\\x_1x_2=\dfrac{c}{a}=-m^2-1\end{matrix}\right.\)
Theo đề: \(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}=-\dfrac{5}{2}\)
\(\Leftrightarrow\dfrac{x_1^2+x_2^2}{x_1x_2}=-\dfrac{5}{2}\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-2x_1x_2}{x_1x_2}=-\dfrac{5}{2}\)
\(\Leftrightarrow2\left(x_1+x_2\right)^2+x_1x_2=0\)
\(\Leftrightarrow2\left(2m\right)^2+\left(-m^2-1\right)=0\)
\(\Leftrightarrow7m^2=1\Leftrightarrow m=\pm\dfrac{\sqrt{7}}{7}\) (thỏa mãn).
Vậy: \(m=\pm\dfrac{\sqrt{7}}{7}.\)

Xét \(\Delta=4\left(m-1\right)^2-4.\left(-3\right)=4\left(m-1\right)^2+12>0\forall m\)
=>Pt luôn có hai nghiệm pb
Theo viet:\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1.x_2=-3\ne0\forall m\end{matrix}\right.\)
Có \(\dfrac{x_1}{x_2^2}+\dfrac{x_2}{x_1^2}=m-1\)
\(\Leftrightarrow x_1^3+x_2^3=\left(m-1\right)x_1^2.x_2^2\)
\(\Leftrightarrow\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)=\left(m-1\right).\left(-3\right)^2\)
\(\Leftrightarrow8\left(m-1\right)^3-3\left(-3\right).2\left(m-1\right)=9\left(m-1\right)\)
\(\Leftrightarrow8\left(m-1\right)^3+9\left(m-1\right)=0\)
\(\Leftrightarrow\left(m-1\right)\left[8\left(m-1\right)^2+9\right]=0\)
\(\Leftrightarrow m=1\)(do \(8\left(m-1\right)^2+9>0\) với mọi m)
Vậy m=1
Vì \(ac< 0\) \(\Rightarrow\) Phương trình luôn có 2 nghiệm phân biệt
Theo Vi-ét, ta có: \(\left\{{}\begin{matrix}x_1+x_2=2m-2\\x_1x_2=-3\end{matrix}\right.\)
Mặt khác: \(\dfrac{x_1}{x_2^2}+\dfrac{x_2}{x_1^2}=m-1\) \(\Rightarrow\dfrac{\left(x_1+x_2\right)\left(x_1^2+x_2^2-x_1x_2\right)}{x_1^2x_2^2}=m-1\)
\(\Leftrightarrow\dfrac{\left(x_1+x_2\right)\left[\left(x_1+x_2\right)^2-3x_1x_2\right]}{x_1^2x_2^2}=m-1\)
\(\Rightarrow\dfrac{\left(2m-2\right)\left(4m^2-8m+13\right)}{9}=m-1\)
\(\Leftrightarrow...\)

Để pt có hai nghiệm pb \(\Leftrightarrow\Delta>0\)\(\Leftrightarrow4-4\left(m-1\right)>0\)\(\Leftrightarrow2>m\)
Theo viet có:\(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=m-1\end{matrix}\right.\)
Có \(x_1^2+x_2^2-3x_1x_2=2m^2+\left|m-3\right|\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-5x_1x_2=2m^2+\left|m-3\right|\)
\(\Leftrightarrow4-5\left(m-1\right)=2m^2+\left|m-3\right|\)
\(\Leftrightarrow2m^2+\left|m-3\right|-9+5m=0\) (1)
TH1: \(m\ge3\)
PT (1) \(\Leftrightarrow2m^2+m-3-9+5m=0\)
\(\Leftrightarrow2m^2+6m-12=0\)
Do \(m\ge3\Rightarrow\left\{{}\begin{matrix}6m-12\ge6>0\\2m^2>0\end{matrix}\right.\)
\(\Rightarrow2m^2+6m-12>0\)
=>Pt vô nghiệm
TH2: \(m< 3\)
PT (1)\(\Leftrightarrow2m^2-\left(m-3\right)-9+5m=0\)
\(\Leftrightarrow2m^2+4m-6=0\) \(\Leftrightarrow2m^2-2m+6m-6=0\)
\(\Leftrightarrow2m\left(m-1\right)+6\left(m-1\right)=0\)\(\Leftrightarrow\left(2m+6\right)\left(m-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=-3\\m=1\end{matrix}\right.\) (Thỏa)
Vậy...
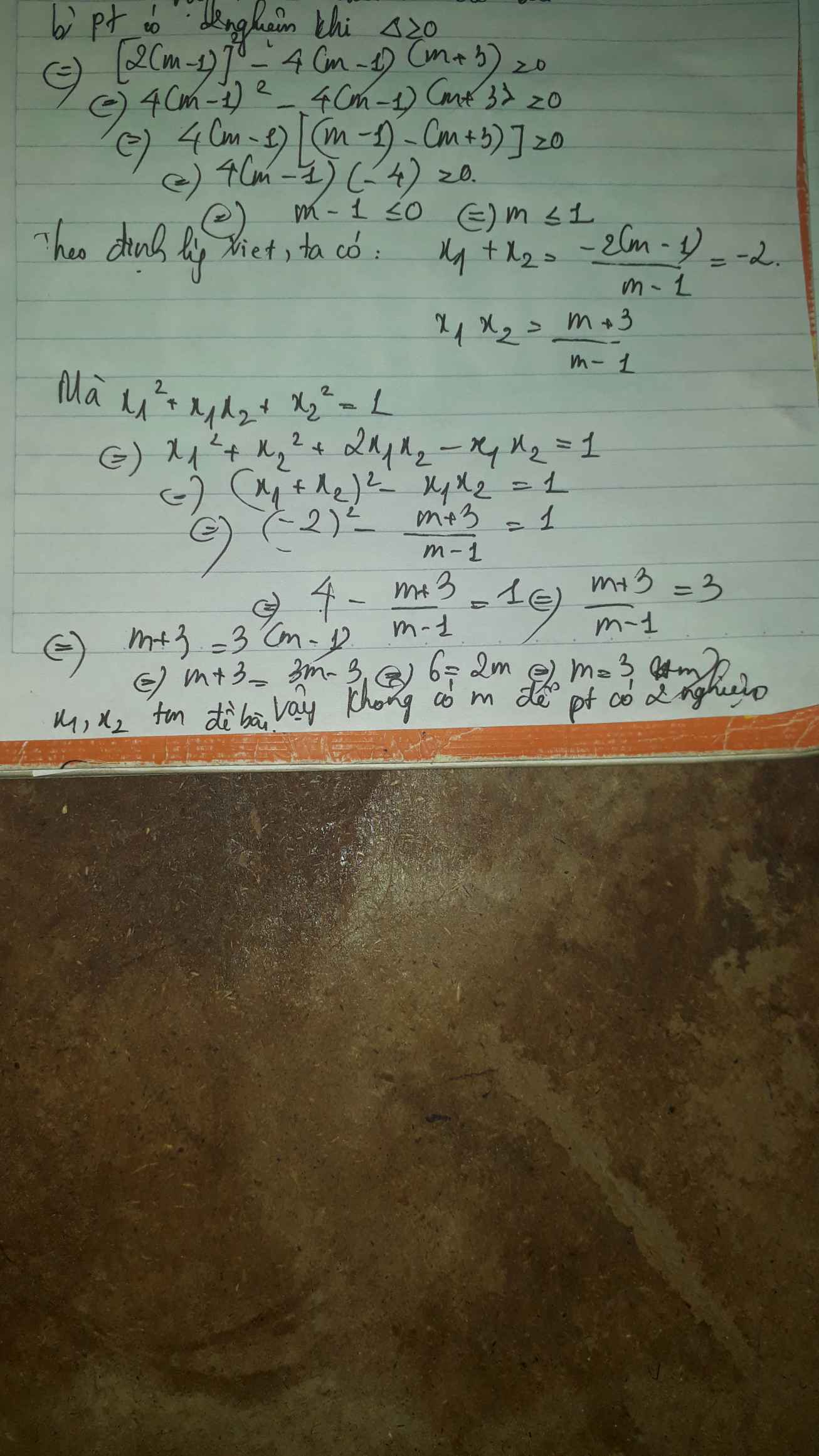
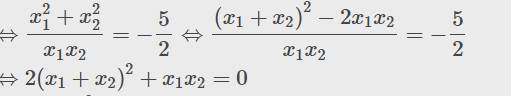
Đáp số: �=−3m=−3