Làm tính trừ phân thức x + 3 x 2 - 1

Khách

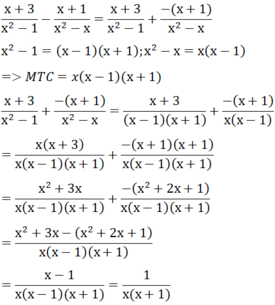
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn lớp

Chọn môn

Mua vip

Pham Trong Bach

11 tháng 7 2017

Làm tính trừ phân thức x + 3 x 2 - 1 - x + 1 x 2 - x

#Toán lớp 8

Cao Minh Tâm

11 tháng 7 2017

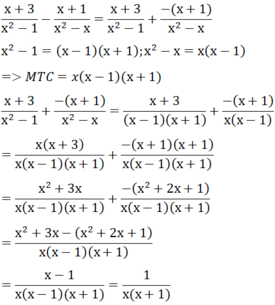
Để học tốt Toán 8 | Giải toán lớp 8

Đúng(0)

Các câu hỏi dưới đây có thể giống với câu hỏi trên

Pham Trong Bach

9 tháng 10 2019

Làm tính trừ phân thức: x + 1 x - 3 - 1 - x x + 3

#Toán lớp 8

Cao Minh Tâm

9 tháng 10 2019

Đúng(0)

Ko Tên

27 tháng 11 2018 - olm

Làm tính trừ các phân thức

a) 1/3x-2 - 1/3x+2 - -3+6/9x² -4

b) 1/x²-x+1 +1 - x² + 2/x³+1

#Toán lớp 8

Đặng Thị Vân Anh

27 tháng 11 2018

$\frac{1}{3x-2}-\frac{1}{3x+2}-\frac{-3+6}{9x^2-4}=\frac{1}{3x-2}-\frac{1}{3x+2}-\frac{3}{\left(3x-2\right)\left(3x+2\right)}=\frac{3x+2_{ }-3x+2-3}{\left(3x-2\right)\left(3x+2\right)}=\frac{1}{\left(3x-2\right)\left(3x+2\right)}$

Đúng(0)

Đặng Thị Vân Anh

27 tháng 11 2018

ý b bạn chỉ cần phân tích mẩu ra nó là 1 hằng đẳng thức rồi lấy mẫu chung là hằng đẩng thưcs đó rồi làm như thường

Đúng(0)

Pham Trong Bach

5 tháng 4 2017

Làm tính trừ các phân thức: 9 x + 5 6 ( x + 3 ) 2 - 5 x - 7 6 ( x + 3 ) 2

#Toán lớp 8

Cao Minh Tâm

5 tháng 4 2017

Đúng(0)

hoàng trần

10 tháng 12 2021

Cộng trừ phân thức
(1)/(x^(2)-x+1)+1-(x^(2)+2)/(x^(3)+1)

#Toán lớp 8

Kenny

10 tháng 12 2021

$\dfrac{1}{x^2-x+1}-\dfrac{x^2+2}{x^3+1}=\dfrac{1}{x^2-x+1}-\dfrac{x^2+2}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}-\dfrac{x^2+2}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{x+1-x^2-2}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{-x^2+x-1}{\left(x+1\right)\left(x^2-x+1\right)}$

Đúng(0)

hoàng trần

13 tháng 12 2021

cảm ơn :))

Đúng(1)

Đoàn Phương Linh

13 tháng 12 2021

giúp mik thực hiện phép trừ phân thức này với

a, $\dfrac{1}{x^2-x+1}+1-\dfrac{x^2+2}{x^3+1}$

#Toán lớp 8

Nguyễn Việt Lâm

Giáo viên

13 tháng 12 2021

$=\dfrac{x+1}{x^3+1}+\dfrac{x^3+1}{x^3+1}-\dfrac{x^2+2}{x^3+1}$

$=\dfrac{x+1+x^3+1-x^2-2}{x^3+1}$

$=\dfrac{x^3-x^2+x}{x^3+1}=\dfrac{x\left(x^2-x+1\right)}{\left(x+1\right)\left(x^2-x+1\right)}$

$=\dfrac{x}{x+1}$

Đúng(1)

nguyễn trần ngọc linh

12 tháng 11 2023

5)thực hiện phân thức cộng trừ saua)1/2a+2/3bb)x-1/x+1-x+1/x-1c)x+y/xy-y+z/yzd)2/x-3-12/x^2-9e)1/x-2+2/x^2-4x+4

#Toán lớp 8

Akai Haruma

Giáo viên

13 tháng 11 2023

Bạn nên viết đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo) để mọi người đọc hiểu đề của bạn hơn nhé.

Đúng(0)

Nguyễn Lê Phước Thịnh

13 tháng 11 2023

a: $\dfrac{1}{2a}+\dfrac{2}{3b}$(ĐKXĐ: a<>0 và b<>0)

$=\dfrac{1\cdot3b+2\cdot2a}{2a\cdot3b}$

$=\dfrac{3b+4a}{6ab}$

b: $\dfrac{x-1}{x+1}-\dfrac{x+1}{x-1}$(ĐKXĐ: $x\notin\left\{1;-1\right\}$)

$=\dfrac{\left(x-1\right)^2-\left(x+1\right)^2}{\left(x+1\right)\left(x-1\right)}$

$=\dfrac{x^2-2x+1-x^2-2x-1}{\left(x+1\right)\left(x-1\right)}=\dfrac{-4x}{x^2-1}$

c: $\dfrac{x+y}{xy-y}+\dfrac{z}{yz}$(ĐKXĐ: $\left\{{}\begin{matrix}x< >1\\y< >0\\z< >0\end{matrix}\right.$)

$=\dfrac{x+y}{y\left(x-1\right)}+\dfrac{1}{y}$

$=\dfrac{x+y+x-1}{y\left(x-1\right)}$

$=\dfrac{2x+y-1}{y\left(x-1\right)}$

d: ĐKXĐ: $x\notin\left\{3;-3\right\}$

$\dfrac{2}{x-3}-\dfrac{12}{x^2-9}$

$=\dfrac{2}{x-3}-\dfrac{12}{\left(x-3\right)\left(x+3\right)}$

$=\dfrac{2x+6-12}{\left(x-3\right)\left(x+3\right)}=\dfrac{2x-6}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{x+3}$

e: ĐKXĐ: x<>2

$\dfrac{1}{x-2}+\dfrac{2}{x^2-4x+4}$

$=\dfrac{1}{x-2}+\dfrac{2}{\left(x-2\right)^2}$

$=\dfrac{x-2+2}{\left(x-2\right)^2}=\dfrac{x}{\left(x-2\right)^2}$

Đúng(0)

Pham Trong Bach

1 tháng 2 2017

Làm tính trừ các phân thức: 4 x + 7 x 2 + 2 - x + 6 x 2 + 2

#Toán lớp 8

Cao Minh Tâm

1 tháng 2 2017

Đúng(0)

Nguyễn Dương Tuấn Kiệt

29 tháng 10 2015 - olm

Trừ phân thức

9x+5/2(x-1)(x+3)² - 5x-7/2(x-1)(x+3)²

#Toán lớp 8

Sách Giáo Khoa

21 tháng 4 2017

Áp dụng quy tắc đổi dấu để các phân thức có cùng mẫu thức rồi làm tính cộng phân thức :

a) $\dfrac{2x^2-x}{x-1}+\dfrac{x+1}{1-x}+\dfrac{2-x^2}{x-1}$

b) $\dfrac{4-x^2}{x-3}+\dfrac{2x-2x^2}{3-x}+\dfrac{5-4x}{x-3}$

#Toán lớp 8

Anh Triêt

21 tháng 4 2017

Giải bài 22 trang 46 Toán 8 Tập 1 | Giải bài tập Toán 8

Đúng(0)

ĐứcLĩnh TH

30 tháng 11 2021

Giải bài 22 trang 46 Toán 8 Tập 1 | Giải bài tập Toán 8

Đúng(0)

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP