K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
28 tháng 9 2021
a) \(=5x^3-x^2+2\)
b) \(=\dfrac{15}{6}xy-1-\dfrac{1}{2}y\)
28 tháng 9 2021
\(a,=5x^3-x^2+2\\ b,=\dfrac{5}{2}x^2\cdot\dfrac{3}{2}y-1-\dfrac{1}{2}x\cdot\dfrac{1}{2}y=\dfrac{15}{4}x^2y-\dfrac{1}{4}xy-1\)
CM
3 tháng 2 2018
5 x 4 - 3 x 3 + x 2 : 3 x 2 = 5 x 4 : 3 x 2 + - 3 x 3 : 3 x 2 + x 2 : 3 x 2 = 5 3 . x 2 - x + 1 3
3 tháng 6 2023
1: Sửa đề: 3x-5
\(=\dfrac{-x^2\left(3x-5\right)-3\left(3x-5\right)}{3x-5}=-x^2-3\)
2: \(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
=5x^2+14x^2+12x+8
3: \(=\dfrac{5x^3+10x^2+4x^2+8x+4x+8}{x+2}=5x^2+4x+4\)
4: \(=\dfrac{\left(x^2-1\right)\left(x^2+1\right)-2x\left(x^2-1\right)}{x^2-1}=x^2+1-2x\)
5: \(=\dfrac{x^2\left(5-3x\right)+3\left(5-3x\right)}{5-3x}=x^2+3\)
27 tháng 7 2021
c) Ta có: \(\dfrac{5x^4+9x^3-2x^2-4x-8}{x-1}\)
\(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
\(=\dfrac{5x^3\left(x-1\right)+14x^2\left(x-1\right)+12x\left(x-1\right)+8\left(x-1\right)}{x-1}\)
\(=5x^3+14x^2+12x+8\)
d) Ta có: \(\dfrac{5x^3+14x^2+12x+8}{x+2}\)
\(=\dfrac{5x^3+10x^2+4x^2+8x+4x+8}{x+2}\)
\(=\dfrac{5x^2\left(x+2\right)+4x\left(x+2\right)+4\left(x+2\right)}{x+2}\)
\(=5x^2+4x+4\)
27 tháng 7 2021
c) Ta có: \(\dfrac{5x^4+9x^3-2x^2-4x-8}{x-1}\)
\(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
\(=\dfrac{5x^3\left(x-1\right)+14x^2\left(x-1\right)+12x\left(x-1\right)+8\left(x-1\right)}{x-1}\)
\(=5x^3+14x^2+12x+8\)
MH
10 tháng 9 2021
x=4
=>x+1=5
A=(x+1)x^5 -(x+1)x^4+(x+1)x^3-(x+1)x^2+(x+1)x-1
=x^6+x^5-x^5-x^4+x^4+x^3-x^3-x^2+x^2-x+1
=x^6-x-1
=4^6-4-1
=4091
10 tháng 9 2021
\(a,A=5\cdot4^5-5\cdot4^4+5\cdot4^3-5\cdot4^2+5\cdot4+1\\ A=4^4\left(20-5\right)+4^2\left(20-5\right)+\left(20-5\right)\\ A=15\left(4^4+4^2+1\right)=15\cdot273=4095\)
\(b,x=7\Leftrightarrow x+1=8\\ \Leftrightarrow B=x^{2006}-\left(x+1\right)x^{2005}+\left(x+1\right)x^{2004}-...+\left(x+1\right)x^2-\left(x+1\right)x-5\\ B=x^{2006}-x^{2006}-x^{2005}+x^{2005}+x^{2004}-...+x^3+x^2-x^2-x-5\\ B=-x-5=-12\)
PT
1
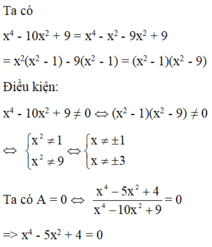
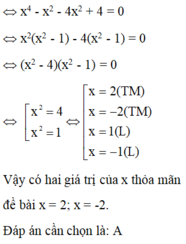
(25x5 – 5x4 + 10x2) : 5x2
= 25x5 : 5x2 + (-5x4) : 5x2 + 10x2 : 5x2
= (25 : 5).(x5 : x2) + (-5 : 5).(x4 : x2) + (10 : 5).(x2 : x2)
= 5.x5 – 2 + (-1).x4 – 2 + 2.1
= 5x3 – x2 + 2