Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: \(\sqrt{2017}-\sqrt{2016}=\dfrac{1}{\sqrt{2016}+\sqrt{2017}}\)
\(\sqrt{2016}-\sqrt{2015}=\dfrac{1}{\sqrt{2016}+\sqrt{2015}}\)
mà \(\sqrt{2016}+\sqrt{2017}< \sqrt{2016}+\sqrt{2015}\)
nên \(\sqrt{2017}-\sqrt{2016}>\sqrt{2016}-\sqrt{2015}\)

Ta có: \(S=\dfrac{1}{2+\sqrt{2}}+\dfrac{1}{3\sqrt{2}+2\sqrt{3}}+\dfrac{1}{4\sqrt{3}+3\sqrt{4}}+...+\dfrac{1}{100\sqrt{99}+99\sqrt{100}}\)
\(=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{99}}-\dfrac{1}{10}\)
\(=1-\dfrac{1}{10}=\dfrac{9}{10}\)

\(\Leftrightarrow2\sqrt{x}+2=\sqrt{x}+3\)
=>\(\sqrt{x}=1\)
hay x=1
\(\dfrac{\sqrt{x+1}}{\sqrt{x+3}}=\dfrac{1}{2}\)
\(\Leftrightarrow2\left(\sqrt{x+1}\right)=\sqrt{x}+3\)
\(\Leftrightarrow2\sqrt{x}+2=\sqrt{x}+3\)
\(\Leftrightarrow2\sqrt{x}+2-\sqrt{x}-3=0\)
\(\Leftrightarrow\sqrt{x}-1=0\Leftrightarrow\sqrt{x}=1\Leftrightarrow x=1\)


Bài 1:
\(D=\dfrac{1}{2}\sqrt{48}-\dfrac{\sqrt{33}}{\sqrt{11}}+5\sqrt{1\dfrac{1}{3}}=\dfrac{1}{2}.4\sqrt{3}-\sqrt{3}+5.\dfrac{2\sqrt{3}}{3}=2\sqrt{3}-\sqrt{3}+\dfrac{10\sqrt{3}}{3}=\dfrac{3\sqrt{3}+10\sqrt{3}}{3}=\dfrac{13\sqrt{3}}{3}\)
\(E=\sqrt{\dfrac{3-\sqrt{5}}{3+\sqrt{5}}}-\sqrt{\dfrac{3+\sqrt{5}}{3-\sqrt{5}}}=\sqrt{\dfrac{\left(3-\sqrt{5}\right)^2}{9-5}}-\sqrt{\dfrac{\left(3+\sqrt{5}\right)^2}{9-5}}=\dfrac{3-\sqrt{5}}{2}-\dfrac{3+\sqrt{5}}{2}=-\sqrt{5}\)
\(F=\sqrt{3+\sqrt{5}}+\sqrt{7-3\sqrt{5}}-\sqrt{2}=\sqrt{\left(\sqrt{\dfrac{5}{2}}+\sqrt{\dfrac{1}{2}}\right)^2}+\sqrt{\left(\dfrac{3}{\sqrt{2}}-\sqrt{\dfrac{5}{2}}\right)^2}-\sqrt{2}=\sqrt{\dfrac{5}{2}}+\sqrt{\dfrac{1}{2}}+\dfrac{3}{\sqrt{2}}-\sqrt{\dfrac{5}{2}}-\sqrt{2}=2\sqrt{2}-\sqrt{2}=\sqrt{2}\)
Bài 2:
Ta có: G-1
\(=\dfrac{\sqrt{x}-x+\sqrt{x}-1}{x-\sqrt{x}+1}\)
\(=\dfrac{-\left(x-2\sqrt{x}+1\right)}{x-\sqrt{x}+1}\)
\(=\dfrac{-\left(\sqrt{x}-1\right)^2}{x-\sqrt{x}+1}\le0\forall x\) thỏa mãn ĐKXĐ
hay \(G\le1\)

Với n\(\in N\)* có: \(\dfrac{1}{\left(n+1\right)\sqrt{n}+n\sqrt{n+1}}=\dfrac{1}{\sqrt{n\left(n+1\right)}\left(\sqrt{n+1}+\sqrt{n}\right)}\)\(=\dfrac{\sqrt{n+1}-\sqrt{n}}{\sqrt{n\left(n+1\right)}\left(n+1-n\right)}=\dfrac{\sqrt{n+1}-\sqrt{n}}{\sqrt{n\left(n+1\right)}}\)\(=\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\)
\(\Rightarrow\)\(\dfrac{1}{\left(n+1\right)\sqrt{n}+n\sqrt{n+1}}=\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\) (*)
a) Áp dụng (*) vào T
\(\Rightarrow T=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{99}}-\dfrac{1}{\sqrt{100}}\)\(=1-\dfrac{1}{10}=\dfrac{9}{10}\)
b) Có \(VT=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\)\(=1-\dfrac{1}{\sqrt{n+1}}=\dfrac{4}{5}\)
\(\Leftrightarrow\sqrt{n+1}=5\Leftrightarrow n=24\) (tm)
Vậy n=24.
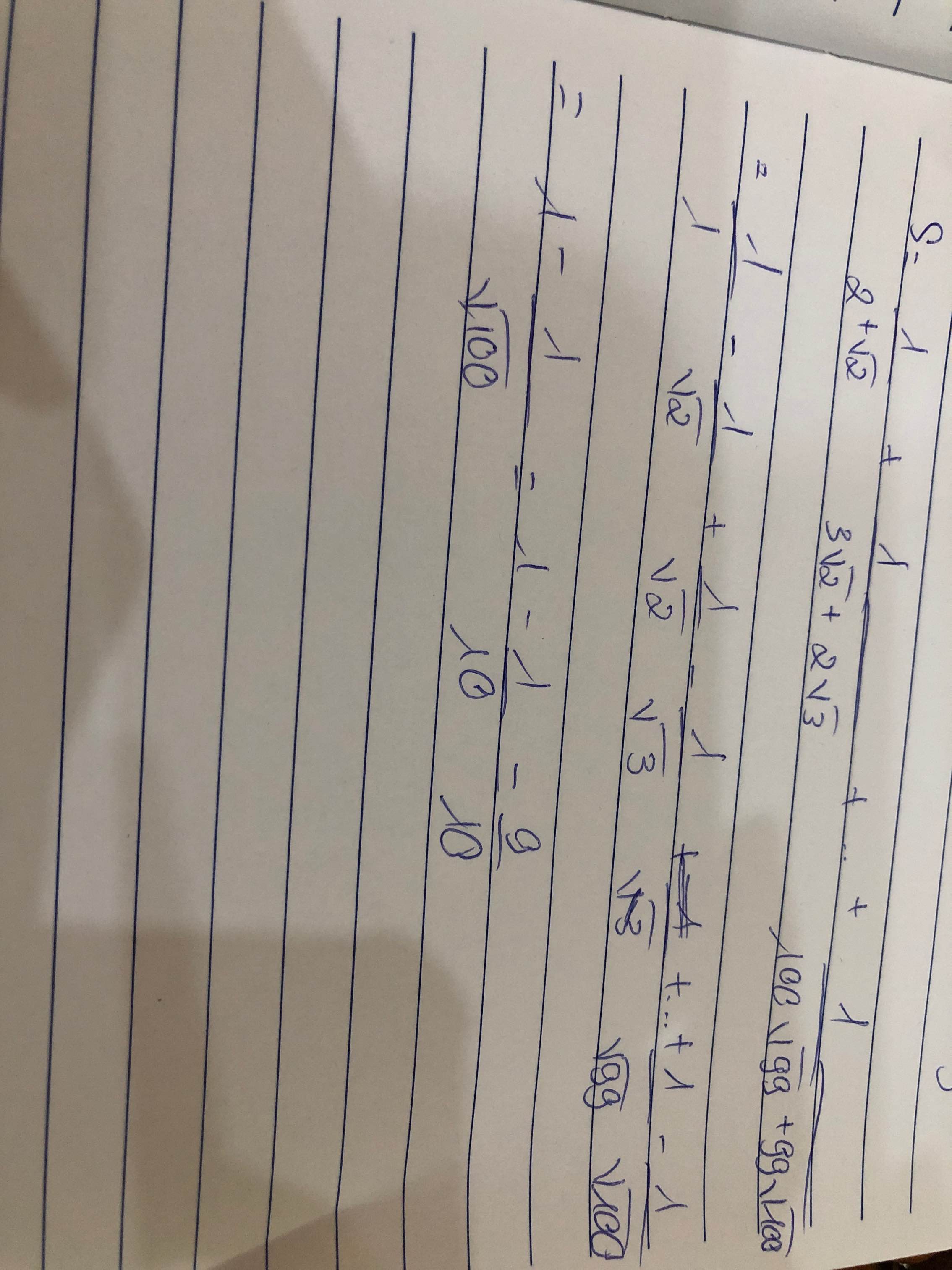
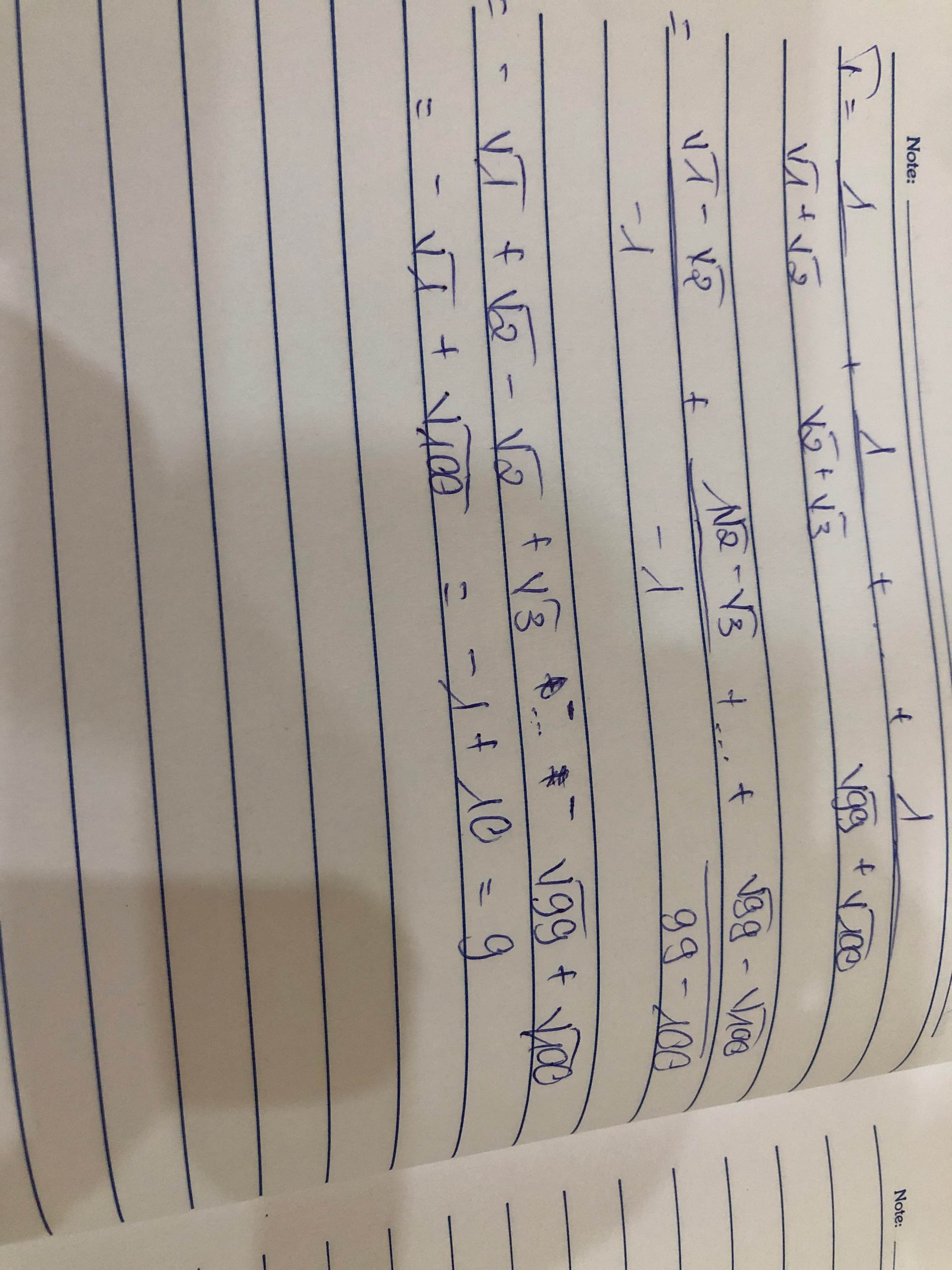


Lời giải:
$A=\frac{\sqrt{2}-1}{(1+\sqrt{2})(\sqrt{2}-1)}+\frac{\sqrt{3}-\sqrt{2}}{(\sqrt{2}+\sqrt{3})(\sqrt{3}-\sqrt{2})}+....+\frac{\sqrt{100}-\sqrt{99}}{(\sqrt{99}+\sqrt{100})(\sqrt{100}-\sqrt{99})}$
$=\frac{\sqrt{2}-1}{1}+\frac{\sqrt{3}-\sqrt{2}}{1}+....+\frac{\sqrt{100}-\sqrt{99}}{1}$
$=\sqrt{2}-1+\sqrt{3}-\sqrt{2}+\sqrt{4}-\sqrt{3}+....+\sqrt{100}-\sqrt{99}$
$=\sqrt{100}-1=10-1=9$