K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
6 tháng 6 2017
Chọn A
f ( x ) = 1 x 2 + x - 2 = 1 3 1 x - 1 - 1 x + 2
Nên ∫ f ( x ) d x = 1 3 ln x - 1 - ln x + 2 + C = F ( x ) = 1 3 ln x - 1 x + 2 + C
.
.
.
.
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 3 2022
Từ giả thiết: \(\int f\left(x\right).e^{2x}dx=x.e^x+C\)
Đạo hàm 2 vế:
\(\Rightarrow f\left(x\right).e^{2x}=e^x+x.e^x\)
\(\Rightarrow f\left(x\right)=\dfrac{e^x+x.e^x}{e^{2x}}=\dfrac{x+1}{e^x}\)
Xét \(I=\int f'\left(x\right)e^{2x}dx\)
Đặt \(\left\{{}\begin{matrix}u=e^{2x}\\dv=f'\left(x\right)\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=2.e^{2x}dx\\v=f\left(x\right)\end{matrix}\right.\)
\(\Rightarrow I=f\left(x\right).e^{2x}-2\int f\left(x\right).e^{2x}dx=\left(\dfrac{x+1}{e^x}\right)e^{2x}-2.x.e^x+C\)
\(=\left(1-x\right)e^x+C\)
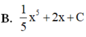
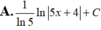
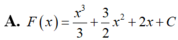
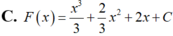
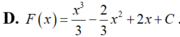
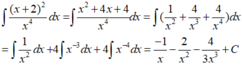
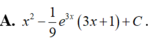
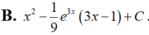
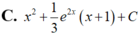
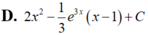
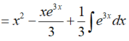
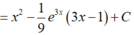
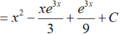
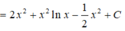
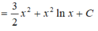
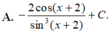
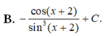
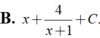
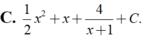
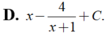
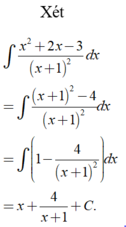
Đáp án A