K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
25 tháng 7 2021
Bài 18
a, Với \(a>0;a\ne1;4\)
\(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
\(=\left(\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}\right):\left(\dfrac{a-1-a+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}\right)\)
\(=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{3}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\)
b, Thay a = 9 => căn a = 3
\(A=\dfrac{3-2}{3.3}=\dfrac{1}{9}\)
c, Ta có : \(A.B=\dfrac{\sqrt{a}-2}{3\sqrt{a}}.\dfrac{3\sqrt{a}}{\sqrt{a}+1}=\dfrac{\sqrt{a}-2}{\sqrt{a}+1}< 0\)
Vì \(\sqrt{a}+1>\sqrt{a}-2\)
\(\left\{{}\begin{matrix}\sqrt{a}+1>0\\\sqrt{a}-2< 0\end{matrix}\right.\Leftrightarrow a< 4\)
Kết hợp với đk vậy \(0< a< 4;a\ne1\)
25 tháng 7 2021
Bài 18:
1) Ta có: \(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
\(=\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{a-1-a+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}\)
\(=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-1\right)\left(\sqrt{a}-2\right)}{3}\)
\(=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\)
2) Thay a=9 vào B, ta được:
\(B=\dfrac{3\cdot3}{3+1}=\dfrac{9}{4}\)
25 tháng 7 2021
a, \(A=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}\right):\dfrac{\sqrt{x}+1}{\left(\sqrt{x}-1\right)^2}\)ĐK : \(x>0;x\ne1\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)
b, \(A=\dfrac{1}{3}\Rightarrow\dfrac{\sqrt{x}-1}{\sqrt{x}}=\dfrac{1}{3}\Rightarrow3\sqrt{x}-3=\sqrt{x}\Leftrightarrow2\sqrt{x}=3\)
\(\Leftrightarrow\sqrt{x}=\dfrac{3}{2}\Leftrightarrow x=\dfrac{9}{4}\)
c, \(P=\dfrac{\sqrt{x}-1}{\sqrt{x}}-9\sqrt{x}=\dfrac{\sqrt{x}-1-9x}{\sqrt{x}}\)
\(=1-\dfrac{1}{\sqrt{x}}-9\sqrt{x}\)Đặt \(\sqrt{x}=t^2\left(t>0\right)\)
\(1-t-9t^2=-\left(9t^2-t-1\right)=-\left(9t^2-2.3.\dfrac{1}{6}.t+\dfrac{1}{36}-\dfrac{37}{36}\right)\)
\(=-\left(3t-\dfrac{1}{6}\right)+\dfrac{37}{36}\le\dfrac{37}{36}\)
Dấu ''='' xảy ra khi t = 1/18 => t^2 = 1/324 => \(\sqrt{x}=\dfrac{1}{324}\Rightarrow x=\dfrac{1}{104876}\)
Vậy GTLN P là 37/36 khi x = 1/104876
16 tháng 8 2021
\(\left\{{}\begin{matrix}\dfrac{9}{\sqrt{2x-1}}+\dfrac{3}{y+1}=2\\\dfrac{4}{\sqrt{2x-1}}-\dfrac{1}{y+1}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{9}{\sqrt{2x-1}}+\dfrac{3}{y+1}=2\left(1\right)\\\dfrac{12}{\sqrt{2x-1}}-\dfrac{3}{y+1}=3\left(2\right)\end{matrix}\right.\)
Lấy \(\left(2\right)+\left(1\right)\) ta được:
\(\dfrac{21}{\sqrt{2x-1}}=5\\ \Leftrightarrow5\sqrt{2x-1}=21\\ \Leftrightarrow25\left(2x-1\right)=441\\ \Leftrightarrow50x-25=441\\ \Leftrightarrow50x=466\Leftrightarrow x=\dfrac{233}{25}\)
Thay x vào (1)
\(\dfrac{9}{\sqrt{2\cdot\dfrac{233}{25}-1}}+\dfrac{3}{y+1}=2\\ \Leftrightarrow\dfrac{9}{\sqrt{\dfrac{441}{25}}}+\dfrac{3}{y+1}=2\\ \Leftrightarrow\dfrac{9}{\dfrac{21}{5}}+\dfrac{3}{y+1}=2\\ \Leftrightarrow\dfrac{15}{7}+\dfrac{3}{y+1}=2\\ \Leftrightarrow15\left(y+1\right)+21=14\left(y+1\right)\\ \Leftrightarrow15y+15+21=14y+14\\ \Leftrightarrow y=-22\)
Vậy pt có tập nghiệm \(\left(x;y\right)=\left(\dfrac{233}{25};-22\right)\)
16 tháng 8 2021
\(\left\{{}\begin{matrix}\dfrac{9}{\sqrt{2x-1}}+\dfrac{3}{y+1}=2\\\dfrac{4}{\sqrt{2x-1}}-\dfrac{1}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{36}{\sqrt{2x-1}}+\dfrac{12}{y+1}=8\\\dfrac{36}{\sqrt{2x-1}}-\dfrac{9}{y+1}=9\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{21}{y+1}=-1\\\dfrac{4}{\sqrt{2x-1}}-\dfrac{1}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y+1=-21\\\dfrac{4}{\sqrt{2x-1}}=\dfrac{20}{21}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-22\\2x-1=\dfrac{441}{25}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{233}{25}\\y=-22\end{matrix}\right.\)
22 tháng 7 2021
a) P rút gọn lại là = x(x-1)
b) Để P = 2 => \(x^2\)- x -2 = 0
=> x = 2 hay x = -1
c) Để P<12 => \(x^2\) - x -12< 0
=> (x-4)(x+3) <0
=> x-4 <0<x+3
=> x<4 hay x >-3
Vậy, -3<x<4 thì P<12
d) GTNN của P = \(x^2\)- x
= \(x^2\)- x +1/4 -1/4
= (x-1/2)\(^2\)-1/4 >= -1/4
Vậy, GTNN của x là -1/4 khi và chỉ khi x = 1/2
Nhớ like giúp mik nha bạn. Thx bạn nhìu:33
22 tháng 7 2021
a) Ta có: \(P=\left(\dfrac{x\sqrt{x}+x-2}{x-1}-\dfrac{1}{\sqrt{x}+1}\right):\dfrac{1}{x\sqrt{x}-x}\)
\(=\dfrac{x\sqrt{x}+x-2-\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\cdot\dfrac{x\left(\sqrt{x}-1\right)}{1}\)
\(=\dfrac{x\sqrt{x}+x-\sqrt{x}-1}{\sqrt{x}+1}\cdot x\)
\(=\dfrac{x\left(\sqrt{x}+1\right)-\left(\sqrt{x}+1\right)}{\sqrt{x}+1}\cdot x\)
\(=x^2-x\)
TH
1
16 tháng 6 2023
2:
1+cot^2a=1/sin^2a
=>1/sin^2a=1681/81
=>sin^2a=81/1681
=>sin a=9/41
=>cosa=40/41
tan a=1:40/9=9/40
CH
0
AH
0
HD
1
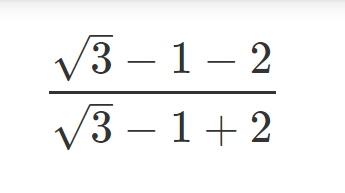
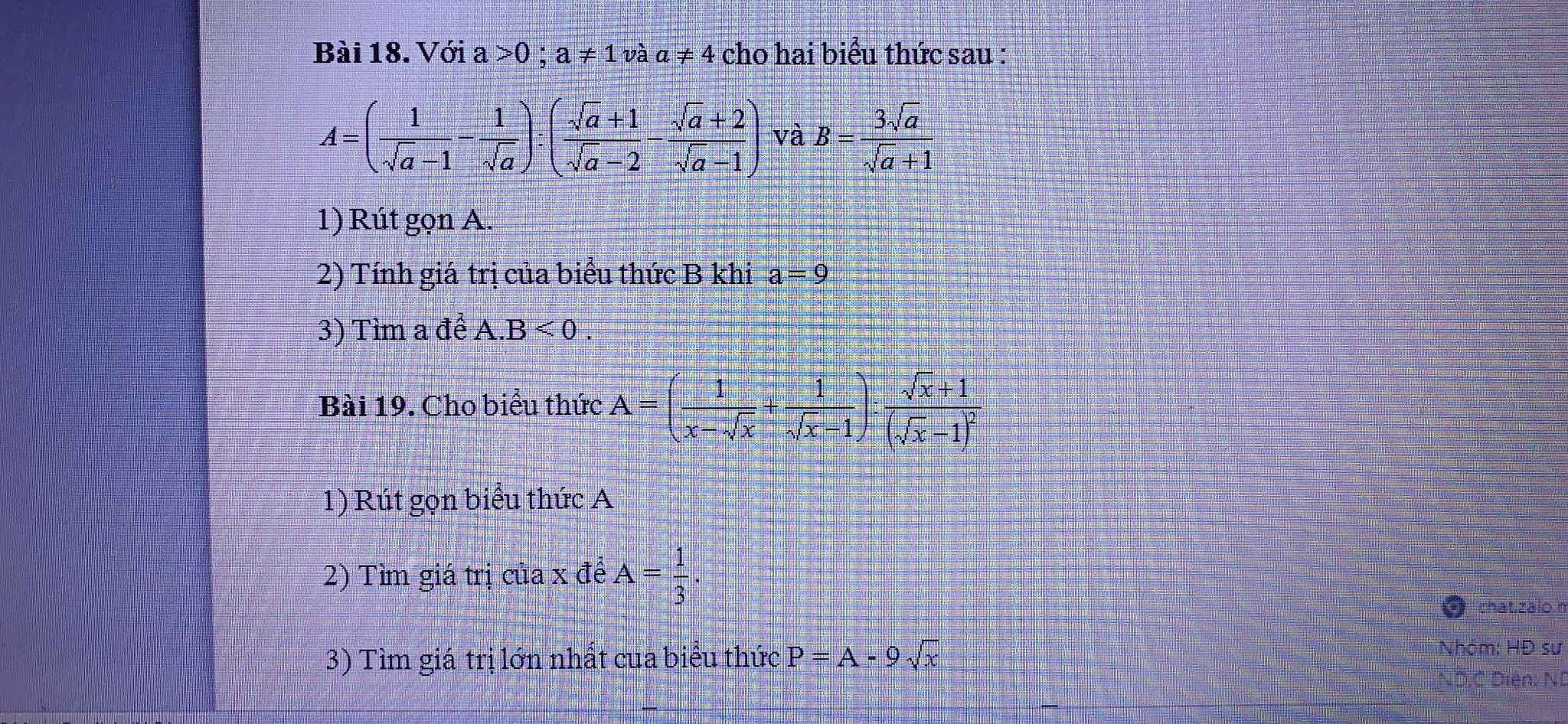
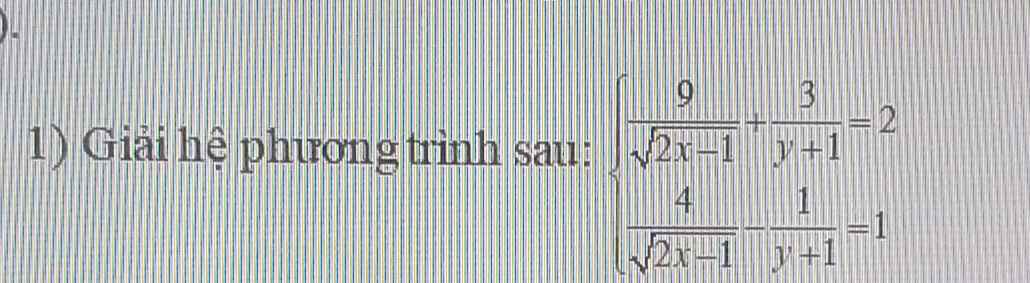
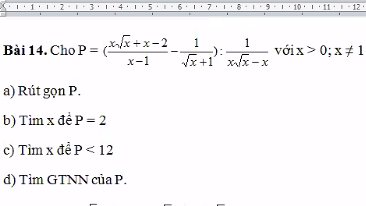
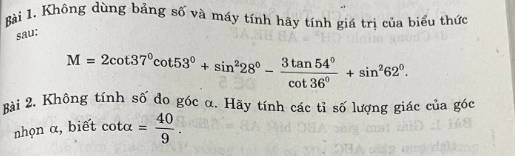
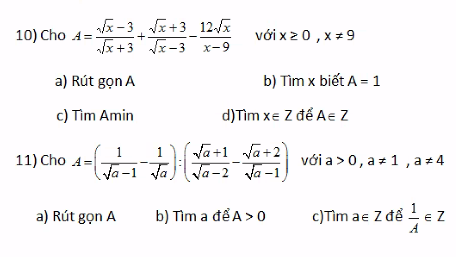
\(\dfrac{\sqrt{3}-3}{\sqrt{3}+1}=\dfrac{\left(\sqrt{3}-3\right)\left(\sqrt{3}-1\right)}{2}=\dfrac{3-\sqrt{3}-3\sqrt{3}+3}{2}=\dfrac{6-4\sqrt{3}}{2}=3-2\sqrt{3}\)