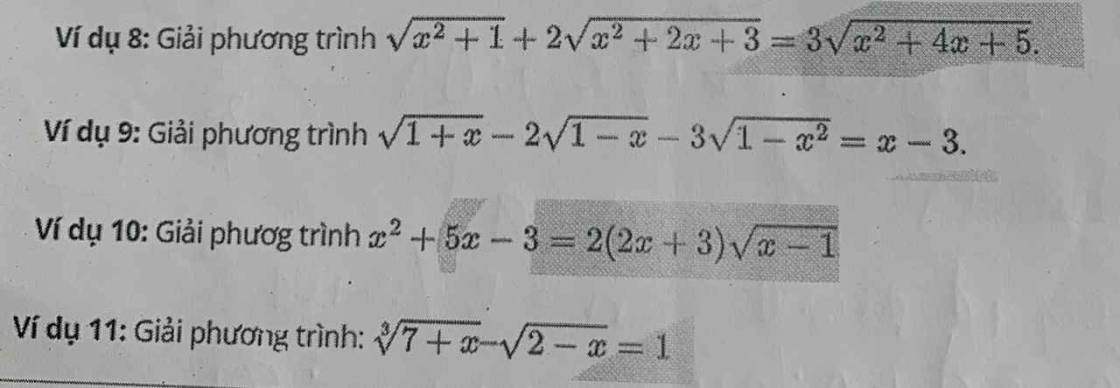K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
HI
0
TL
1
11 tháng 7 2023
a: f(x)=0
=>(x-3)(x+3)=0
=>x=3 hoặc x=-3
b: f(x)=0
=>(-2x+4)(2x^2+1)=0
=>4-2x=0
=>x=2
22 tháng 2 2017
a)\(\frac{a+b}{2}\ge\sqrt{ab}\Rightarrow a+b\ge2\sqrt{ab}\)
\(\Rightarrow a^2+2ab+b^2\ge4ab\)
\(\Rightarrow a^2-2ab+b^2\ge0\Rightarrow\left(a-b\right)^2\ge0\)
Dấu "=" xảy ra khi \(a=b\)
b)Áp dụng BĐT AM-GM ta có:
\(\left\{\begin{matrix}\frac{bc}{a}+\frac{ca}{b}\ge2\sqrt{\frac{bc}{a}\cdot\frac{ca}{b}}=2c\\\frac{bc}{a}+\frac{ab}{c}\ge2\sqrt{\frac{bc}{a}\cdot\frac{ab}{c}}=2b\\\frac{ca}{b}+\frac{ab}{c}\ge2\sqrt{\frac{ca}{b}\cdot\frac{ab}{c}}=2a\end{matrix}\right.\)
Cộng từng vế của 3 BĐT trên rồi thu gọn ta được điều cần chứng minh
Dấu "=" xảy ra khi \(a=b=c\)
c)Áp dụng BĐT AM-GM ta có:
\(\frac{3a+5b}{2}\ge\sqrt{3a\cdot5b}\Leftrightarrow\left(3a+5b\right)^2\ge4\cdot15P\)
\(\Leftrightarrow12^2\ge60P\Leftrightarrow P\le\frac{12}{5}\)
Dấu "=" xảy ra khi \(\left\{\begin{matrix}a=2\\b=\frac{6}{5}\end{matrix}\right.\)
HN
0
HB
6
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 1 2024
8.
Đặt \(\left\{{}\begin{matrix}\sqrt{x^2+2x+3}=a>0\\\sqrt{x^2+4x+5}=b>0\end{matrix}\right.\) \(\Rightarrow2a^2-b^2=x^2+1\)
Pt trở thành:
\(\sqrt{2a^2-b^2}+2a=3b\)
\(\Leftrightarrow\sqrt{2a^2-b^2}=3b-2a\)
\(\Rightarrow2a^2-b^2=4a^2-12ab+9b^2\)
\(\Leftrightarrow2a^2-12ab+10b^2=0\Rightarrow\left[{}\begin{matrix}a=b\\a=5b\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\sqrt{x^2+2x+3}=\sqrt{x^2+4x+5}\\\sqrt{x^2+2x+3}=5\sqrt{x^2+4x+5}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2+2x+3=x^2+4x+5\\x^2+2x+3=25\left(x^2+4x+5\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\24x^2+98x+122=0\left(vn\right)\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 1 2024
9.
ĐKXĐ: \(-1\le x\le1\)
Đặt \(\left\{{}\begin{matrix}\sqrt{1+x}=a\ge0\\\sqrt{1-x}=b\ge0\end{matrix}\right.\) \(\Rightarrow a^2+2b^2=3-x=-\left(x-3\right)\)
Pt trở thành:
\(a-2b-3ab=-\left(a^2+2b^2\right)\)
\(\Leftrightarrow a-2b+a^2-3ab+2b^2=0\)
\(\Leftrightarrow a-2b+\left(a-b\right)\left(a-2b\right)=0\)
\(\Leftrightarrow\left(a-2b\right)\left(a-b+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}a=2b\\a+1=b\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{1+x}=2\sqrt{1-x}\\\sqrt{1+x}+1=\sqrt{1-x}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}1+x=4\left(1-x\right)\\x+2+2\sqrt{1+x}=1-x\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=3\Rightarrow x=\dfrac{3}{5}\\-1-2x=2\sqrt{1+x}\left(1\right)\end{matrix}\right.\)
Xét (1) \(\Leftrightarrow\left\{{}\begin{matrix}-1-2x\ge0\\\left(-1-2x\right)^2=4\left(1+x\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\le-\dfrac{1}{2}\\x^2=\dfrac{3}{4}\end{matrix}\right.\) \(\Rightarrow x=-\dfrac{\sqrt{3}}{2}\)
Vậy \(x=\left\{\dfrac{3}{5};-\dfrac{\sqrt{3}}{2}\right\}\)
AN
30 tháng 10 2017
Hoành độ đỉnh: \(\dfrac{-b}{2a}=-\dfrac{-2}{2}=1\)
a > 0 nên đồ thị hướng lên
Vậy HS đồng biến trong khoảng (1;+\(\infty\)) -> Chọn A
CD
0